Let $p_n$ be the $n$-th prime.

For each integer $k \ge 0$, do there exist an infinite number of $k+3$ consecutive primes $(p_n, p_{n+1}, \ldots, p_{n+2+k})$ so that

• (1) The gap between the 1st and 2nd, and between the 2nd and last, are equal: $p_{n+1}-p_n = p_{n+2+k}-p_{n+1}$.

• (2) There are $k$ primes between the 2nd and last, i.e., between $p_{n+1}$ and $p_{n+2+k}$.

For $k=0$, the answer is Yes by the recent breakthroughs on prime gaps. Here are some examples:
     
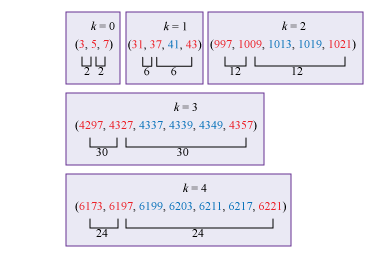
One could whimsically imagine "skipping" a flat stone on the primes, where the first bounce covers the gap between the 1st and 2nd primes, followed by $k+1$ smaller bounces that together cover the same gap before sinking on the last prime.