This is inspired by the self-similarity of the celebrated Golay-Rudin-Shapiro sequence, more exactly, of its alternating partial sums. (This latter one is oeis 020990). The pictures show the 550 first terms, then the 9000 first terms.

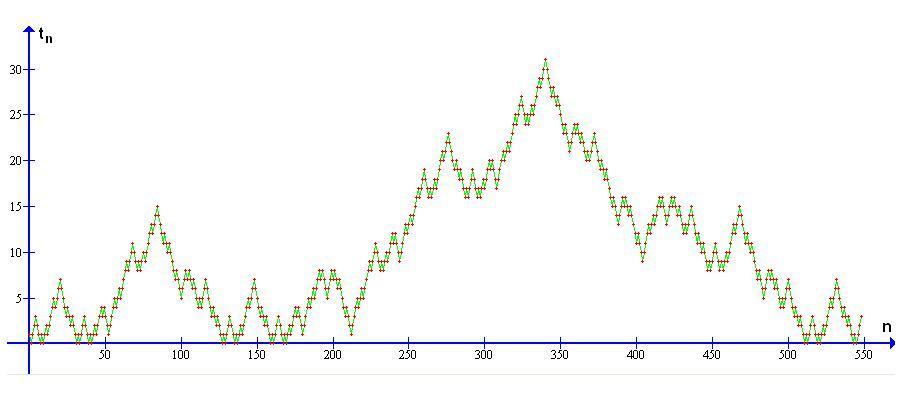
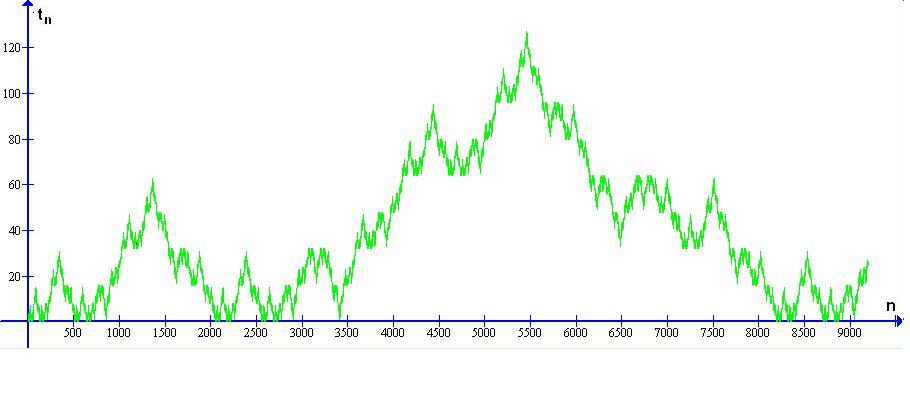
It makes sense to define a certain fractal $F$ as the "limit" of the graph $\Gamma=\{(n,a_n)\}_{n\ge0}$.
More precisely:
Fix a rectangle $R\subset\mathbb R^2$, e.g. the unit square. Take the part $\Gamma_k$ of $\Gamma$ between $n=2^{2k-1}$ and $n=2^{2k+1}-1$ and rescale it to a graph $\Gamma^0_k$ that fits $R$ best.
Then because of the geometrical (almost-) similarity of the $\Gamma^0_k$, the limit $F:=\lim\limits_{k\to\infty}\Gamma^0_k\subset\mathbb R^2$ is well-defined. Note that its Hausdorff dimension is $d=3/2$.

Other examples:

• the sequence oeis 004074 that defines likewise the Blancmange_curve, dimension $d=1$
• sequences linked to the Gray code, like 003188 or 006068, both with $d=1$
• Stern's diatomic series yields a fractal with dimension $d=\frac{\ln 3}{\ln 2}$
• it makes sense to relate (if not to identify) the Cantor set with the sequence $1,0,1,0,0,0,1,0,1,... $ where $a_n=1$ iff the ternary representation of $n$ has only 0's and 2's (equivalently, the cellular automaton where at each step $1 \mapsto 101$ and $0 \mapsto000$), and to say this sequence has dimension $\frac{\ln2}{\ln3}$. Likewise for the "fat Cantor set" iterating 11100111 (dimension $\frac{\ln5}{\ln8}$) and all other sorts of Cantor dust.
• the devil's staircase, obtained by "integrating" the Cantor set, corresponds to this sequence, and a "mirrored" version of it can be found here.
• Other sequences of Toothpick and Cellular Automata type

Note that it is not at all straightforward or even possible to define a fractal for every self-similar integer sequence $a=(a_n)_{n\ge0}$ (self-similar meaning as usual that there is a $k\ge2$ and $\lambda$ such that $a_n=\lambda a_{kn}$). On the other hand, there are also sequences with a fractal-like appearance without being self-similar in the above sense.

Question:

• Has the idea of the "fractal dimension" of certain sequences been treated before?