3
$\begingroup$

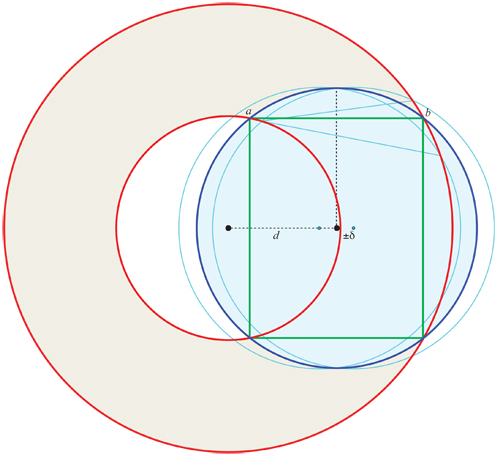
Given two concentric circles $[C_1,C_2 ]$ with radii $(R_1 < R_2) $ creating an annulus; where should a third circle ($C_3$, radius $R_3$) be located such that the area of intersection between the annulus and $C_3$ is maximum?

When I was trying to solve the problem I assumed WLOG, due to symmetry, that the center of $C_3$ is placed at some point along the x-axis $(d,0)$ and found $d$ to satisfy

$2(R_3^2 + d^2) = R_1^2 + R_2^2$

(If $d$ is not real, then let $d=0$ and all three circles are concentric)

  1. Is that correct?
  2. If so, is there a geometrical reason for that criteria?
Improve this question
$\endgroup$
2
  • $\begingroup$ It looks plausible. [It works for a few extremal cases at least.] How did you arrive at that? $\endgroup$
    – Pat Devlin
    Commented Apr 10, 2012 at 0:36
  • $\begingroup$ This site is for questions of research interest. I'm not convinced there's any research interest here, in which case math.stackexchange.com may be a better place to post. And I don't see where this is algebraic geometry. $\endgroup$
    – Gerry Myerson
    Commented Apr 10, 2012 at 11:07

2 Answers 2

Reset to default
3
$\begingroup$

Your condition on $d$ ensures that the intersections $a$ and $b$ between circles $C_3$ and $C_1$ and $C_2$ respectively, are at the same $y$-coordinate. Then the different slopes of $C_1$ and $C_2$ at these points ensure that the area is a local maximum.
    Annulus & Circle

Improve this answer
$\endgroup$
2
$\begingroup$

There is a formula for the area of the intersection of two circles of given radii in terms of the distance between the centers. The formula can be found here: http://mathworld.wolfram.com/Circle-CircleIntersection.html

If the radius of $C_3$ is smaller than $R_2-R_1$ or greater than $R_2$ then the answer is obvious. In the other cases you can also calculate the explicit area formula of the intersection. If we denote $\mathcal{A}(C_i,C_j)$ the area of the intersection of the circles $C_i,C_j$. Then the area of the intersection of $C_3$ with the annulus is $\mathcal{A}(C_2,C_3)-\mathcal{A}(C_1,C_3)$, and using the formulas presented in the link you can write the exact formula in terms of $d$, and then optimize with respect to $d$ the formula you get.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged .