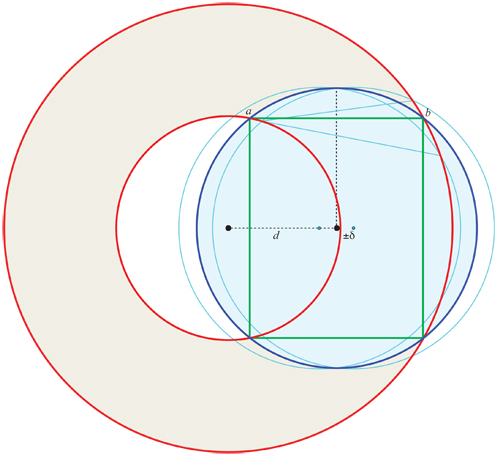
Your condition on $d$ ensures that the intersections $a$ and $b$ between circles
$C_3$ and $C_1$ and $C_2$ respectively, are at the same $y$-coordinate.
Then the different slopes of $C_1$ and $C_2$ at these points ensure that
the area is a local maximum.
Annulus & Circle http://cs.smith.edu/%7Eorourke/MathOverflow/AnnulusCircle.jpg