8
$\begingroup$

What is a good reference for the following result which I believe is proved by Tchebotarev.

There are exactly 5 types of Lunes that are squarable. (Hippocrates produced three and then two more were given by Euler).

Improve this question
$\endgroup$
1
  • $\begingroup$ @Chebolu: I added what I think are more appropriate tags (but left your original ag.algebraic-geometry tag). $\endgroup$
    – Joseph O'Rourke
    Commented Mar 27, 2012 at 12:26

2 Answers 2

Reset to default
6
$\begingroup$

"The Problem of Squarable Lunes." M. M. Postnikov, translated from the Russian by Abe Shenitzer. The American Mathematical Monthly. Vol. 107, No. 7 (Aug.-Sep., 2000), pp. 645-651. JSTOR link.

See also the MathPages web page entitled "The Five Squarable Lunes," and the Wikipedia page "Lune of Hippocrates," from which this image of the "lunes of Alhazen" is taken:
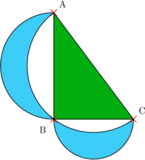
           Lunes
"The two blue lunes together have the same area as the green right triangle."

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ Note, however, that the Postnikov proof involves (p. 647) a statement whose proof "is beyond the level of this book." $\endgroup$
    – John Stillwell
    Commented Mar 27, 2012 at 0:15
  • $\begingroup$ @John: Interesting; I didn't notice that. The statement is that a certain single-variable polynomial of potentially high degree cannot, except in one special case, be reduced to the solution of quadratic equations. $\endgroup$
    – Joseph O'Rourke
    Commented Mar 27, 2012 at 13:48
3
$\begingroup$

This is indeed the theorem of Chebotarev and Dorodnov, the original articles are

Tschebotaröw, Nikolaj Über quadrierbare Kreisbogenzweiecke. I. (German) Zbl 0010.00103. Math. Z. 39, 161-175 (1934).

A. V. Dorodnov, On circular moonlets which are quadrable by compass and ruler (Russian) Zbl 0030.10302. Dokl. Akad. Nauk SSSR, II. Ser. 58, 965-968. (1947)

There is also a Russian translation of Chebotarev's paper in his Complete Works, vol. 1, p. 193-207. Dorodnov's paper is available only in Russian, and unfortunately cannot be found on Internet (he was a student of Chebotarev, and completed Chebotarev's proof).

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .