Let $f(x)=\sin x$, and $g(x)=\sin x + 1$. Consider a set $S=\{(x,y)| f(x)\leq y \leq g(x), x\in [0,2\pi]\}$. This set $S$ can be considered as "Raceway" My question is finding the shortest path in $S$ such that initial point lies in $\{0\}\times [0,1]$, and the terminal point lies in $\{2\pi\}\times [0,1]$.
$\begingroup$
$\endgroup$
5
-
$\begingroup$ Could you say a little bit more about where the problem comes from? What's the shortest length that you have been able to obtain? $\endgroup$– Yemon ChoiCommented Feb 15, 2012 at 3:57
-
$\begingroup$ (It is clear what to do for $0\leq x\leq \pi/2$ and $3\pi/2\leq 2\pi$, and there is a natural guess for what to do for $\pi/2\leqx\leq 3\pi/2$, but without checking or thinking further it's not clear to me how to prove that patching together these three paths gives a global minimum for the total path length.) $\endgroup$– Yemon ChoiCommented Feb 15, 2012 at 3:57
-
$\begingroup$ If you try to "peel" the middle section away from the sine curve, I think you will find the x coordinate goes away from the outer sections (because of the slope of the tangent being negative). While I am not willing to do the arithmetic, I think a global minimum follows from Yemon's hints and mine. Also, this is more appropriate for math.stackexchange. Gerhard "Ask Me About System Design" Paseman, 2012.02.14 $\endgroup$– Gerhard PasemanCommented Feb 15, 2012 at 6:02
-
$\begingroup$ Gerhard, I am not so sure it belongs better on MSE, my feeling is that there it would attract many eager but incomplete answers. $\endgroup$– Yemon ChoiCommented Feb 15, 2012 at 7:25
-
$\begingroup$ Yemon, perhaps so. I still have the feeling that this could be (or was) posed as a homework problem in an advanced high school calculus course. ArtofProblemSolving website then? Gerhard "Ask Me About System Design" Paseman, 2012.02.15 $\endgroup$– Gerhard PasemanCommented Feb 16, 2012 at 1:15
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
4
Just an illustration of the question:
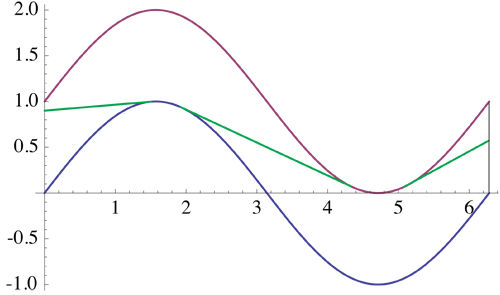
-
$\begingroup$ Although one could have a shorter path by going from $(0,0)$ to $(\pi/2,0).$ and from $(3\pi/2,0)$ to $(2\pi,0).$ The middle linear segment might go from approximately $(1/2+0.117)\pi$ to $(3/2-0.117)\pi.$ It seems obvious that anything differing from this can be made shorter. $\endgroup$ Commented Feb 15, 2012 at 18:10
-
$\begingroup$ @Aaron: I interpreted the question (perhaps incorrectly) as having a given point specified within the possible start interval, and a given point specified within the possible termination interval. But I can see another interpretation is to minimize over all starting and ending points. $\endgroup$ Commented Feb 15, 2012 at 18:50
-
$\begingroup$ @Joseph: Thank you for posting picture to my problem. I originally thought about this problem long ago, and I thought straight line segments for $[0,\pi/2]$, and $[3\pi/2,2\pi]$, and a sine curve in the middle section would be shortest, but I am not sure that it is the shortest one. $\endgroup$ Commented Feb 16, 2012 at 3:50
-
1$\begingroup$ Ah, so I did misinterpret the problem as starting and ending anywhere on those vertical segments. As Aaron says, those three straight segment tangents plus a portion of the curves is clearly the shortest. And starting anywhere is just a small variation: the middle segment is the same, and the two end segments are tangents as illustrated. $\endgroup$ Commented Feb 16, 2012 at 11:10
