6
$\begingroup$

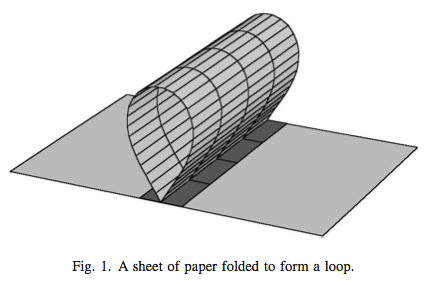
I take an rectangular sheet of paper, of height $H$ and Young's Modulus $E$, and in the absence of gravity, I bend it into a "teardrop" shape so that the edges along the top and bottom touch only along a single line.

What analytic function describes this "teardrop" shape?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Are you specifying the boundary condition that the tangent cones of the edges coincide, or that they form some specified dihedral angle? $\endgroup$
    – S. Carnahan ♦
    Commented Jan 24, 2012 at 23:45
  • 1
    $\begingroup$ You may find this question on MSE useful: math.stackexchange.com/questions/51539/… $\endgroup$
    – Samuel Reid
    Commented Jan 25, 2012 at 0:02

1 Answer 1

Reset to default
8
$\begingroup$

All in here (if using Euler Bernoulli beam theory)

http://sci-toys.com/bent_paper_problem.pdf

(Added by Joseph O'Rourke). Here is Fig.1 from the paper by Antoni Colom, "Analysis of the shape of a sheet of paper when two opposite edges are joined," PDF link above.
         Tear Drop

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ @Paul: I took the liberty of adding detail to your answer. $\endgroup$
    – Joseph O'Rourke
    Commented Jan 25, 2012 at 1:34

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .