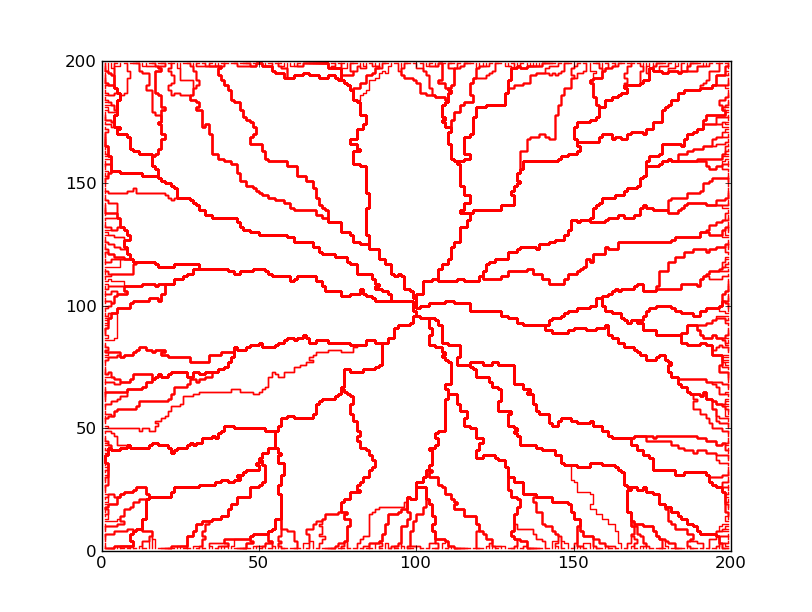
Consider the last passage percolation model on $\mathbb{Z}^2$ with, say, geometric weights on each edge. By a landmark result of Johansson (http://arxiv.org/abs/math/9903134), we know that if $T_n(\alpha)$ is the passage time (or distance) between the origin and the point of coordinates $(\alpha n, n)$, then $$ \frac{T_n(\alpha) - \omega(\alpha) n}{\sigma(\alpha)n^{1/3}}\to X, $$ where $X$ has the Tracy-Widom distribution. Here $\omega(\alpha), \sigma(\alpha)$ are two constants whose value is known and is not important for this question.
I am interested in the following natural question:
Q1) Given $\alpha, \beta$, and letting $\gamma_n(\alpha)$ denote the geodesic between 0 and $(\alpha n, n)$, how many edges do $\gamma_n(\alpha)$ and $\gamma_n(\beta)$ share?
Intuitively, one possible way to approach this question is to first ask
Q2) How big is the covariance between between $T_n(\alpha)$ and $T_n(\beta)$?
The reason why these two questions seem related is that one would expect $\text{cov}(T_n(\alpha), T_n(\beta)) $ to be roughly proportional to the number of edges on $\gamma_n(\alpha)\cap \gamma_n(\beta)$. (At least this is what happens for deterministic paths).
Presumably, Johannson's result tells us that var$(T_n(\alpha))$ is of order $n^{2/3}$ (though it's not a straightforward consequence of that result), so Cauchy-Schwarz implies that the covariance is at most of order $n^{2/3}$. This would suggest that $|\gamma_n(\alpha)\cap \gamma_n(\beta)|$ is at most of order $n^{2/3}$. However, it is hard to believe that this is sharp!
Does anyone know if these questions have been studied ? And what if we only know that $\text{var} (T_n(\alpha)) = o(n)$ (as in this paper, Link, by Benjamini Kalai and Schramm), does it follows that $|\gamma_n(\alpha) \cap \gamma_n(\beta)| = o(n)$?