What is the largest possible volume of the convex hull of an open/closed curve of unit length in $\mathbb{R}^3$?
$\begingroup$
$\endgroup$
6
asked Dec 9, 2011 at 3:47
-
$\begingroup$ If the optimal object is not a round sphere then the answer is not known. (There are few exceptions, but it is almost true.) $\endgroup$– Anton PetruninCommented Dec 9, 2011 at 4:14
-
4$\begingroup$ It is definitely not a sphere. $\endgroup$– Vladimir ReshetnikovCommented Dec 9, 2011 at 4:22
-
1$\begingroup$ I nominate a fractional orange slice: A slightly warped semicircular arc and part of a second one in a plane perhaps 120 degrees from the first one, sharing common end points. I leave the details to those with the computational power. Gerhard "Not Ready To Compute Volumes" Paseman, 2011.12.08 $\endgroup$– Gerhard PasemanCommented Dec 9, 2011 at 5:42
-
$\begingroup$ related: mathoverflow.net/questions/26212 $\endgroup$– Steve HuntsmanCommented Dec 9, 2011 at 22:26
-
$\begingroup$ @Gerard: I like your answer (an attempt :-). I could apply your way to my attempt below by adjusting the angles of arcs which at this time are set rigidly along being roughly parallel. $\endgroup$– Włodzimierz HolsztyńskiCommented Aug 22, 2014 at 19:58
|
Show 1 more comment
3 Answers
$\begingroup$
$\endgroup$
I believe this problem has been mentioned a few times in the literature, and has been solved for certain restrictions on the curve. For example if the curve has no four coplanar points then the maximal volume is achieved by one turn of a circular helix of height $\frac{1}{\sqrt{3}}$ and base radius $\frac{1}{\pi\sqrt{6}}$, this is due to Egervary. For more references see section A28 of "Unsolved problems in geometry" by H.T. Croft, K.J. Falconer, R.K. Guy. Melzak and Schoenberg have treated the corresponding problem for closed loops (Schoenberg has treated even dimensions), and have given answers under similar restrictions. In no case is a complete answer known.
answered Dec 9, 2011 at 6:27
$\begingroup$
$\endgroup$
2
Here is an image of the optimal open convex curve. Taken from Open Problems from CCCG 2012, based on this paper, which cites Nudel'man (1975):
Paolo Tilli. "Isoperimetric inequalities for convex hulls and related questions." Trans. Amer. Math. Soc. 362 (2010), 4497-4509.
answered Aug 23, 2014 at 1:02
-
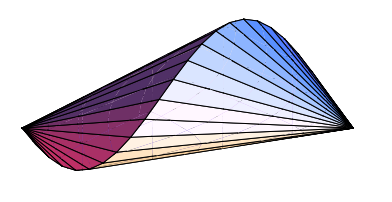
1$\begingroup$ This is the same as the curve mentioned in my answer, right? I believe the reference goes further back than 2010 :) $\endgroup$ Commented Aug 23, 2014 at 9:10
-
$\begingroup$ Yes, this is the curve you mention. And you are right about the references: This was proved by Nudel'man in 1975. Tilli says that proving this is optimal among all curves is open as of 2010. $\endgroup$ Commented Aug 23, 2014 at 13:02
$\begingroup$
$\endgroup$
In the case of a closed curve my first wild (but educated :-) guess would be: connect the following $\ 8\ $points (vertices of a cube but also belonging to a sphere) in the given cyclic order (of a maximal shift register):
$$(-a\ -\!a\ -\!a)\quad(-a\ -\!a\ +\!a)\quad(-a\ +\!a\ +\!a)\quad(-a\ +\!a\ -\!a)$$ $$(+a\ +\!a\ -\!a)\quad(+a\ +\!a\ +a)\quad(+a\ -\!a\ +\!a)\quad(+a\ -\!a\ -\!a) $$
where $\ a\ $ is such that the length of the large arc which connects the consecutive points on the sphere which contains these $\ 8\ $ points is $\ \frac18$.
EDIT Shooting from the hip cannot be that precise. Thus two related questions may help some:
What is a maximal volume of a convex hull of a closed curve contained in a sphere? (Then the respective radius would be of interest).
Is there such a curve but not spherical which encloses a larger volume (i.e. its convex hall would be larger than for any closed curve contained in a sphere)?
answered Aug 22, 2014 at 19:31