12
$\begingroup$

I was looking at some random problems and questions I liked when I was in high school and I found this one which I still cannot prove.

Does there exist a configuration of a countable number of straight lines in the plane such that:

1) no two are parallel

2) no three are concurrent

3) any bounded subset of the plane is intersected by a finite number of lines

4) the area of every minimal polygon is equal, where a minimal polygon is a polygon formed by a finite subset of the set of lines such that no lines pass through the inside of the polygon.

The answer is certainly no, but it is not that easy to prove. Any ideas?

Improve this question
$\endgroup$
6
  • 1
    $\begingroup$ What exactly is a minimal polygon? (minimal with respect to area?) It seems to me that 4) is too demanding (if my understanding of minimal is correct). The answer is probably "yes" if you ask that every polygon as in 4) has area smaller than some constant $C$. $\endgroup$
    – Roland Bacher
    Commented May 31, 2011 at 11:43
  • $\begingroup$ I'm not even sure the answer should be no. Surely you are requiring a lot of conditions, but it still doesn't strike me as totally counter-intuitive… $\endgroup$
    – Ale De Luca
    Commented May 31, 2011 at 11:55
  • $\begingroup$ For an infinite arrangement of lines satisfying 1,2,& 3 some portion of the plane will be tiled by minimal polygons (many tangent lines to a parabola shows that there can be unbounded regions as well.) Is it clear that there can be large complex regions made up of such polygons all equal in area? (Perhaps one can start from sets of 2m equally spaced parallel lines, m horizontal and m vertical and perturb slightly, but perhaps not). And if that can be done, can it be done in such a way that when the boundary lines are extended none of the polygons so created are smaller than the given ones? $\endgroup$
    – Aaron Meyerowitz
    Commented May 31, 2011 at 18:27
  • 2
    $\begingroup$ A) Is it always true that for a pentagonal star, one of the 5 triangles has area strictly smaller than the central pentagon? (Can assume the pointy tips lie on a circle if it helps, or else that 2 sides are orthogonal and of length 1.) B) that should prove that any equal area tiling could can only consist of triangles and quadrilaterals... C) but then what? D) Love the question! Seemed like such an easy, overconstrained no-brainer! $\endgroup$
    – Yaakov Baruch
    Commented May 31, 2011 at 19:45
  • $\begingroup$ @Roland Bacher By minimal polygon I mean a polygon which does not have any segments inside of it. Which is the same as to say minimal with respect to area, i.e. a polygon D is minimal if there is no polygon of smaller area contained in D. $\endgroup$
    – Manuel Rivera
    Commented Jun 1, 2011 at 0:05

1 Answer 1

Reset to default
13
$\begingroup$

I can sketch a proof based on assuming this "finite" result:

A). For any pentagonal star one of the 5 triangles will have area strictly smaller than that of the central pentagon. (I think a brute force attack should yield a proof here.) star

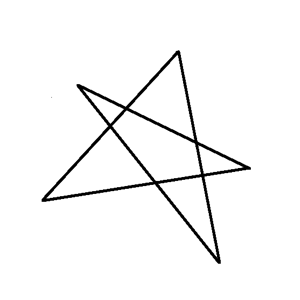
The proof of the original problem would then go as follows.

b). A) generalizes to n-agons by considering the pentagon spanned by any 5 vertices. hexagon

c). b) implies that a tiling with polygons of EQUAL AREA is not possible unless all polygons are either triangles or quadrilaterals.

d). Take one 4-tile and continue tiling next to it inside the cone enclosed by the converging lines of 2 opposite edges; we have a sequence of quadrilaterals which must end with a triangle were the the 2 lines meet. This shows that the tiling must contain a 3-tile somewhere.

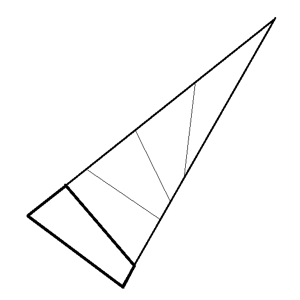
4-tile

e). By d) take a 3-tile and continue tiling outwards, inside each of the 3 beams generated by the lines of each pair of edges; the original 3-tile will be the first tile in each beam, but every other tile after it must be a 4-tile (build them one at a time and keep using c)). We can ignore what happens in the 3 leftover cones radiating from the 3 vertices.

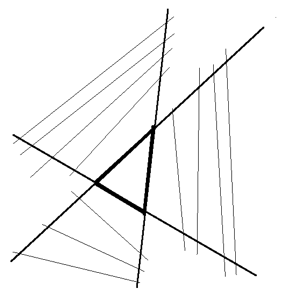
3-tile

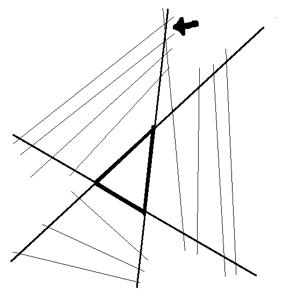
f). In one of the 3 beams (which now look like ladders) take any one of the new rungs from step e) and extend it - that line will then collide with one of the other 2 beams (but cannot overlap with any of its rungs). That will cut one of the 4-tiles, creating a 5-tile.

contradiction!

Apologies for bumping up the question repeatedly while trying to edit my answer.

Improve this answer
$\endgroup$
6
  • $\begingroup$ what a nice story! $\endgroup$
    – Pietro Majer
    Commented Jun 1, 2011 at 19:03
  • $\begingroup$ very nice proof! $\endgroup$
    – Olivier Bégassat
    Commented Jun 1, 2011 at 22:26
  • 2
    $\begingroup$ Re. the pentagonal star, one can wiggle around any one of the 5 lines in such a way that the pentagon and 3 triangles keep the same area, while the other 2 triangles are equalized. Therefore one can assume all 5 triangles to have the same area. Forcing this condition on the pentagon with vertices (1,0), (0,0), (0,1), (a,b), (c,d) can easily be seen to determine a, b, c and d. Therefore, up to affine transformation, the unique star whose triangles have equal area is the regular star. $\endgroup$
    – Yaakov Baruch
    Commented Jun 18, 2011 at 23:33
  • 1
    $\begingroup$ It looks like ImageShack has decided to delete your images and replace them with ad banners. If you can, please reupload your images using the upload button in the editor toolbar. $\endgroup$
    – Ilmari Karonen
    Commented Aug 18, 2015 at 11:41
  • 1
    $\begingroup$ @IlmariKaronen: done. Thank you for pointing it out to me. $\endgroup$
    – Yaakov Baruch
    Commented Aug 19, 2015 at 2:38

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .