Surfaces
When one parameter curves are given a particular polar symmetric situation can be handled. On an arbitrary axisymmetric patches with polar symmetric geodesics with a common Clairaut's constant $ r_o$ minimum radius of fiber tangency obey differential equation:
$$ r_o= r \, \sin \psi= const $$
Orthogonal trajectories of these geodesic family are given by $ \psi\rightarrow \pi-\psi $
$$ r_b= r \, \cos \psi= const $$
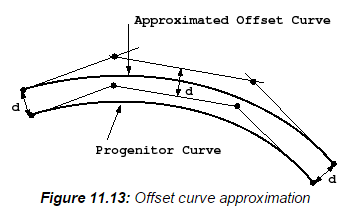
which can be called 3D involutes as orthogonal trajectories. On the following surface, 2D geodesics can be seen on the outside and equidistant tubes drawn for 3D clarity on the inside.
Just as in the plane case, the width of cyclic involutes is constant, a concept inherent with radial geodesic polar coordinate parameter.. right from Leibnitz's time.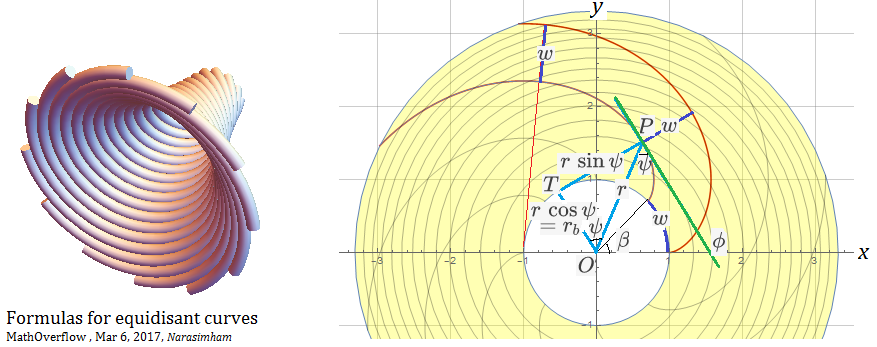
Planar case
Involutes of circle ( we know how it is constructed using a string ) have constant width marked $w$ along common normal brought on from originating base circle circumference arc.
The base circle radius is an invariant of transformation. Width $w$ is seen as a fraction of $r_b$.
$$ r_b = r \cos \psi \tag1 $$
Differentiating w.r.t arc
$$ \psi ^{'}= \frac {\cos^2\psi}{r\, \sin \psi} \tag2 $$
Curvature of involute
$$ = \phi^{'}= (\psi^{'}+ \theta^{'}) =(\psi^{'}+ \frac{\sin \psi}{r}) = \frac {\cos^2\psi}{r\, \sin \psi}+\frac{\sin \psi}{r}=\frac {1}{r\, \sin \psi}= \frac{1}{TP} \tag3$$
$$ r^2 = {(r\, \sin \psi)}^2+{(r\, \cos \psi})^2= TP^2 + OT^2\tag4$$
$$ Width = w = T_1T_2 = r_b\, \beta = 2 \pi r_b\, \frac{\beta}{2 \pi} \tag5 $$
is constant because for the taut unwinding part of string it is shown circumferentially carried outward along involute arc.