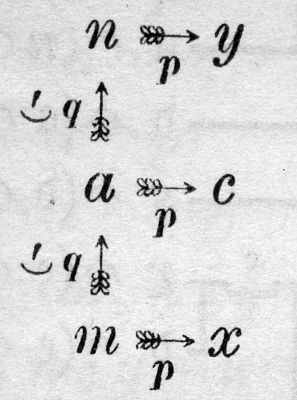whence commutative diagrams?

Question

It seems that commutative diagrams appeared sometime in the late 1940s -- for example, Eilenberg-McLane (1943) group cohomology paper does not have any, while the 1953 Hochschild-Serre paper does. Does anyone know who started using them (and how they convinced the printers to do this)?

Answer 1

I can muddy the waters...!

According to editor E. Scholz of Hausdorff’s Collected Works (2008, p. 884):

In a note of 3/20/1933 (Nachlass, fasc. 449) and in a further undated note (fasc. 571), Hausdorff symbolized the functoriality property of homology (in our later terminology) with a commutative diagram of homomorphisms between the terms of two sequences of groups $(A_n)_{n\in\mathbf N}$, $(A'_n)_{n\in\mathbf N}$: (Nachlass, fasc. 571, leaf 1).

Could this have, somehow, made its way out of Bonn (where Hausdorff lectured on combinatorial topology that year) and to Hurewicz, Eilenberg, Steenrod, et al.? This seems not impossible, as e.g. Tucker (1932, footnote 4) mentions discussion of Hausdorff letters in the Princeton seminar. Maybe also noteworthy: Hausdorff’s colleague and mentor in Bonn was Eduard Study, author of another early commutative diagram.

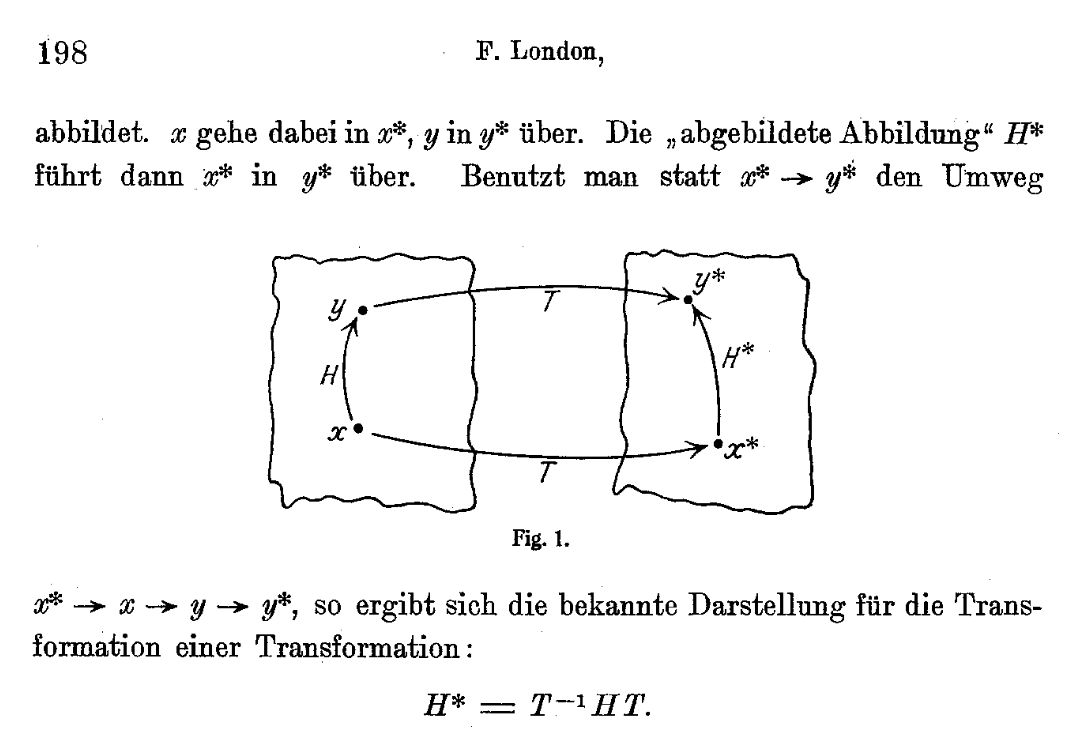
Addition: Two years later, essentially the same “schema” is in print, p. 151 of H. Freudenthal, Die Hopfsche Gruppe, eine topologische Begründung kombinatorischer Begriffe, Compos. Math. 2 (1935) 134-162. And six years earlier, Fritz London (son of yet another Bonn mathematician) already had, in Winkelvariable und kanonische Transformationen in der Undulationsmechanik, Z. f. Physik 40 (Dec. 1926) 193-210:

(For the story of the arrows themselves, see the relevant questions on $\to$ and $\mapsto$.)

Answer 2

An excerpt from Mac Lane's Categories for the Working Mathematician (p29, Notes on Chapter 1):

The fundamental idea of representing a function by an arrow first appeared in topology about 1940, probably in papers or lectures by W. Hurewicz on relative homotopy groups; see [1941].

His initiative immediately attracted the attention of R.H. Fox (see Fox [1943]) and N.E. Steenrod, whose [1941] paper used arrows and (implicitly) functors; see also Hurewicz-Steenrod [1941]. The arrow $f: X\to Y$ rapidly displaced the occasional notation $f(X)\subset Y$ for a function. It expressed well a central interest of topology. Thus a notation (the arrow) led to a concept (category).

Commutative diagrams were probably also first used by Hurewicz.

Categories, functors and natural transformations themselves were discovered by Eilenberg-Mac Lane [1942a] in their study of limits (via natural transformations) for universal coefficient theorems for Čech cohomology. In this paper commutative diagrams appeared in print (probably for the first time). Thus $\mathrm{Ext}$ was one of the first functors considered. A direct treatment of categories in their own right appeared in Eilenberg-Mac Lane [1945].

This last mentioned paper is the one referred to in KConrad's answer.

Answer 3

Eduard Study in Von den Bewegungen und Umlegungen, Math. Ann. 39 (1891) 441-566, writes on p. 508:

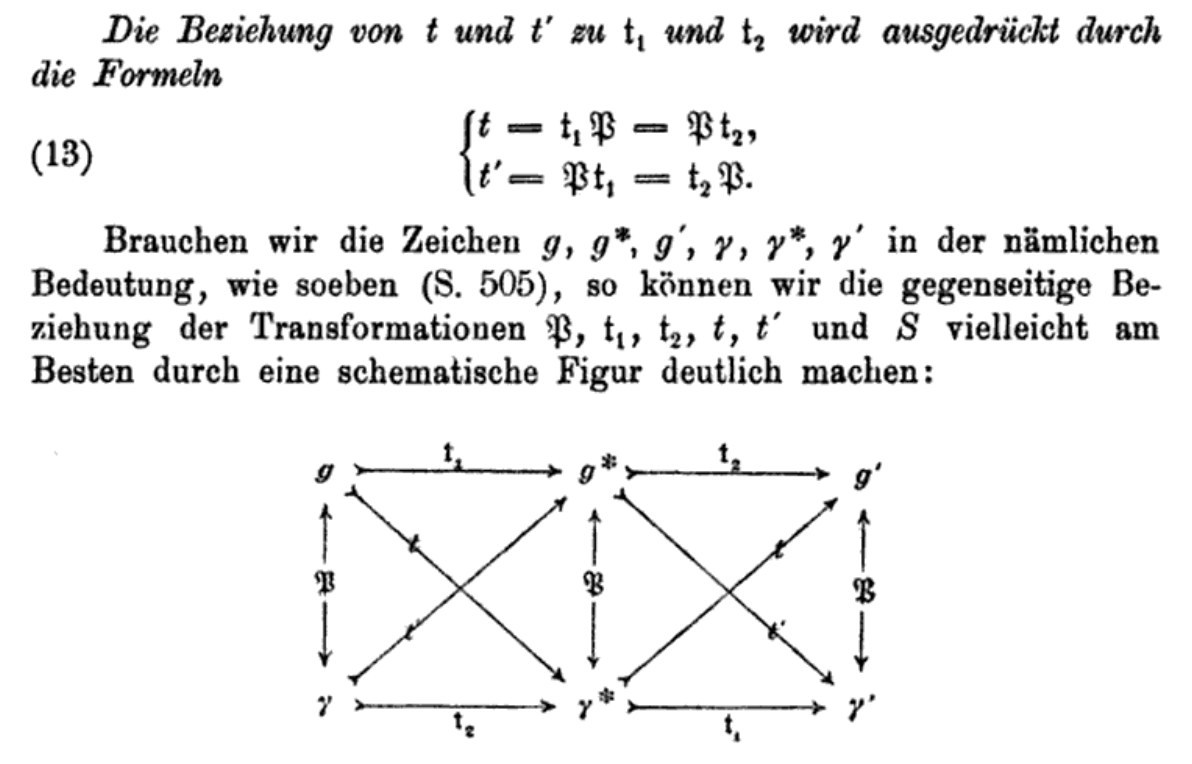
Here $g, g^*, g'$ are rays in space with polar planes $\gamma, \gamma^*, \gamma'$, $\mathfrak P$ is the “polarity” taking rays to planes and conversely, the $\mathfrak t_i$ are commuting collineations acting on both rays and planes, and Study uses $\mathfrak t_i\mathfrak P$ to denote the composition we would write $\mathfrak P\circ\mathfrak t_i$.

(Essentially the same diagram is repeated in Study’s book Einleitung in die Theorie der Invarianten linearer Transformationen auf Grund der Vektorenrechnung (1923, p. 217), with credit to (1891).)

Answer 4

There's Russell's example from 1919, see here where conjugacy between relations is expressed diagrammatically.

Answer 5

Commutative diagrams really show their significance when dealing with categories, so I would guess they first appeared in that context. Look at the paper which first introduced categories: Eilenberg and Mac Lane's "General Theory of Natural Equivalences" (Trans. AMS) 58 (1945), 231--294. You can find that paper through JSTOR, but if you don't have access to JSTOR then get it at http://killingbuddha.altervista.org/FILOSOFIA/GToNe.pdf. In this paper, commutative diagrams are used only a few times and the authors don't say the diagrams commute. Instead they use more elaborate descriptions like "the two paths around the diagram are equivalent". Even if this is not the first place a commutative diagram appeared, that they are used sparingly (considering the context) and described in an awkward way suggests it was one of the earliest times this concept jumped off the blackboard into print.

Weibel's paper on the history of homological algebra, which is available at his website, may provide some earlier sources if you track down references he gives.

EDIT: Perhaps the first book which made extensive use of commutative diagrams is Eilenberg and Steenrod's "Foundations of Algebraic Topology" (1952). On page xi, under the heading of a section they call New Methods, they introduce the term commutativity for certain diagrams of groups and homomorphisms.

Answer 6

Further to David Corfield's post: The diagram below appeared in Frege's 1893 Grundgesetze der Arithmetik (Vol. I, §§130, 172) and illustrates the construction of a mapping that Frege uses to prove that for any finite concept, the objects falling under it can be well-ordered.

Russell, of course, studied the Grundgesetze closely; perhaps he found inspiration in Frege's figure (if not the almost childish arrows).