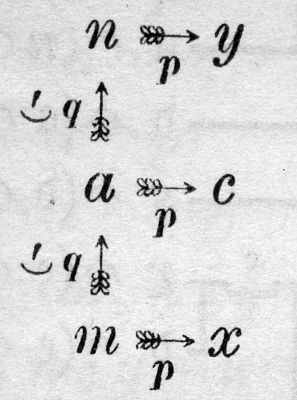Further to David Corfield's post: The diagram below appeared in Frege's 1893 Grundgesetze der Arithmetik (vol .1, §172Vol. I, §§130, 172) and illustrates the construction of a mapping that Frege uses to prove that for any finite concept, the objects falling under it can be well-ordered.
Russell, of course, studied the Grundgesetze closely; perhaps he found inspiration in Frege's figure (if not the almost childish arrows).