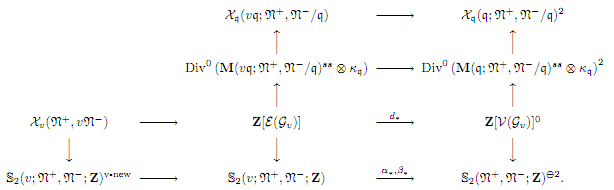Is it not possible to prove mutiplicity one type statements for character groups of quaternionic Shimura curves by simply using Raynaud's description for character groups at primes dividing the underlying discriminant?
To be slightly more precise, let $F$ be a totally real field of degree $d$. Fix ideals $\mathfrak{M}^+, \mathfrak{M}^{-} \subset \mathcal{O}_F$. Suppose that $\mathfrak{M}^{-}$ is the squarefree product of a number of primes congruent to $d-1 \mod 2$. Assume that $(\mathfrak{M}^+, \mathfrak{M}^{-}) =1$. Let $M(\mathfrak{M}^+, \mathfrak{M}^{-})$ denote the Shimura curve of level $\mathfrak{M}^+$ associated to the indefinite quaternion algebra of discriminant $\mathfrak{M}^{-}$. (Here, "indefinite" means ramified at all but one of the real places of $F$, hence the condition on $\mathfrak{M}^{-}$). Fix a prime $v \subset \mathcal{O}_F$ that does not divide $\mathfrak{M}^+ \mathfrak{M}^{-}$. Assume from now on that the level of $M(\mathfrak{M}^+, \mathfrak{M}^{-})$ is maximal at $v$. Then, there exists a nice integral model ${\bf{M}}(\mathfrak{M}^{+}, \mathfrak{M}^{-})$ of $M(\mathfrak{M}^+, \mathfrak{M}^{-})$ over $\mathcal{O}_{F_v}$ (due to Carayol in the case that $v \nmid \mathfrak{M}^{-}$, or due to combined works of Cerednik and Varshavsky in the case that $v \mid \mathfrak{M}^{-}$). Let $J(\mathfrak{M}^{+}, \mathfrak{M}^{-})$ denote the jacobian of $M(\mathfrak{M}^{+}, \mathfrak{M}^{-})$, with ${\bf{J}}(\mathfrak{M}^{+}, \mathfrak{M}^{-})$ its Neron model over $\mathcal{O}_{F_v}$, and ${\bf{J}}_v^0(\mathfrak{M}^{+}, \mathfrak{M}^{-})$ the component of the identity of its special fibre. Let $\mathcal{X}_v(\mathfrak{M}^{+}, \mathfrak{M}^{-})$ denote the character group of the maximal torus of ${\bf{J}}_v^0(\mathfrak{M}^{+}, \mathfrak{M}^{-})$. Additionally, given an ideal $\mathfrak{m} \subset \mathcal{O}_F$ that does not divide $\mathfrak{M}^+ \mathfrak{M}^{-}$, let $M(\mathfrak{m}; \mathfrak{M}+, \mathfrak{M}^{-})$ denote the Shimura curve $M(\mathfrak{M}^{+}, \mathfrak{M}^{-})$ with maximal level structure at primes dividing $\mathfrak{m}$ inserted (so, $M(\mathfrak{m}\mathfrak{M}^+, \mathfrak{M}^{-})$ is the Shimura curve of $\mathfrak{m}\mathfrak{M}^{+}$-level structure associated to the indefinite Shimura curve of discriminant $\mathfrak{M}^{-}$, with the extra condition that the level be maximal at primes dividing $\mathfrak{m}$).
Suppose now that we have ideals $\mathfrak{N}^+, \mathfrak{N}^{-} \subset \mathcal{O}_F$ such that $(\mathfrak{N}^+, \mathfrak{N}^{-}) =1$ and $\mathfrak{N}^{-}$ is the squarefree product of a number of primes congruent to $d \mod 2$. Given a ring $\mathcal{O}$, let $\mathbb{S}_2(\mathfrak{N}^{+}, \mathfrak{N}^{-}; \mathcal{O})$ denote the space of $\mathcal{O}$-valued automorphic forms of weight $2$ and level $\mathfrak{N}^+$ on the totally definite quaternion algebra of discriminant $\mathfrak{N}^{-}$ over $F$. Let $\mathbb{T}(\mathfrak{N}^+, \mathfrak{N}^{-})$ denote the associated algebra of Hecke operators. Given an ideal $\mathfrak{n} \subset \mathcal{O}_F$ that does not divide $\mathfrak{N}^+ \mathfrak{N}^{-}$, let $\mathbb{S}_2(\mathfrak{n}; \mathfrak{N}, \mathfrak{N}^{-}; {\bf{Z}})$ denote the space of forms of level $\mathfrak{n}\mathfrak{N}^+$, with the level being maximal at primes dividing $\mathfrak{n}$. Fix a prime $v \subset \mathcal{O}_F$ that does not divide the product $\mathfrak{N}^+ \mathfrak{N}^{-}$. Let us now take $\mathfrak{M}^+ = \mathfrak{N}^+$ and $\mathfrak{N}^{-} = v \mathfrak{N}^{-}$ in the Shimura curves setup above. In particular, consider the Shimura curve $M(\mathfrak{N}^+, v\mathfrak{N}^{-} )$, with $\mathcal{X}_v(\mathfrak{N}^+, v\mathfrak{N}^{-})$ the associated character group. Raynaud's theory gives a combinatorial description of $\mathcal{X}_v(\mathfrak{N}^+, v\mathfrak{N}^{-})$ in terms of the dual graph $\mathcal{G}_v = (\mathcal{V}(\mathcal{G}_v), \mathcal{E}(\mathcal{G}_v))$ of the special fibre of ${\bf{M}}(\mathfrak{N}^+, v\mathfrak{N}^{v})$. That is, fix an orientation $s, t: \mathcal{E}(\mathcal{G}_v) \longrightarrow \mathcal{V}(\mathcal{G}_v)$ of the dual graph. Then, by Raynaud's theory, there is a short exact sequence of ${\bf{Z}}$-modules
$$\begin{align*}\mathcal{X}_v(\mathfrak{N}^+, v\mathfrak{N}^{-}) \longrightarrow {\bf{Z}}[\mathcal{E}(\mathcal{G}_v)] \longrightarrow {\bf{Z}}[\mathcal{V}(\mathcal{G}_v)]^0. \end{align*}$$ that identifies the character group $\mathcal{X}_v(\mathfrak{N}^+, v\mathfrak{N}^{-})$ with the kernel of the coboundary/degeneracy map $d_* = s_* - t_*$. Let us now assume that $\mathfrak{N}^{-}\neq \mathcal{O}_F$. Fix a prime divisor $\mathfrak{q} \mid \mathfrak{N}^{-}$. Fixing a suitable orientation of the dual graph, and using various identifications (from the theory of Mumford-Kurihara uniformization on the bottom, then from Carayol's description supersingular points on the top), one can obtain the following diagram, where the vertical arrows are all isomorphisms:
Some details aside (for brevity -- and compilation issues), the important extraction to make here is that of the induced short exact sequence of $\mathbb{T}(v ; \mathfrak{N}^+, \mathfrak{N}^{-})$-modules
$$\begin{align*} \mathcal{X}_v(\mathfrak{N}^{+}, v\mathfrak{N}^{-}) \longrightarrow \mathcal{X}_{\mathfrak{q}}(v\mathfrak{q}; \mathfrak{N}^+, \mathfrak{N}^{-}/\mathfrak{q}) \longrightarrow \mathcal{X}_{\mathfrak{q}}(\mathfrak{q}; \mathfrak{N}^+, \mathfrak{N}^{-}/\mathfrak{q})^2.\end{align*}$$ In particular, via the induced isomorphism $\mathcal{X}_{\mathfrak{q}}(\mathfrak{q}; \mathfrak{N}^+, \mathfrak{N}^{-}/\mathfrak{q}) \cong \mathbb{S}_2(\mathfrak{N}^+, \mathfrak{N}^{-}; {\bf{Z}})$, the character group $\mathcal{X}_{\mathfrak{q}}(\mathfrak{q}; \mathfrak{N}^+, \mathfrak{N}^{-}/\mathfrak{q})$ inherits the structure of a $\mathbb{T}(\mathfrak{N}^+, \mathfrak{N}^{-})$ module. So, is it not clear (e.g. by Jacquet-Langlands plus multiplicity one for $\operatorname{GL}_2$) that $\mathcal{X}_{\mathfrak{q}}(\mathfrak{q}; \mathfrak{N}^+, \mathfrak{N}^{-}/\mathfrak{q})$ is free of rank $1$ over $\mathbb{T}(\mathfrak{N}^+, \mathfrak{N}^{-})$? Moreover, suppose we take any maximal ideal $\mathfrak{m} \subset \mathbb{T}(\mathfrak{N}^+, \mathfrak{N}^{-})$. Let $\mathcal{X}_{\mathfrak{q}}(\mathfrak{q}; \mathfrak{N}^+, \mathfrak{N}^{-}/\mathfrak{q})_{\mathfrak{m}}$ denote the localization of $\mathcal{X}_{\mathfrak{q}}(\mathfrak{q}; \mathfrak{N}^+, \mathfrak{N}^{-}/\mathfrak{q})$ at $\mathfrak{m}$. Let $\mathbb{T}(\mathfrak{N}^+, \mathfrak{N}^{-})_{\mathfrak{m}}$ denote the localization of $ \mathbb{T}(\mathfrak{N}^+, \mathfrak{N}^{-})$ at $\mathfrak{m}$. Is it not also clear that $\mathcal{X}_{\mathfrak{q}}(\mathfrak{q}; \mathfrak{N}^+, \mathfrak{N}^{-}/\mathfrak{q})_{\mathfrak{m}}$ is free of rank $1$ over $\mathbb{T}(\mathfrak{N}^+, \mathfrak{N}^{-})_{\mathfrak{m}}$?