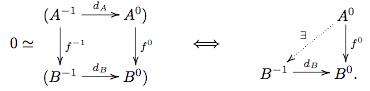Is anything known about the "closure" of an additive category by adding all the images and kernels?

Question

It is possible well-known: is there a "minimal" pre-abelian (or abelian) category, containing the given additive category, or which conditions should be performed for this property?

For example, let us take a category of vector bundles over a given manifold. Then, we can include it into a category of sheaves on this manifold, and close it there taking kernels and cokernels, finally getting the category of perfect sheaves (sheaves with finite resolution).

Overlooking the size of the category of vector bundles and all the sheaves, we can see that this construction somehow is similar as trying to find $\mathbb{Q} \subset \mathbb{R}$, knowing $\mathbb{Z} \subset \mathbb{R}$ inclusion.

Maybe any abstract procedure for this "closure" exists, or there are many "minimal" pre-abelian categories?

Idea 1. It may be possible to define an universal property of this category. For example, this might work: "after gluing all the isomorphic objects together, if $V$ is our additive category, $V \hookrightarrow A$ is it's closure and $B$ is other pre-additive category, equipped by morphism $V \hookrightarrow B$ there are $A \longrightarrow B$, commuting with previous two morphisms".

Idea 2. I've tried to construct this category directly. If we know a left resolution for an object $X$, we know $Hom(X, A)$. If we know right resolution, we know $Hom(A, X)$. Left resolution and right resolution together is an exact sequence $0 \rightarrow L_n ... \rightarrow L_1 \rightarrow R_1 ... \rightarrow R_n \rightarrow 0$.

Now let us assume that we actually know what an exact sequence is. Than we can add to our category a new object, corresponding to the one of the morphisms of a given exact sequence, and define Hom's to this object and from this object.

What's with the composition? Let us work with a sequence of length four (which will simplify our calculations). I don't know anything about the case of long sequences.

So, having a sequence $0 \rightarrow A \rightarrow B \rightarrow C \rightarrow D \rightarrow 0$ we imeddiately add a new object $X$, equipped with

$0 \rightarrow A \rightarrow B \rightarrow X \rightarrow 0$

and

$0 \rightarrow X \rightarrow C \rightarrow D \rightarrow 0$

Then, watching the properties of $Hom$, for any object S we define $Hom(S, X)$ by an exact sequence $0 \rightarrow Hom(S, X) \rightarrow Hom(S, C) \rightarrow Hom(S, D)$ (and $Hom(X, S)$ dually).

It's easy to see that $Hom(X, X)$ is well-defined.

There are also no problems with compisition of $Hom(X, S)$ and $Hom(S, T)$ for any $S$ and $T$. We just lift $Hom(X, S)$ to the $Hom(B, S)$, compose them and check that it's well-defined.

But how can we compose morphism of $Hom(S, X)$ and $Hom(X, T)$? Both parts of our exact sequence participate in the definition of this sets! So we need a some kind of condition on our "exact sequence".

I think I know what to do with it.

But maybe this is a well-known construction? Thanks for answering.

Answer 1

Note: I'm only addressing David's question how one one can "add cokernels" to an additive category and how one can use this in order to embed an additive category into an abelian one, even in a universal way.

Concerning the request on minimality in the question, it is unclear to me what exactly it is, Lev wants to achieve. What happens in the mentioned example of sheaves that one identifies an interesting category (vector bundles = finitely generated projective $\mathcal{O}_{X}$-modules) and ends up with the category coherent sheaves. In this case, the category constructed below embeds into the category of sheaves just because one started out with a class of projective objects in an abelian category already.

---

The idea is to embed the additive category $\mathcal{A}$ into the category of morphisms $\mathcal{A}^{\to}$. Note that a morphism in $\mathcal{A}^{\to}$ corresponds to a commutative square. More precisely, the idea is that a morphism $(A \to B)$ of $\mathcal{A}$ should represent its cokernel.

Let's pretend this works. Since the cokernel of $0 \to A$ in $\mathcal{A}$ is $A$, we see that we must embed $\mathcal{A}$ via $A \mapsto (0 \to A)$. This embedding of $\mathcal{A} \to \mathcal{A}^{\to}$ is clearly fully faithful. But we're not quite there, yet. There are nonzero morphisms of morphisms $(A^{-1} \to A^{0}) \to (B^{-1} \to B^{0})$ that should induce the zero map on the (putative) cokernels, namely precisely those for which the map $A^{0} \to B^{0}$ factors over $B^{-1}$:

It is easy to see that these morphisms form an ideal $\mathcal{J}$ in $\mathcal{A}^{\to}$ (they are closed under composition and sums), so we may factor this ideal out. In other words, a morphism of $\mathcal{A}^{\to}$ is identified with zero if and only if it lies in $\mathcal{J}$. The resulting category $\text{fp}(\mathcal{A}) = \mathcal{A}^{\to}/\mathcal{J}$ is what we want because we have:

Theorem (Freyd, 1965; Beligiannis, 2000)

1. The category $\text{fp}(\mathcal{A})$ has cokernels. The functor $A \mapsto (0 \to A)$ is fully faithful and universal among functors to additive categories with cokernels: more precisely, every functor $F: \mathcal{A} \to \mathcal{C}$ to a category with cokernels extends uniquely to a cokernel-preserving functor $\text{fp} (\mathcal{A}) \to \mathcal{C}$.
   
2. Moreover, $\text{fp}{(\mathcal{A})}$ is abelian if and only if $\mathcal{A}$ has weak kernels (a weak kernel has the factorization property of a kernel but uniqueness of the factorization is not required).

So if $\mathcal{A}$ has weak kernels, we're already done. If not, we may play the same game again, using this theorem. We first embed the category with kernels $(\text{fp} \mathcal{A})^{\text{op}}$ into the abelian category $\text{fp}\left( (\text{fp} \mathcal{A})^{\text{op}} \right)$ and then pass to the opposite category $\mathcal{F}{(\mathcal{A})} = \left(\text{fp}\left( (\text{fp} \mathcal{A})^{\text{op}} \right)\right)^{\text{op}}$. The diagrams get a bit unwieldy, but one may check that:

Theorem (Adelman, 1971) The embedding $\mathcal{A} \to \mathcal{F}(\mathcal{A})$ is fully faithful and universal among all functors to abelian categories (every functor to an abelian category extends to an exact functor on $\mathcal{F}(\mathcal{A})$).

The category $\text{fp}(\mathcal{A})$ is sometimes used in connection with the derived category/triangulated categories (the inclusion $\mathcal{T} \to \text{fp}(\mathcal{T})$ is the universal homological functor on the triangulated category $\mathcal{T}$. It already appears in Verdier's thesis - attributed to Freyd). Closely related are also the hearts of $t$-structures (perverse sheaves).

The most important references are:

1. Peter Freyd, Representations in abelian categories, Proc. Conf. Categorical Algebra (La Jolla, Calif., 1965), Springer, New York, 1966, pp. 95–120.

2. A. Beligiannis: ''On the Freyd Categories of an Additive Category'', Homology, Homotopy and Applications Vol. 2, No.11 (2000), pp. 147-185.