I am currently reading the paper Virtual Cartier divisors and blow-ups where the virtual Cartier divisor on an $X$ scheme $S$ over a quasi-smooth closed immersion $Z\rightarrow X$ is defined to be the quasi-smooth closed immersion of virtual codimension one $i_{D}: D\rightarrow S$ fitting in to the commutative diagram of derived schemes:
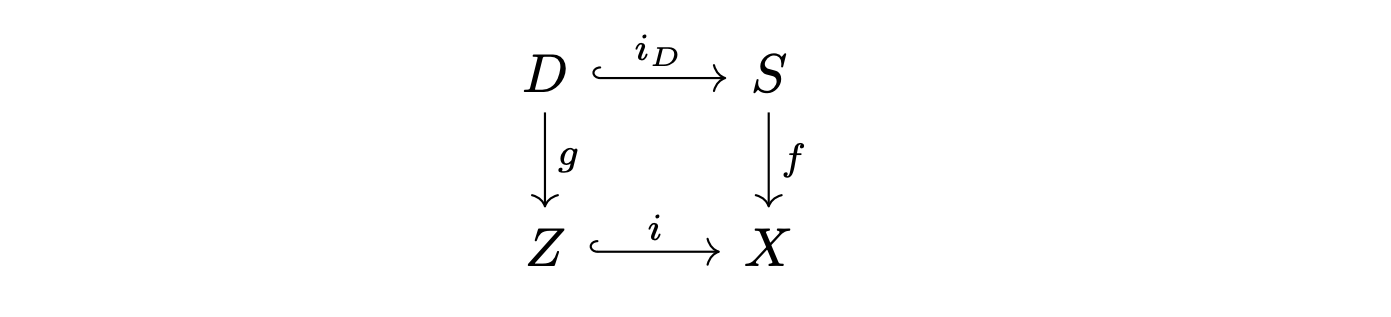satisfying the following conditions:
(1). The underlying square of classical schemes is a Cartesian diagram.
(2). The induced morphism of conormal sheaf $g^{*}N_{Z/X}\rightarrow N_{D/S}$ is surjective on $\pi_{0}$. Here the conormal sheaf of a closed immersion $Z\rightarrow X$ is defined to be the shifted cotangent complex $L_{Z/X}[-1]$.
Then we can define the derived blow-up $Bl_{Z}X$ as the stack sending $S\in dSch_{X}$ to the space of virtual Cartier divisors lying over $(Z,X)$, which is represented by a derived scheme by theorem 4.1.5 in the paper. The conditions of virtual Cartier divisors can be deduced to the conditions on the induced morphism $D\rightarrow S\times_{X} Z$ (as shown in Remark 4.1.3 of the above paper), thus the space of virtual Cartier divisors can be viewed as a subspace of $dSch_{S\times_{X} Z}^{\simeq}$ which discards all the noninvertible morphisms between them.
And also there is a conclusion that the derived blow up is represented by a derived scheme, and thus we can say that the the space of Cartier divisor on classical scheme $S$ is $\textbf{discrete}$ because $Map_{dSch_{X}}(S,Bl_{Z}X)\cong Map_{dSch_{X}}(S,\pi_{0}(Bl_{Z}X))$ is a set in this case. However, if we don't know the fact that the derived blow up of quasi-smooth closed immersion is represented, then the space of Cartier divisors on a classical scheme $S$ is not easy to be seen as discrete.
$\textbf{Question :}$$\textbf{Are there more direct ways to see this space is discrete}$?
Remark: I tried to take a step back and consider the case when $X,Z,S$ are all classical schemes and $Z\stackrel{i}{\longrightarrow}X$ is regular closed immersion, in this case, the derived blow up itself is also a classical scheme actually by thm 4.1.5vi of the above paper, and pullback of the exceptional divisor along the morphism to this classical blow up leads to the effective divisors on $S$. And I can't help asking whether the virtual Cartier divisors on $S$ are 0-truncated and exactly the effective divisors on $S$?.
For any Cartier divisor $i_{D}:D\rightarrow S$ lying over $(Z,X)$, there is an associated point in the space of virtual Cartier divisors $Bl_{Z}X(S)$, that is, the Cartier divisor $i_{\pi_{0}(D)}: \pi_{0}(D)\rightarrow S$, and this is a classical scheme and in the case when $Z,X,S$ are classical, it is an effective divisor on $S$. So there is a set consisting of effective divisors on $S$ lying over $(Z,X)$ situated in the space of virtual Cartier divisors on $S$ and in bijection with the connected component of this space. However, this doesn't lead to the conclusion that each virtual Cartier divisor lies in the same component with some effective Cartier divisor, that is, there may not exist a path between them, so they are not equivalent as schemes in $dSch_{S\times_{X} Z}$. This is obvious, if we take $(Z,X)=(0,\mathbb{A}^{1})$ then if $S=Spec(C)\rightarrow \mathbb{A}^{1}$ is induced by some non-regular element in $C$, then $S\times_{\mathbb{A}^{1}} 0$ is not classical as the associated Koszul complex is not acylic. I think this reveals how meaningless the isomorphism of sets is, especially when they are of infinite cardinal. And the slogan $\textbf{the set of effective Cartier divisors is in bijection with the set of virtual Cartier divisors}$ seems not to be of much use.
