3
$\begingroup$

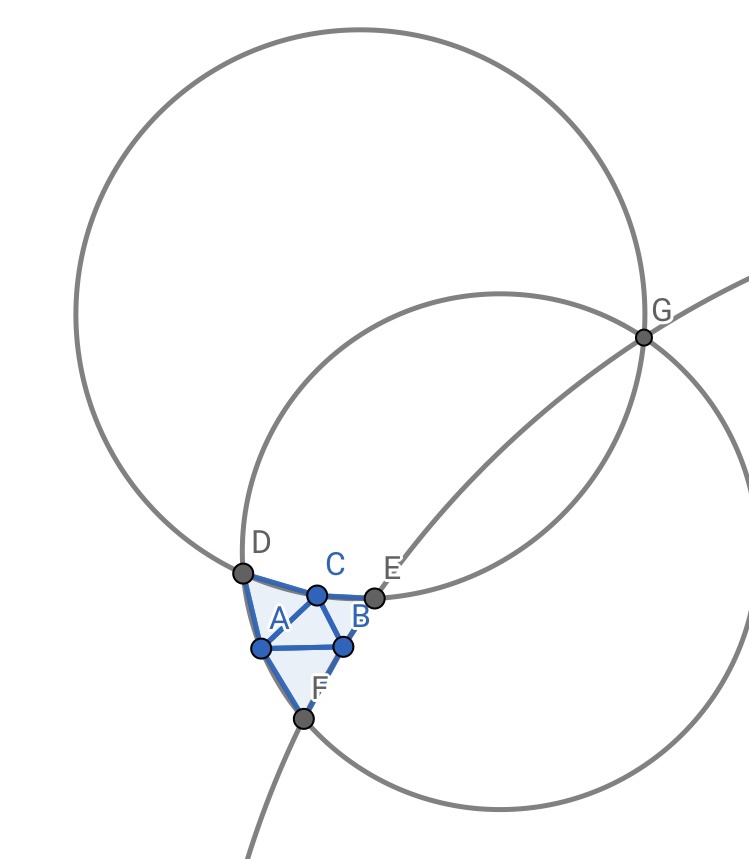
enter image description here

Let $A, B$, and $C$ be the vertices of a given triangle. Let $ACD, ABF$, and $BCE$ form equilateral triangles (internal or external). Then circles $ADF, BEF$, and $CDE$ are concurrent at point $G$.

Surely there must be some formal name for this point, right? What is it known as?

Improve this question
$\endgroup$
1

1 Answer 1

Reset to default
2
$\begingroup$

Using GeoGebra, I constructed the point for a triangle of sides $AB=13$, $BC=6$ and $CA=9$, and computed the (oriented) distance from it to the line $(BC)$, getting a value $kx\approx -13.209$.

Comparing with the list https://faculty.evansville.edu/ck6/encyclopedia/Search_6_9_13.html, I got a match as the point $X(1337)$, that is the "1st Wernau Point", whose definition matches yours. For references (Olympiad problems), see https://faculty.evansville.edu/ck6/encyclopedia/ETCPart2.html

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .