Motivated by an interest in the interplay between dynamical systems and elliptic curves also On a question of Mordell, I derived a dynamical system corresponding to the elliptic curve:
$ E_d: Y^2 = X^3 + B_d,d=|x+y| $
where $ B_d = -2(6d)^3 (d^3 + 4 \, \text{Sign}(z) \, k). $
The derived dynamical system is given by:
$ X_{n+1} = \lambda_n^2 - 2X_n $ $ Y_{n+1} = \lambda_n (X_n - X_{n+1}) - Y_n $ where $\lambda_n$ is a varying parameter.
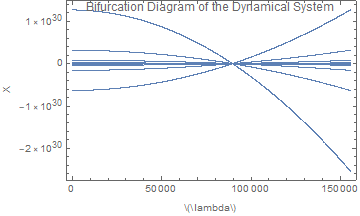
By iterating this dynamical system for various parameter values, I generated a bifurcation diagram exhibiting complex behavior as shown below.
Research Question:
Is there a correlation between the bifurcation points of this dynamical system and the integral points of the elliptic curve $E_d$? Specifically, can we establish a relationship between the dynamical system's behavior (e.g., periodicity, chaos) and the arithmetic properties of the elliptic curve (e.g., rank, torsion group)?
Note:The motivation behind this question is to discuss the analytic behavior of the equation $x^3+y^3+z^3=k$ in integers