Divide angles by coefficients relate to Fibonacci sequence

Question

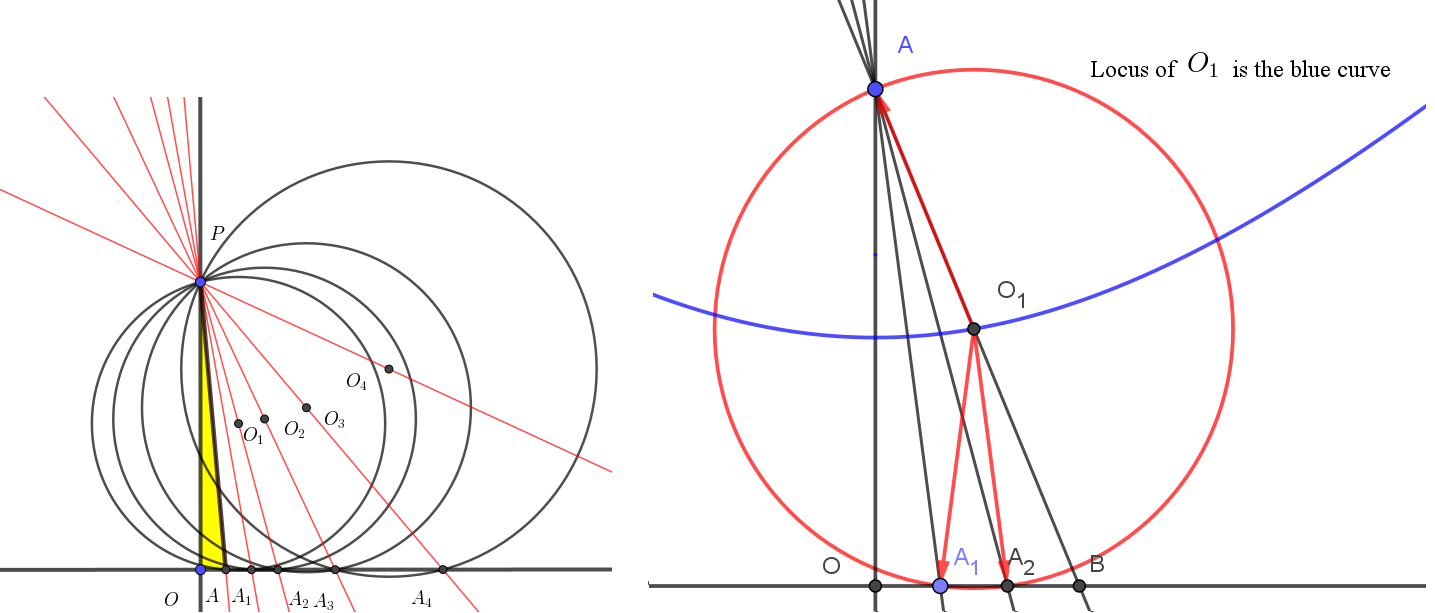
In the left Figure, consider a right triangle $OPA$ with $\angle {AOP} = 90^\circ$. Let $\ell$ be the reflection of $PO$ in $PA$ and $\ell$ meets $OA$ at $A_1$. Let $O_1$ be the center of the circle $(PAA_1)$, the line $PO_1$ meets $OA$ at $A_2$. Let $O_2$ be the center of the circle $(PA_1A_2)$, the line $PO_2$ meets $OA$ at $A_3$,.....Let $O_n$ be the center of the circle $(PA_{n-1}A_{n})$, the line $PO_n$ meets $OA$ at $A_{n+1}$ for $n=3, 4, 5,\ldots$. Denote $\angle {OPA} = \angle {APA_1} = \alpha_1$, $ \angle A_i PA_{i+1}=\alpha_{i+1}$ for $i=\overline{1,n}$
Easily to show that $\alpha_2=\alpha_1$ and $\alpha_{n+1}=\alpha_{n}+\alpha_{n-1}$ for $n=2,3,4,...$ this is Fibonacci sequence.
Now in Cartesian coordinates, let $P=(0,1)$, $O=(0,0)$, $A=(x,0)$. What is the locus equation of some circumcenters $O_1$, $O_2$, $O_3$...when we move $A$ on $Ox$? If we can find these locus equations, we can divide any angles by coefficients relate to Fibonacci sequence.
Example: In right Figure, let $AOB$ be a right triangle with $A(0,1)$, $O(0,0)$, $B(x,0)$. The locus of $O_1$ (blue curve) meets $AB$ at $O_1$, the circle $(O_1, O_1B)$ meets $OB$ at $A_1, A_2$ then $\angle OAA_1 = \angle A_1AA_2 = \angle A_2AB = \frac{\angle OAB}{3}$.

According to your conjecture $\alpha_i=\alpha_1F_i$ in particular diverges. But shouldn’t by definition $\alpha_i$ be an angle of some triangle, thus less than $\pi$? — Pietro Majer, Commented Jun 19 at 21:29
Haven't you defined $\alpha_i$ as an angle of the triangle with vertices $A_{i-1}$, $P$, $A_i$ ? Maybe you mean another definition ? — Pietro Majer, Commented Jun 20 at 12:18
As it is, what is true is $$\alpha_{n+1}=\alpha_n+\alpha_{n-1}\mod\pi$$ — Pietro Majer, Commented Jun 20 at 12:22
Standard notation is $90^\circ,$ not $90^o$ or $90^0$ or the like. I edited accordingly. — Michael Hardy, Commented Jun 20 at 14:22

Đào Thanh Oai · Accepted Answer · 2024-06-21 13:46:53Z

0

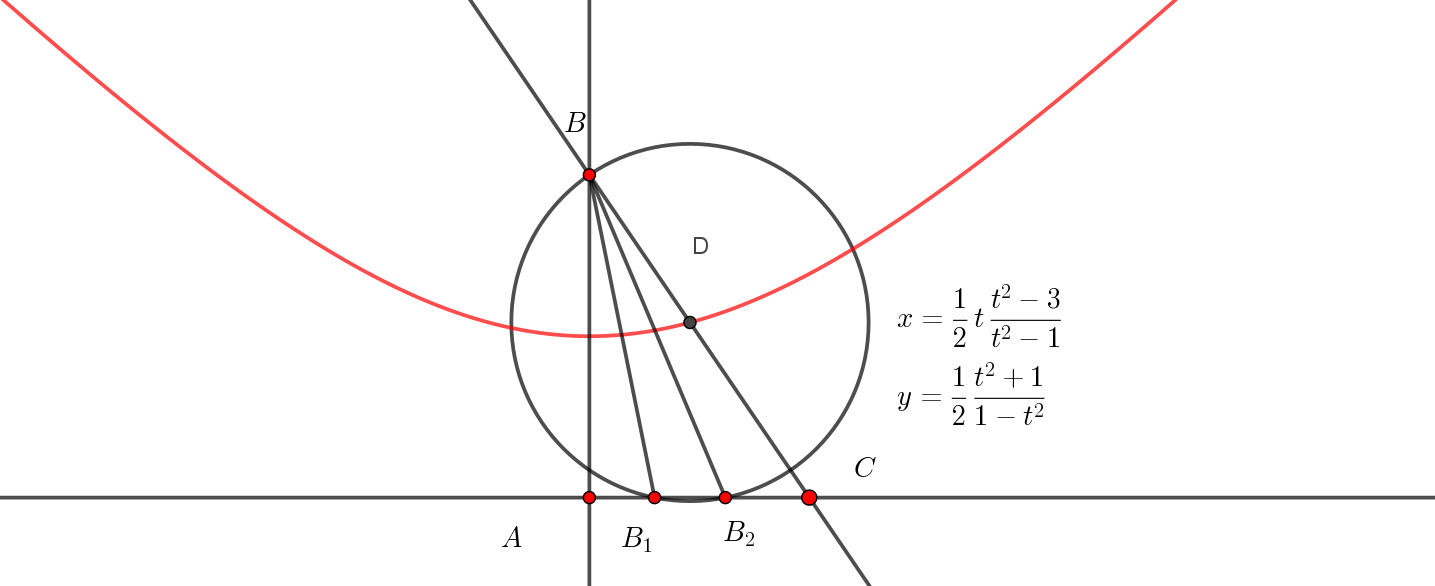
Locus equation of the point $O_1$, In Cartesian coordinates, as follows: $$x=\frac{1}{2}t\frac{t^2-3}{t^2-1}$$ $$y=\frac{1}{2}\frac{t^2+1}{1-t^2}$$ where $-1<t<1$ or the equation: $$x^2-y^2=\frac{2y^2-1}{2y+1}$$ where $y>0$.
Let $ABC$ be a triangle with $A=(0,0), B(0,1)$ and $C$ lie on $Ox$, let $BC$ meets the curve at $D$, the circle circle $(D, DB)$ meets $AC$ at $B_1, B_2$ then $$\angle ABB_1=\angle B_1BB_1 = \angle B_2BC = \frac{\angle ABC}{3} $$

answered Jun 21 at 7:05

Đào Thanh Oai

1,77611 silver badges37 bronze badges

Add a comment |

1 Answer 1