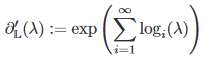This paper gives a derivation definition on log-atomic surreal numbers:
where the logarithm with lower index means iterated logarithm.
I think — I may be wrong — that $\omega$ is a log-atomic number. In that case, the derivation follows this formula.
But we know that the derivation of $\omega$ should be $1$, so, this long expansion should be in the limit equal to 1 (the sum should be zero). But it is not.
What's wrong here?
Note that the first iteration 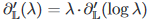 looks indeed as $1$ if $\omega$ is inserted for $\lambda$.
looks indeed as $1$ if $\omega$ is inserted for $\lambda$.