1
$\begingroup$

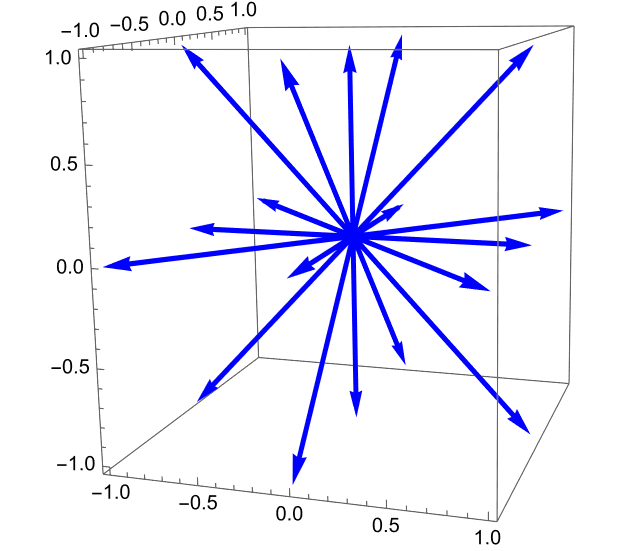
The root lattice of $\mathfrak{so}(7)$ is given by the following 18 roots:

$$ \left(\begin{array}{c}0\\0\\1\end{array}\right) , \left(\begin{array}{c}0\\0\\-1\end{array}\right) , \left(\begin{array}{c}1\\1\\0\end{array}\right) , \left(\begin{array}{c}-1\\1\\0\end{array}\right) , \left(\begin{array}{c}1\\-1\\0\end{array}\right) , \left(\begin{array}{c}-1\\-1\\0\end{array}\right) , \left(\begin{array}{c}1\\0\\0\end{array}\right) , \left(\begin{array}{c}-1\\0\\0\end{array}\right) , \left(\begin{array}{c}0\\1\\0\end{array}\right) , \left(\begin{array}{c}0\\-1\\0\end{array}\right) , \left(\begin{array}{c}1\\0\\1\end{array}\right) , \left(\begin{array}{c}-1\\0\\1\end{array}\right) , \left(\begin{array}{c}0\\1\\1\end{array}\right) , \left(\begin{array}{c}0\\-1\\1\end{array}\right) , \left(\begin{array}{c}1\\0\\-1\end{array}\right) , \left(\begin{array}{c}-1\\0\\-1\end{array}\right) , \left(\begin{array}{c}0\\1\\-1\end{array}\right) , \left(\begin{array}{c}0\\-1\\-1\end{array}\right) $$

Its root lattices looks like this:

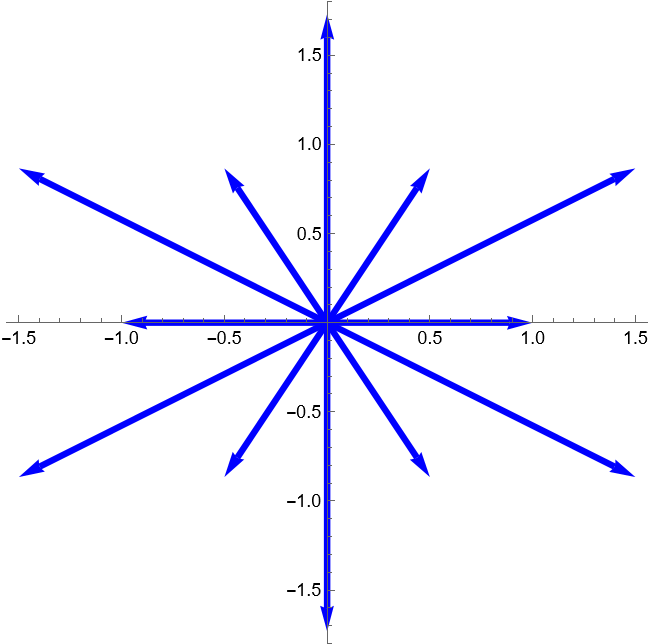
enter image description here

$\mathfrak{g}_2$ is a sub algebra of $\mathfrak{so}(7)$. So it seems naturally to ask, whether there is a relation between the two root lattices. The root lattice of $\mathfrak{g}_2$ is two-dimensional.

The roots are given by:

$$ \left(\begin{array}{c}0\\\sqrt{3}\end{array}\right) , \left(\begin{array}{c}0\\-\sqrt{3}\end{array}\right) , \left(\begin{array}{c}\frac{1}{2}\\\frac{\sqrt{3}}{2}\end{array}\right) , \left(\begin{array}{c}\frac{1}{2}\\-\frac{\sqrt{3}}{2}\end{array}\right) , \left(\begin{array}{c}-\frac{1}{2}\\\frac{\sqrt{3}}{2}\end{array}\right) , \left(\begin{array}{c}-\frac{1}{2}\\-\frac{\sqrt{3}}{2}\end{array}\right) , \left(\begin{array}{c}1\\0\end{array}\right) , \left(\begin{array}{c}-1\\0\end{array}\right) , \left(\begin{array}{c}\frac{3}{2}\\\frac{\sqrt{3}}{2}\end{array}\right) , \left(\begin{array}{c}\frac{3}{2}\\-\frac{\sqrt{3}}{2}\end{array}\right) , \left(\begin{array}{c}-\frac{3}{2}\\\frac{\sqrt{3}}{2}\end{array}\right) , \left(\begin{array}{c}-\frac{3}{2}\\-\frac{\sqrt{3}}{2}\end{array}\right) $$

and they look like:

enter image description here Is it possible to obtain some scaled version of this root lattice from a projection of the root lattice of $\mathfrak{so}(7)$ onto a particular plane?

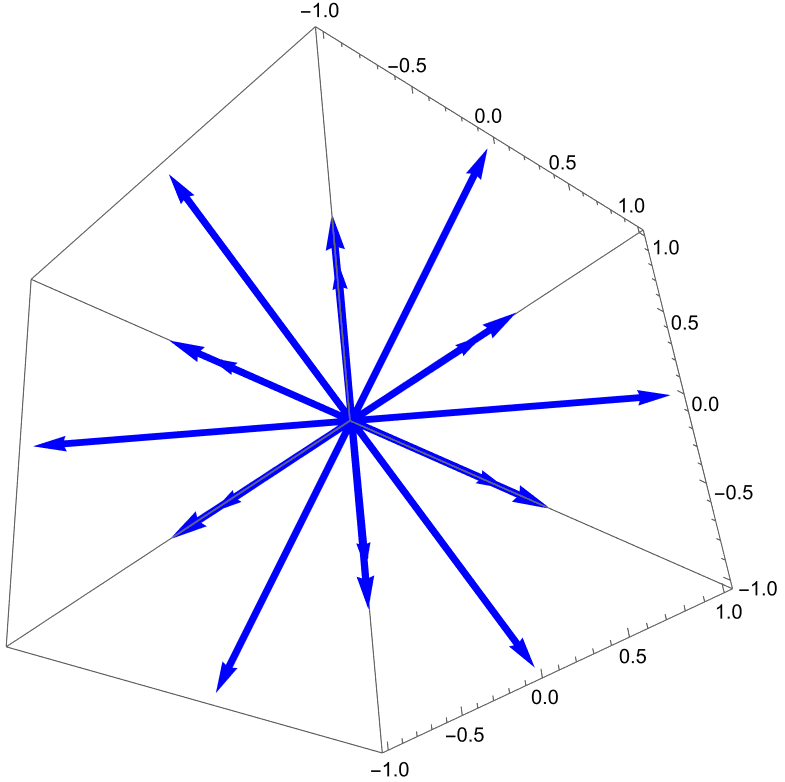
An experimental attempt lets me rotate the root lattice of $\mathfrak{so}(7)$ that the root structure is similar to the one of $\mathfrak{g}_2$ but the lengths of merging roots do not match:

enter image description here

Improve this question
$\endgroup$
1
  • 1
    $\begingroup$ Any Coxeter plane should work, for instance the plane spanned by $(1,-1,2)$ and $(0,1,-1)$. I wonder if the difference in root lengths in your last picture is because your viewer does not perform orthogonal projection. $\endgroup$
    – Grant B.
    Commented Apr 26 at 11:07

1 Answer 1

Reset to default
4
$\begingroup$

Let $\{\beta_1,\beta_2,\beta_3\}$ be the simple roots of type $B_3$, where $\beta_3$ is the short root (here, let $(\beta_1,\beta_1)=(\beta_2,\beta_2)=2$ and $(\beta_3,\beta_3)=1$). In your coordinates, $\beta_1=(1,-1,0)$, $\beta_2=(0,1,-1)$, and $\beta_3=(0,0,1)$. Then $$\big\{\beta_2=(0,1,-1),\frac13(\beta_1+2\beta_3)=\big(\frac13,-\frac13,\frac23\big)\big\}.$$ are the simple roots of type $G_2$ (as mentioned by Grant). Then span of $\beta_2$ and $\frac13(\beta_1+2\beta_3)$ is the orthogonal complement of $\beta_1-\beta_3=(1,-1,-1)$, so the relevant projection is $$p(x):=x-\frac13(\beta_1-\beta_3,x)(\beta_1-\beta_3).$$ Under this projection: $$\begin{align*} p(\beta_1)&=\beta_1-\frac23(\beta_1-\beta_3)=\frac13(\beta_1+2\beta_3)\\ p(\beta_2)&=\beta_2\\ p(\beta_3)&=\frac13(\beta_1+2\beta_3). \end{align*}$$

P.S. The linear combinations $\{\beta_2,\frac13(\beta_1+2\beta_3)\}$ can be computed as follows. Let $\{\alpha_1,\alpha_2,\alpha_3,\alpha_4\}$ be the simple roots of type $D_4$, where $\alpha_2$ is the vertex in the middle. Then $B_3$ is a folding under the involution swapping $\alpha_3$ and $\alpha_4$, so the simple roots are $\{\beta_1:=\alpha_1,\beta_2:=\alpha_2,\beta_3:=\frac12(\alpha_1+\alpha_3+\alpha_4)\}$. Similarly, $G_2$ is the folding under the $S_3$-action permuting $\alpha_1$, $\alpha_3$, and $\alpha_4$, so the simple roots are $\{\alpha_2,\frac13(\alpha_1+\alpha_3+\alpha_4)\}$. Now the simple roots of $G_2$ are obtained from the simple roots of $B_3$ as follows: $$\begin{align*} \alpha_2&=\beta_2\\ \frac13(\alpha_1+\alpha_3+\alpha_4)&=\frac13\alpha_1+\frac13(\alpha_3+\alpha_4)\\ &=\frac13\beta_1+\frac23\beta_3. \end{align*}$$

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .