Given a $CW$-complex $X$ and a closed surface group $\pi_1(\Sigma,v) < \pi_1(X,x)$, there exists a map $\phi: (\Sigma,v) \to (X,x)$ such that the image of the fundamental group is this subgroup. Take a 1-vertex triangulation of $\Sigma$ with vertex $v$, map the vertex $v$ to the basepoint $x\in X$, then each edge $e$ represents a closed loop and hence an element of $\pi_1(\Sigma)$. Thus we may map this element into $X$ uniquely up to homotopy rel basepoint. The boundary of each triangle of $\Sigma$ is homotopically trivial in $\Sigma$, so its image in $X$ is homotopically trivial, and hence can be filled in with a disk. More generally, this shows that for any group $G <\pi_1(X)$ and two-complex $C$ with $\pi_1(C)=G$, there is a map $C\to X$ inducing the map $G\to \pi_1(X)$ on the level of fundamental group.
If $X$ is a 3-manifold, then it is shown by Schoen and Yau that $\phi:\Sigma\to X$ is homotopic to a minimal area immersion in its homotopy class. However, this proof is overkill (the proof that an area minimizing surface is immersed is due to Osserman and Gulliver). The essential point is a technique of Whitney which classifies maps of $n$-manifolds into $2n-1$ Euclidean space, showing that they may be approximated by immersions. Bing and Papakyriokopoulos applied this technique to maps of disks and spheres into 3-manifolds, and this was generalized by Gabai and Oertel to maps of surfaces. The proof of the main theorem of Oertel explains this argument. (And also provides the figures below.)
Whitney classified singularities of maps, which in the case of maps of surfaces to 3-manifolds looks like:
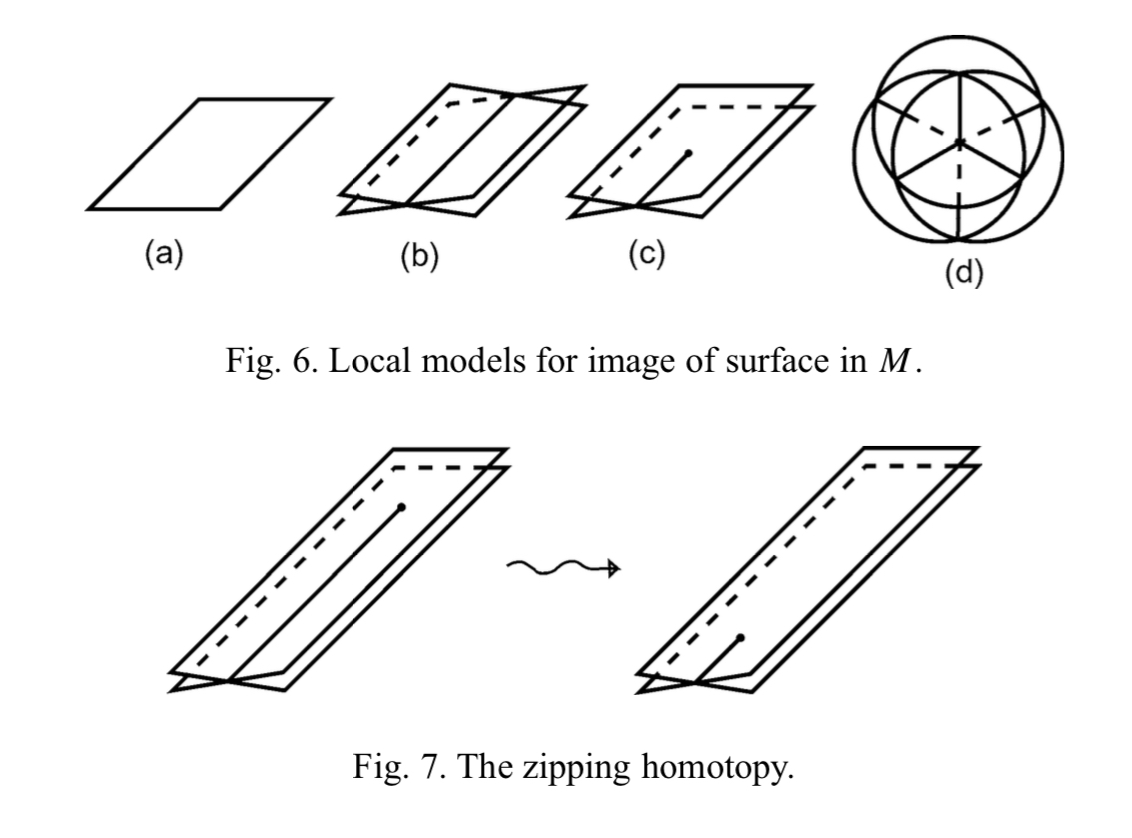 The singular set includes arcs and curves of double points, triple points, and branch points.
The singular set includes arcs and curves of double points, triple points, and branch points.
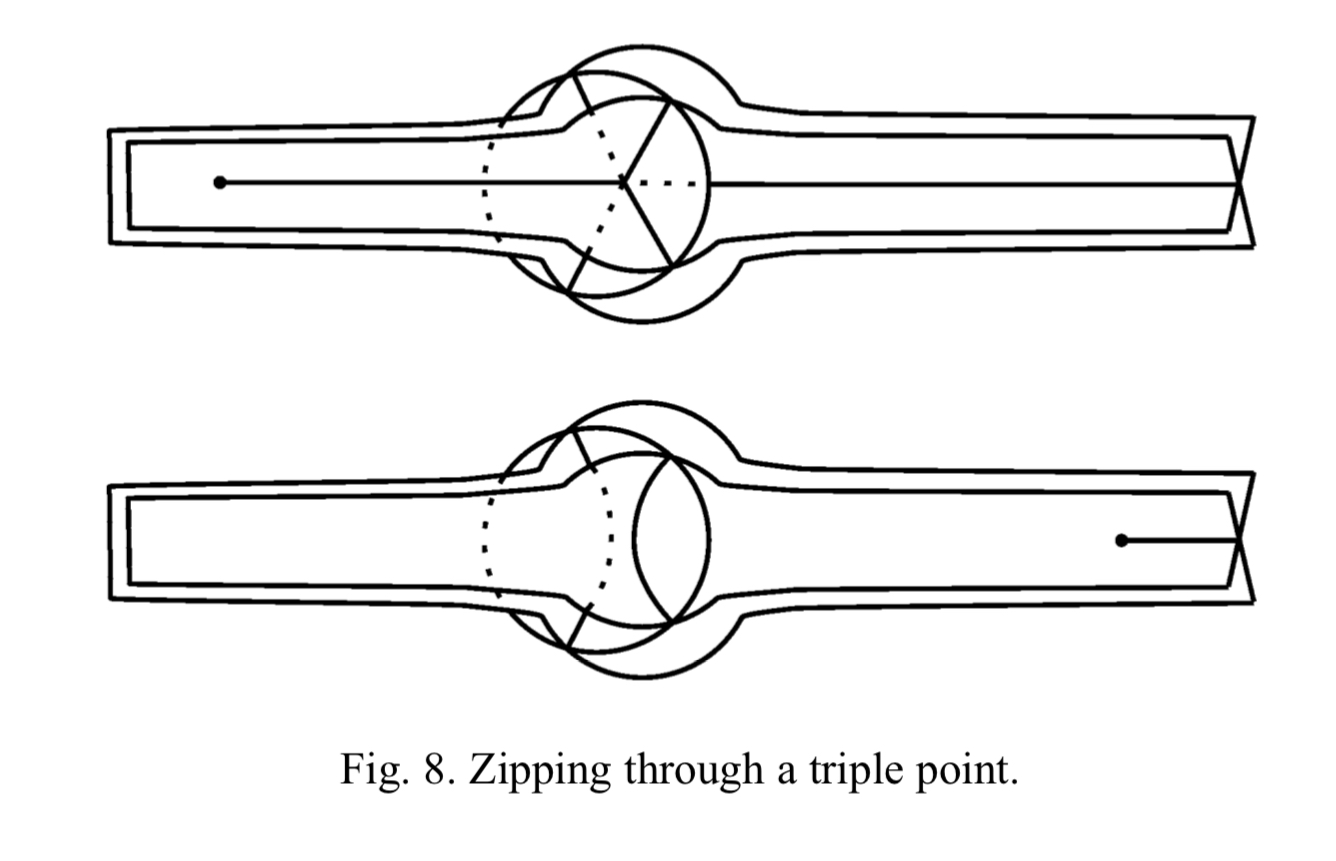
To get rid of branched points, and hence create an immersion, one starts at a branched point and “zips” to decrease the length of the singular arc of double points as in Figure 7. One may push through triple points (decreasing the number of triple points) as in Figure 8 until another branch point is reached as in Figure 10 (this is the same argument employed by Papakyriokopoulos and Bing; it may go back to Dehn but I didn’t check).
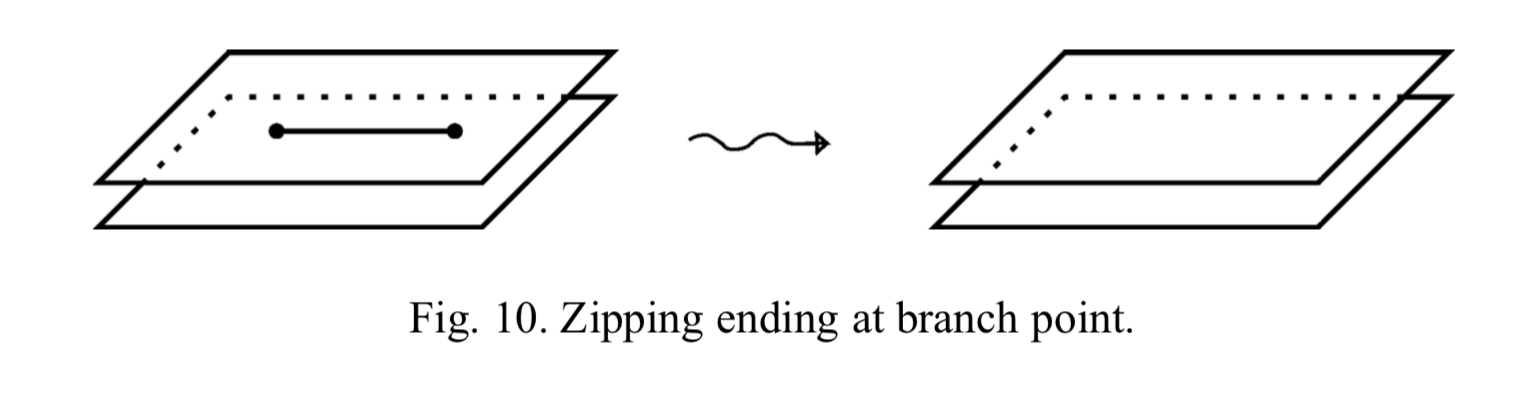
There is another local picture (not shown) which looks like a crosscap; in this case the surface is not $\pi_1$-injective since the core of the Mobius strip is homotopically trivial. In the $\pi_1$-injective case (or more generally simple-loop injective), the immersed arc (shown in Figure 10 ) may be surgered away to give a disconnected surface with a sphere component, and the sphere component removed. After finitely many such operations, the branched points are removed and the map is an immersion.



