Mittag-Leffler expansions converging to bounded function

Question

Is it true that

$$\lim_{N\to\infty}\left\langle\sum_{n=-N^2}^{N^2}\frac1{(Nx-n)^2}\right\rangle_N=\pi^2$$

for some suitable definition of "minima smoothing" such as $\langle f(x)\rangle_N\overset{?}{=}\inf_{|y-x|<\frac2N} f(y)$?

Here is $\sum_{n=-N^2}^{N^2}1/(Nx-n)^2$ for $N=5$ and $N=9$, with the green line at $\pi^2$:

,

---

Mittag-Leffler expansions ("pole expansions") are ubiquitous for functions with countably many poles.
Is there literature on writing bounded functions as "pathological" sums with poles eventually forming dense subset of $\mathbb R$

$$\lim_{N\to∞}\frac1{N^2}\sum_{i\in[-N,-N+\frac1N,\ldots,N-\frac1N,N]}\frac{a_{N,i}}{(x-i)^2}$$

Perhaps it is done for complex functions with $S_N=\bigcup_{m=-N^2}^{N^2}\bigcup_{n=-N^2}^{N^2}\unicode{123}\frac{n+mi}N\unicode{125}$ as

$$\lim_{N\to∞}\frac1{N^4}\sum_{i\in S_N}\frac{a_i}{|z-i|}$$?

Answer 1

$\newcommand{\ts}{\tilde s}$Yes, this is true.

Indeed, take any real $c\ge1/2$ and let \begin{equation} s_n(y):=\sum_{-n^2\le k\le n^2}\frac1{(ny-k)^2} \end{equation} and \begin{equation} \ts_n(x):=\inf_{|y-x|<c/n}s_n(y). \end{equation}

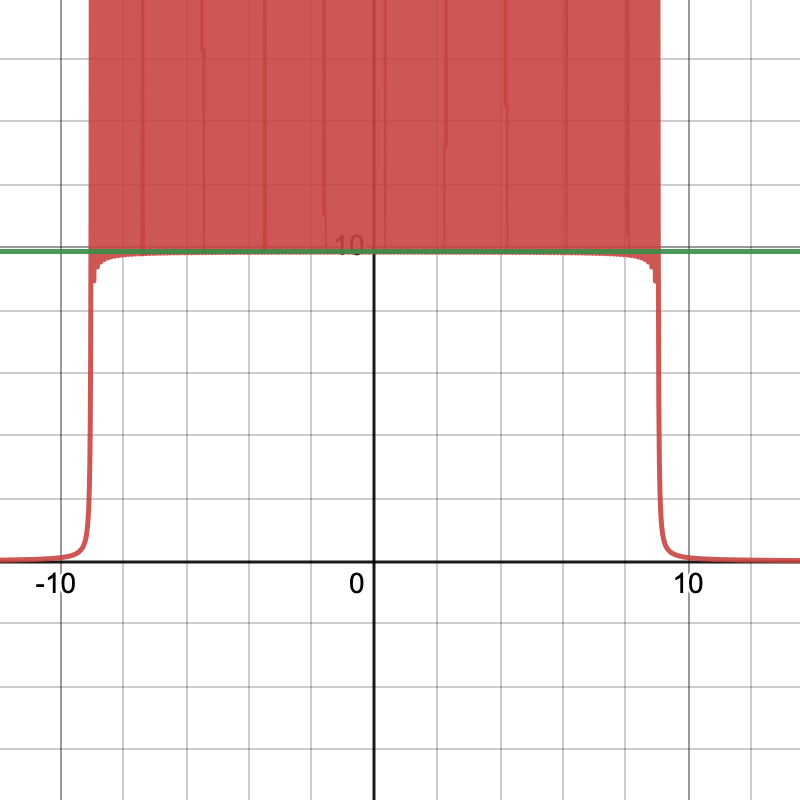
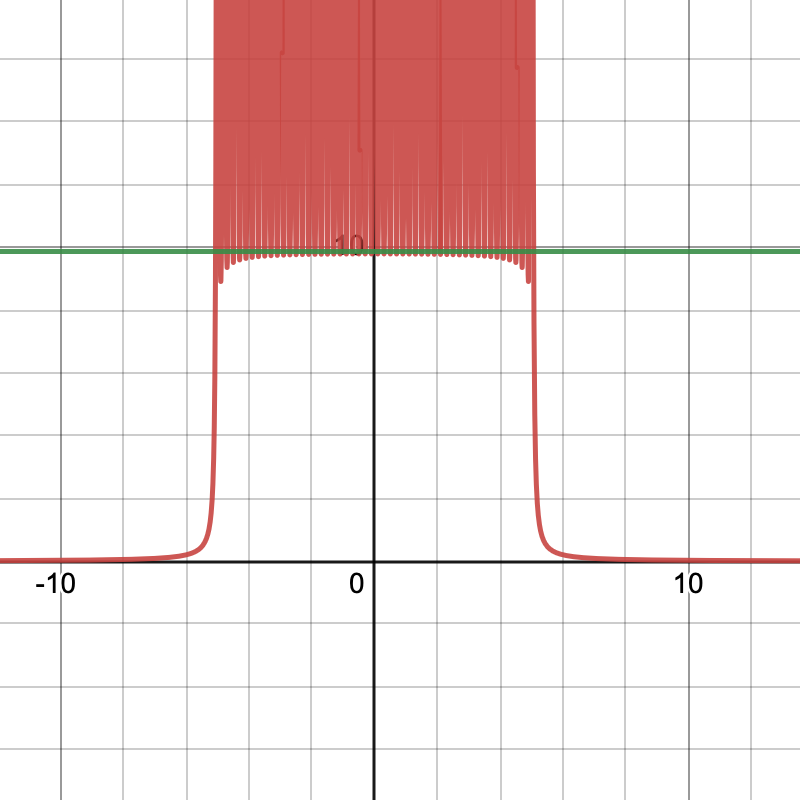
Proposition 1: $\ts_n(x)\to\pi^2$ (as $n\to\infty$) uniformly over all real $x$ such that $n^2-n|x|\to\infty$.

The condition $n^2-n|x|\to\infty$ can be rewritten as $|x|\le n-\dfrac{\rho_n}n$ for some $\rho_n$ going (however slowly) to $\infty$. (This seems to be in agreement with your pictures.)

Proof of Proposition 1: Suppose that $n$ is large enough so that $n^2-n|x|>c+1$. Take any real $y$ such that $|y-x|<c/n$, so that $n|y|<n^2-1$. If $n|y|$ is an integer, then $s_n(y)=\infty$, so that excluding such values of $y$ does not affect the value of $\ts_n(x)$. Now, excluding such values of $y$ indeed and letting \begin{equation} m:=\lfloor ny\rfloor, \end{equation} we get \begin{equation} s_n(y)=s_{n,m}(u):=\sum_{-n^2+m\le j\le n^2+m}\frac1{(u+j+1/2)^2}, \end{equation} where \begin{equation} u:=ny-(m+1/2)\in(-1/2,1/2). \end{equation}

For $q=1,2,\dots,\infty$, let \begin{equation} t_q(u):=\sum_{-q-1< j<q}\frac1{(u+j+1/2)^2}. \end{equation} Then $t_q$ is an even convex function on the interval $(-1/2,1/2)$, so that \begin{equation} t_q\ge t_q(0) \end{equation} and, moreover, \begin{equation} t_\infty(0)=\pi^2. \end{equation} Furthermore, by the convexity of $\frac1{z^2}$ in $z>0$, \begin{equation} \pi^2-t_q(0)=t_\infty(0)-t_q(0)=2\sum_{j\ge q}\frac1{(j+1/2)^2} <2\int_q^\infty\frac{dz}{z^2}=\frac2q. \end{equation}

So, for $y$ as described above, \begin{equation} s_n(y)=s_{n,m}(u)\ge t_{n^2-|m|}(u)\ge t_{n^2-|m|}(0) >\pi^2-\frac2{n^2-|m|}\to\pi^2, \end{equation} since $n^2-n|x|\to\infty$, $|m|\le n|y|+1$, and $|y-x|<c/n$, so that $n^2-|m|\ge n^2-n|x|-(c+1)\to\infty$, so that \begin{equation} \ts_n(x)=\inf_{|y-x|<c/n}s_n(y)\ge \pi^2-o(1). \end{equation}

On the other hand, letting $l:=\lfloor nx\rfloor$, we have $|\frac{l+1/2}n-x|\le\frac1{2n}\le\frac cn$, so that \begin{equation} \ts_n(x)=\inf_{|y-x|<c/n}s_n(y)\le s_n\Big(\frac{l+1/2}n\Big) =s_{n,l}(0)<t_\infty(0)=\pi^2. \end{equation}

Proposition 1 follows immediately from the latter two displays. $\quad\Box$