Is it true that
$$\lim_{N\to\infty}\left\langle\sum_{n=-N^2}^{N^2}\frac1{(Nx-n)^2}\right\rangle_N=\pi^2$$
for some suitable definition of "minima smoothing" such as $\langle f(x)\rangle_N\overset{?}{=}\inf_{|y-x|<\frac2N} f(y)$?
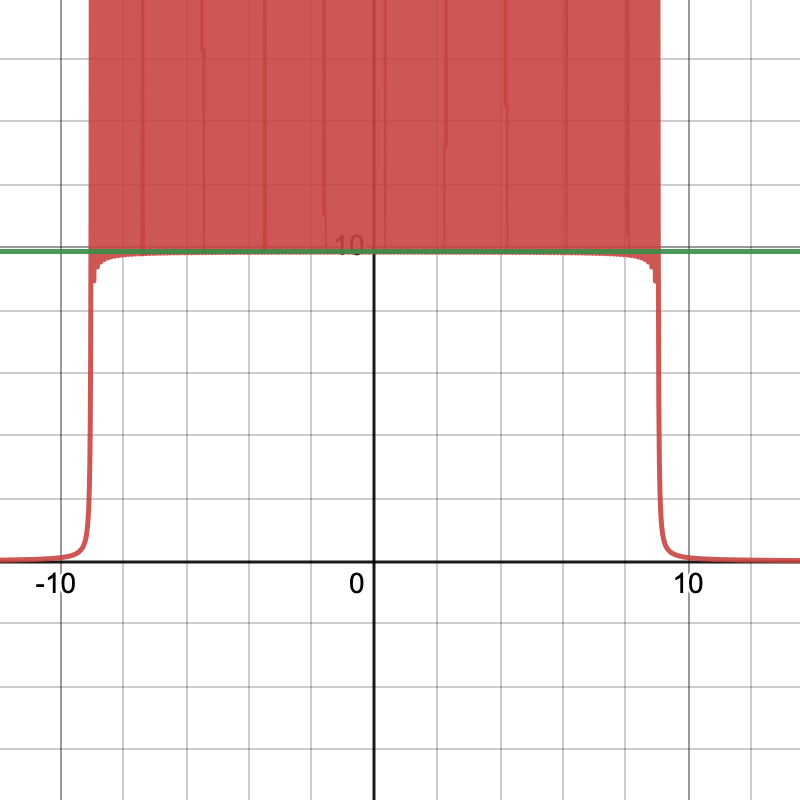
Here is $\sum_{n=-N^2}^{N^2}1/(Nx-n)^2$ for $N=5$ and $N=9$, with the green line at $\pi^2$:
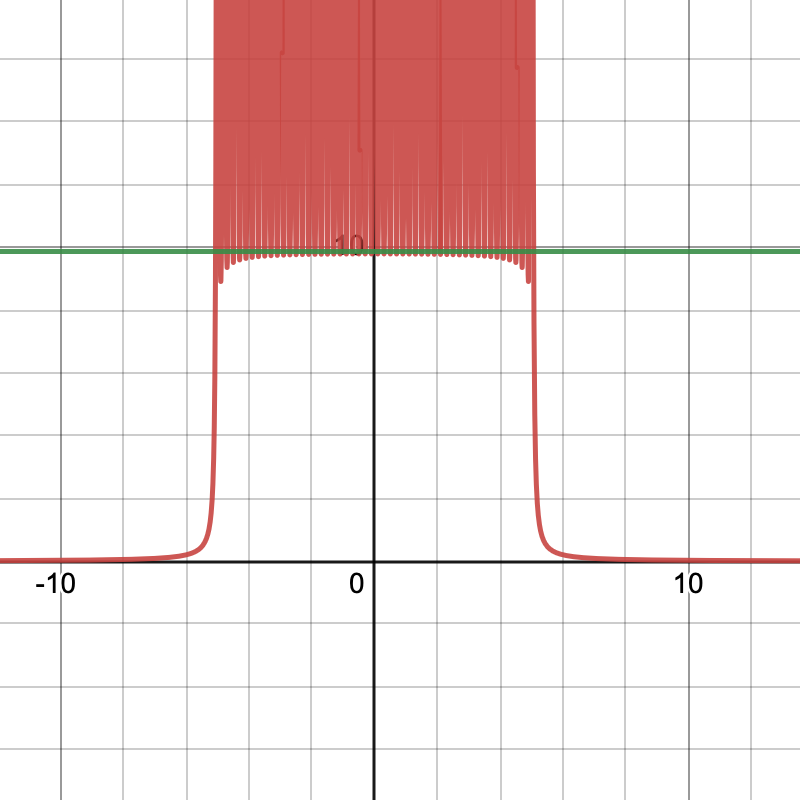 ,
,
Mittag-Leffler expansions ("pole expansions") are ubiquitous for functions with countably many poles.
Is there literature on writing bounded functions as "pathological" sums with poles eventually forming dense setsubset of $\mathbb R$
$$\lim_{N\to∞}\frac1{N^2}\sum_{i\in[-N,-N+\frac1N,\ldots,N-\frac1N,N]}\frac{a_{N,i}}{(x-i)^2}$$
Perhaps it is done for complex functions with $S_N=\bigcup_{m=-N^2}^{N^2}\bigcup_{n=-N^2}^{N^2}\unicode{123}\frac{n+mi}N\unicode{125}$ as
$$\lim_{N\to∞}\frac1{N^4}\sum_{i\in S_N}\frac{a_i}{|z|}$$$$\lim_{N\to∞}\frac1{N^4}\sum_{i\in S_N}\frac{a_i}{|z-i|}$$?
