I am trying to understand Klyachko's following description of the cohomology groups of locally free (hopefully more generally of reflexive) sheaves on toric varieties.
Whereas detailed literature regarding the constructiob of Klychko's filtration is available (e.g. Perling) I was not able to find references discussing the part on cohomology. I have the following questions:
- Is anyone aware of references that discuss and prove the part on cohomology?
- Does theorem 3.1 also work for reflexive sheaves?
- Consider $X=\mathbb{P}^n$ and the cotagnet bundle $\Omega_{\mathbb{P}^n}$. For this case, we know that the filtrations are given by
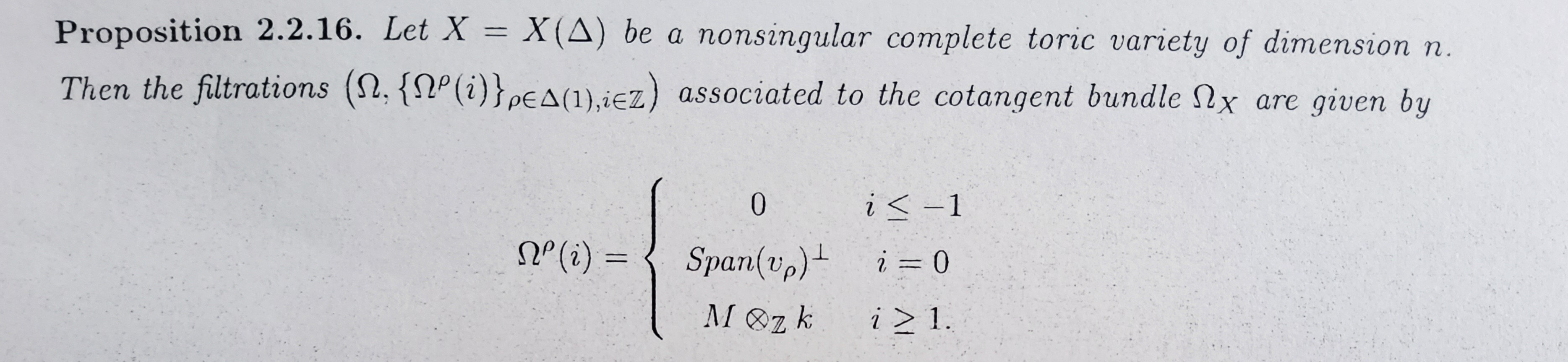In particular, consider $n=2$ and the complete fan with rays $\rho_0 =(1,0), \rho_1 = (0,1), \rho_2 =(-1,-1)$ with the maximal cones $\sigma_0 =Cone(\rho_0,\rho_1), \sigma_1 =Cone(\rho_1,\rho_2), \sigma_2 =Cone(\rho_2,\rho_0)$. How do we compute the space $E_{\sigma}(\chi)$ and the complex $C^{*}(E,\chi)$ for a given cone $\sigma$ and a given character $\chi$?
