In the paper Canonical heights on varieties with morphisms by Joseph H. Silverman, in page 184 (which is page 23 in the PDF) Silverman uses Lang's Fundamentals of Diophantine Geometry to show that
$$|h_{{V,\eta}_V}(Q_V)-\deg Q^\ast\eta|\leq c$$

Where $V$ is a generic fiber of the family $\pi: T\to \mathcal{V}$ is a family of abelian varieties as defined in page 177, section 3 (page 16 in the PDF).
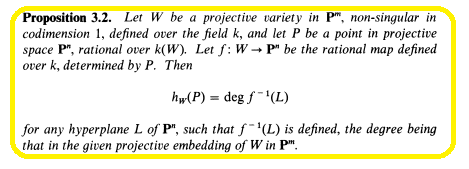
The given reference is:
Which is an equality and not an inequality as in the first image. My question is where did the constant in the inequality $|h_{{V,\eta}_V}(Q_V)-\deg Q^\ast\eta|\leq c$ came from. My guess is that we can think on $\eta_V$ as a formal sum of hyper surfaces in $\Bbb{P}^n$, and since the Weil height has the property: $h_{D+E} = h_D + h_E + O(1)$, that is why the constant $c$ appears.
I'm a bit confused.
Thank in advance.