4
$\begingroup$

Let $S$ be a smooth closed connected embedded hypersurface in $\mathbb R^n$.

Is it true that $S$ is convex, i.e. is a boundary of a convex set, if and only if any section of $S$ by a hyperplane is connected?

If this is the case and is (surely) known I would appreciate the reference.

I have a sort of heuristic argument for $n=3$:

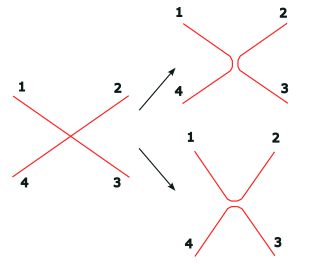
Suppose $p\in S$ is a point with strictly negative curvature, so the surface near $p$ qualitatively looks like the graph of $X^2-Y^2$ around $0$ (up to the second order). Consider the tangent hyperplane $H_p$ at $p$. If necessary, perturb $p$ slightly to make sure that $H_p\setminus\{p\}$ intersects $S$ transversally (i.e. $H_p\cap S$ is singular only at $ p$). Now if we slightly parallel tranport $H_p$ up and down, the corresponding intersections $H^+_p\cap S$ and $H^-_p\cap S$ will look near $p$ like this:

enter image description here

with the rest of the picture being topologically unaltered. Case by case analysis suggests that $H^-_p\cap S$ and $H^+_p\cap S$ cannot both be conneted: indeed, the strings can be connected 1-4;2-3 or 1-2;4-3 (1-3;2-4 would cause intersections other than $p$).

Improve this question
$\endgroup$
3
  • $\begingroup$ You need to assume that $n\ge3$, right? $\endgroup$
    – Iosif Pinelis
    Commented Jan 24 at 15:55
  • $\begingroup$ @IosifPinelis yes, thank you! or otherwise reformulate as "has the same number of components as the boundary of $n-1$-ball" to incorporate $n=1,2$ case $\endgroup$
    – Dmitrii Korshunov
    Commented Jan 24 at 16:54
  • 1
    $\begingroup$ This question reminds the following: Suppose $S$ is a convex surface in $\mathbb{R}^3$ such that intersection of $S$ with its copy is either empty or connected. Then $S$ is a sphere or cylinder with two spherical caps. Unfortunately I cannot find a reference to this statement, if I remener right it was proved by Pogorelov. $\endgroup$
    – Anton Petrunin
    Commented Jan 28 at 22:13

1 Answer 1

Reset to default
3
$\begingroup$

Suppose $n\geqslant 2$. Let $S$ be the boundary of $\tfrac12$-neighborhood of the unit $n$-sphere in $\mathbb{R}^{2{\cdot}n+1}$. (Note that $S$ is homeomorphic to $\mathbb{S}^n\times\mathbb{S}^n$.)

Evidently $S$ is not convex. Let us show that the intersection of $S$ with any hyperplane $\Pi$ is connected or empty. Assume the contrary; without loss of generality, we can assume that $\Pi$ is a level surface of a linear function $\ell\colon\mathbb{R}^{2{\cdot}n+1}\to\mathbb{R}$ such that $\ell$ is a Morse function on $S$. Note that $\ell|_S$ has 4 critical points with indices $0$, $n$, $n$, and $2{\cdot} n$. Observe that passing thru intermediate critical values does not breake the connectedness of the level set — a contradiction.

Improve this answer
$\endgroup$
2
  • 1
    $\begingroup$ thank you, this is cool! just a little elaboration for those (like me) who didn't see it right away: 1) if a hyperplane section is disconnected, then the sections by all nearby hyperplanes are also disconnected -- hence it is enough to prove for Morse linear functions. 2) once we pass a critical value of index less than dimension of the levelset ($\le 2n-2$ in our case) the contentedness doesn't change, e.g. because $H_{d-1}(\partial M^d)$ is not affected by gluing in a $d-2$-dimensional cell. $\endgroup$
    – Dmitrii Korshunov
    Commented Jan 29 at 13:54
  • $\begingroup$ this is funny how it's actually true for $n=1$ in light of the characterization of closed subsets mathoverflow.net/questions/28967/… because an (at least reasonable) open set in $\mathbb R^2$ is contractible iff its boundary is connected $\endgroup$
    – Dmitrii Korshunov
    Commented Jan 29 at 13:58

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .