Kepler conjecture: Are there only two most efficient packings or could there be more than two?

Question

Today I attended a talk by Terence Tao, attended by (I'm guessing) probably at least a couple of thousand people, in which among other things he said it had been proved that no packing of spheres in three dimensions is more efficient that cubic close packing and hexagonal close packing, which are equally efficient.

My question is whether it has also been proved that no other packing is equally efficient to those two. I.e. they are the only ones that attain the greatest efficiency.

Postcript: It is being pointed out in comments that in addition to cubic close packing and hexagonal close packing, there are mixtures of the two, in which some regions of space of spheres packed "cubically" and others "hexagonally". So the question must be taken to be: do mixtures of those two exhaust the possibilities, or are there others? Has it been proved that there are no others?

Postpostscript: All right, I will be explicit about something I would have thought was obvious: Construe the question as asking whether there are any other triply periodic packings other than those two that are just as efficient as those two?

Answer 1

The short answer is that classifying all maximum-density three-dimensional sphere packings, even the periodic ones, remains an open problem.

It will help to introduce some terminology. In a lattice packing, the centers of the spheres form a discrete subgroup of $\mathbb{R}^3$. A periodic packing is a finite union of translates of a lattice packing. As noted by other respondents, we can form uncountably many distinct maximum-density sphere packings in $\mathbb{R}^3$ by stacking hexagonally packed layers on top of each other; at each layer, we have two choices, so such packings, which are called Barlow packings, are indexed by bi-infinite sequences on three letters such that consecutive letters are distinct.

The title question asks if it is possible that there are just two maximum-density packings. I cannot think of any interpretation of this question under which the answer is yes. If we restrict to maximum-density lattice packings, then the face-centered cubic packing is the only lattice packing (the hexagonal close packing is periodic but it is not a lattice packing). If we require only that the packing be periodic, then there are infinitely many periodic Barlow packings (though of course, not every Barlow packing is periodic).

Again, as others have noted, if we allow non-periodic packings, then there are all kinds of packings that can achieve maximum density. But we could ask, is every maximum-density periodic packing a Barlow packing? This type of question is potentially tractable; for example, Viazovska's work actually shows that $E_8$ is the unique periodic packing in $\mathbb{R}^8$ of maximum density. However, as far as I can tell, even this question remains open in three dimensions. A standard reference is Conway and Sloane, What are all the best sphere packings in low dimensions?, Discrete Comput. Geom. 13 (1995), 383–403. Among other things, they propose a definition of a "tight" packing and show that, subject to two unproved postulates, the Barlow packings are the only tight packings. At the time of Conway and Sloane's paper, of course, the Kepler conjecture was still open, but when Hales announced his proof in An overview of the Kepler conjecture, he pointed out that Conway and Sloane's problem of characterizing the tight packings in $\mathbb{R}^3$ was still open.

Answer 2

I am posting this as a community wiki post because I am just reiterating things said in the comments, but I think you are missing some basic points and so wanted to highlight those.

You should read the Wikipedia article Close-packing of equal spheres.

In particular, you should look at this image from that Wikipedia page: 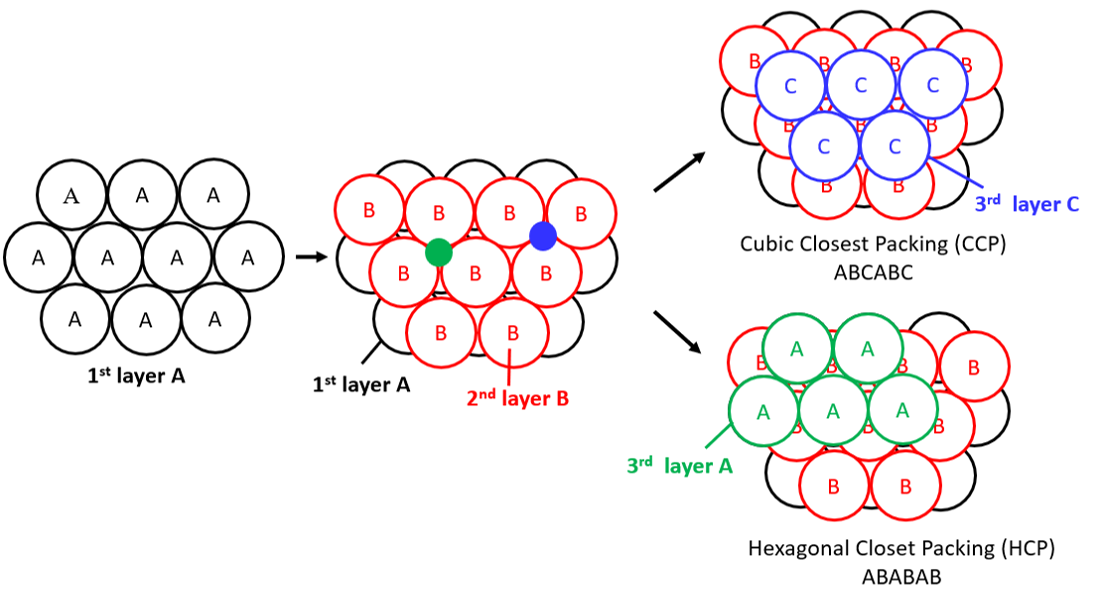It explains how to iteratively create a close packing of spheres (one attaining the maximum density) in layers. In the first step, we follow the "A" pattern, in the second step, we follow the "B" pattern, and then in the 3rd step we have a choice of either "A" or "C" pattern. We can continue in this way as long as at each step we choose a different letter than the one we are at. So different sequences of A's, B's, C's give different packings.

The cubic close packing corresponds to the pattern ABC ABC ABC ..., while the hexagonal close packing corresponds to the pattern AB AB AB .... Taking some other pattern, but which still is periodic, like say ABC AB ABC AB ..., will give another close packing of spheres which is not either of those two, but which is still "triply periodic" in the sense of your postpostscript. So the literal answer to the question in your postpostscript is: no, there are other packings beyond those two which are triply periodic and just as efficient.

EDIT: Packings of these kind are called Barlow packings. As suggested in the comments, perhaps your question ought to be whether all periodic packings of maximum density are Barlow packings. I am fairly certain this must be an open question. Again, referencing something brought up in the comments, in Kuperberg's "Notions of denseness", he says "It seems likely that a sphere packing is weakly recurrent among dense packings if and only if it is Barlow, but not all Barlow packings are uniformly recurrent." Here "weakly recurrent" and "uniformly recurrent" are technical notions having to deal with the issue of asymptotically-zero-density deformations, but morally they should be similar to periodic.

On the other hand, if maybe your interest is instead to single out the cubic close packing and hexagonal close packing among all maximally dense sphere packings, then you may be interested in this quote from "Recent Progress in Sphere Packing" by Conway, Goodman-Strauss, and Sloane: "Only two of these [Barlow packings] are 'uniform,' however, in the sense that there is a symmetry of the packing taking any one sphere to any other." So possibly that could be a characterization: the CCP and HCP are the only maximally dense sphere packings where the symmetry group acts transitively on the spheres. I am not sure if that is known.