4
$\begingroup$

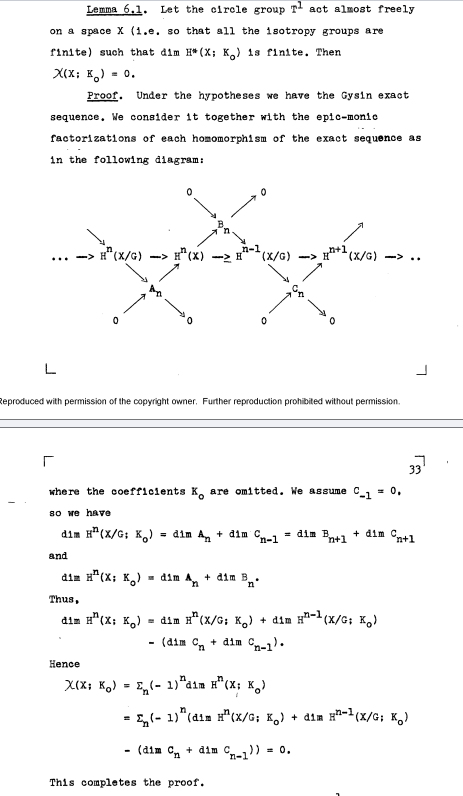
There is a claim in the following thesis regarding the exact sequence of Gysin. Shouldn't the spherical bundle $\mathbb{S}^1 \rightarrow X \rightarrow X/\mathbb{S}^1$ be orientable for the Gysin exact sequence?

I read in some sources that the spherical bundle $\mathbb{S}^1 \rightarrow X \rightarrow X/\mathbb{S}^1$ is orientable for free action of $\mathbb{S}^1$ on $X$, but I could not find a complete reference.

Under what conditions, $\mathbb{S}^1 \rightarrow X \rightarrow X/\mathbb{S}^1$ is orientable fiber bundle. Is it enough to be almost free?

enter image description here

Improve this question
$\endgroup$

1 Answer 1

Reset to default
3
$\begingroup$

See Bredon’s Sheaf Theory Chapter IV the intro to Section 13. The orbit map of a circle action is always orientable.

Improve this answer
$\endgroup$
3
  • $\begingroup$ If the orbit map of a circle action is always orientable, then why does the action need to be almost free? $\endgroup$
    – Mehmet Onat
    Commented Dec 14, 2023 at 8:38
  • $\begingroup$ The statement of the Lemma says why that hypothesis is being imposed — the isotropy groups are all finite. $\endgroup$
    – David Snyder
    Commented Dec 15, 2023 at 14:53
  • 1
    $\begingroup$ I did'nt understand your comment. As far as I can see, this hypothesis is not used in the proof. I thought this hypothesis was necessary for the Gysin exact sequence. I thought this was related to the orientability of the spherical fiber bundle. $\endgroup$
    – Mehmet Onat
    Commented Dec 15, 2023 at 16:20

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .