What can we say about the reciprocal of a reduced regular continued fraction?

Question

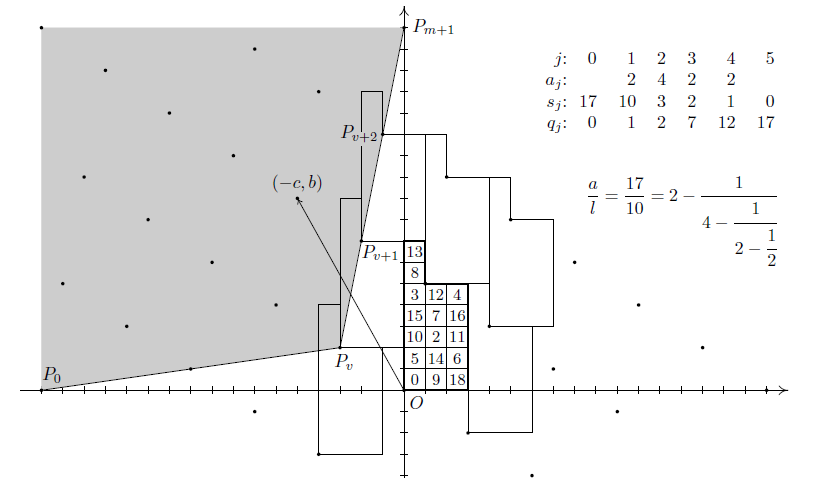
For positive integers $a>b>0$, we can represent $a/b$ uniquely as $$\frac{a}{b}=a_1-\cfrac{1}{a_2-\cfrac{1}{\cdots-\cfrac{1}{a_n}}}=:[a_1,\dots,a_n]^{-}$$ with $a_i\geq 2$, and this is called the reduced regular (or Hirzebruch-Jung) continued fraction.

For ordinary continued fraction $[a_1,\dots,a_n]^+:= \frac{a}{b}=a_1+\cfrac{1}{a_2+\cfrac{1}{\cdots+\cfrac{1}{a_n}}}$, it is known that $1/[a_1,\dots,a_n]^+=[0,a_1,\dots,a_n]^+$ : https://math.stackexchange.com/questions/86043/reciprocal-of-a-continued-fraction.

Is there anything that we can say about the reciprocal $1/[a_1,\dots,a_n]^-$ of a reduced regular continued fraction $[a_1,\dots,a_n]^-$?

Also are there any books or papers about properties of reduced regular continued fractions?

Answer 1

It is better to look at two reduced regular continued fractions (RRCF) $$\frac{p}q=1-\cfrac{1}{a_1-\cfrac{1}{\cdots-\cfrac{1}{a_n}}}=\cfrac{1}{b_1-\cfrac{1}{\cdots-\cfrac{1}{b_m}}}$$ as on two sides of classical continued fraction (CCF). First fraction gives upper convergents, while the second one gives lower convergents. The union of these two sets is the set of best approximations of the first kind. They are either convergents or intermediate fractions of the CCF representing given number.

They are also clearly visible from geometrical point of view. Let $L$ be a lattice with the basis $(q,0)$, $(p,1)$. We can define two sails $$S_+=\partial\,\mathrm{Conv}\{(x,y)\in L: x,y\ge 0, (x,y)\ne 0\}$$ $$S_-=\partial\,\mathrm{Conv}\{(x,y)\in L: x\le 0,y\ge 0, (x,y)\ne 0\}.$$ The first RRCF corresponds to the integer points on the boundary of $S_+$, while the second one to the integer points on the boundary of $S_-$. Convergents of CCF correspond to the vertices of $S=S_+\cup S_-.$ Intermediate fractions are integer points on $S$ between vertices.

On the picture below the lattice $L$ has basis $(17,0)$, $(7,1)$ (and contains some unnecessary information). The boundary of the grey region is $S_-$, numbers $s_j$ and $q_j$ are continuants.

Explicit formulae for the coordinates of all these points can be written in terms of continuants, see definition here.