2
$\begingroup$

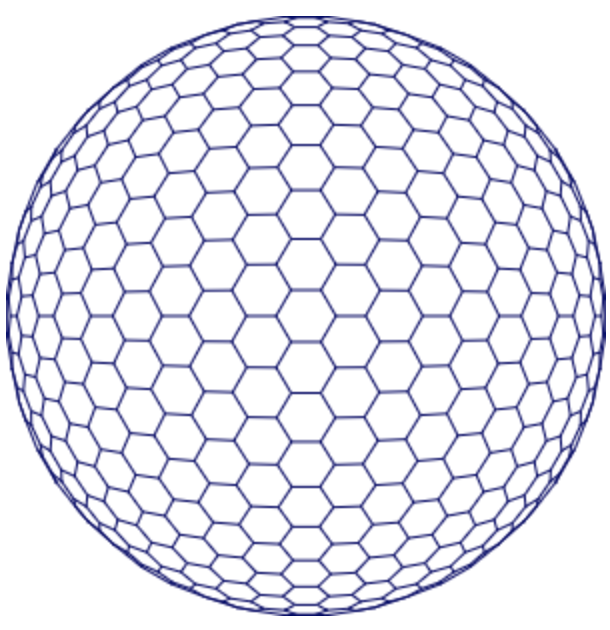
Let $S^2$ be the 2 dimensional sphere embedded in $\mathbb{R}^3$. It is well known, using the Euler characteristic, that it is not possible to tessellate $S^2$ with all congruent regular hexagons.

My question is, would be it possible to partition $S^2$ with congruent regular hexagons, and only a finite number of regular $k$-gons, with $k\neq 6$? That is, does it exist a constant $C$ such that, for any $N$, would it be possible to partition $S^2$ using at least $N-C$ congruent regular hexagons, with the remaining ones being regular $k$-gons, $k\neq 6$? ($C$ has to be independent of $N$)

I have tried using Goldberg polyhedra (icosahedral ones), which has only 12 regular pentagons and the rest being regular hexagons, and then projecting, using the gnomonic projection, the resulting partition on the sphere. However, equal sized hexagons on the icosahedron are projected to non equal sized ones on the sphere, due to the distorsions of the gnomonic projection...

Thanks!

Improve this question
$\endgroup$
8
  • $\begingroup$ Is your projection the same as a ray from the center of $S$ through each vertex of the polyhedron? $\endgroup$
    – Joseph O'Rourke
    Commented Sep 24, 2023 at 20:48
  • 7
    $\begingroup$ All spherical regular hexagons have interior angles larger than 120 degrees, which blocks even three from sharing a vertex. $\endgroup$
    – Akiva Weinberger
    Commented Sep 24, 2023 at 21:23
  • 3
    $\begingroup$ In fact, even non-regular congruent hexagons wouldn't work for this, because the set of valid angle triples adding up to 360 degrees in such a hexagon would be insufficient to extend a patch arbitrarily far (otherwise the polygon would tile the plane, which it can't do with an angle sum over 720). Of the finitely many sets of such triples, one will extend maximally far - that's an upper bound on how large a patch of congruent hexagons can get on the sphere. $\endgroup$
    – RavenclawPrefect
    Commented Sep 25, 2023 at 0:29
  • $\begingroup$ The standard soccer ball contains 20 regular hexagons and 12 pentagons. Not sure whether one can do better. $\endgroup$
    – quarague
    Commented Sep 25, 2023 at 6:45
  • $\begingroup$ @Joseph O'Rourke Yes, it is quite the same, with the tangent planes of the gnomonic projection (en.wikipedia.org/wiki/Gnomonic_projection) centered around the centers of the hexagons in the icosahedron $\endgroup$
    – maria_c
    Commented Sep 26, 2023 at 17:19

2 Answers 2

Reset to default
1
$\begingroup$

If you can accept a slight irregularity then you can have sixty congruent hexagonal faces in the $72$-hedron pictured below[1](https://www.researchgate.net/publication/288751841_The_Architecture_and_Growth_of_Extended_Platonic_Polyhedra). Although not exactly regular, the congruence of the hexagons is guaranteed by the sixty rotational elements of the icosahedral pointvgroup to which this figure belongs. The twelve pentagons occupy less of the surface area in this figure than in the soccer ball. Illustration from 1.

enter image description here

Reference

  1. Deng, Tao & Yu, M.-L & Hu, Guang & Qiu, W.-Y. (2012). "The Architecture and Growth of Extended Platonic Polyhedra". Match. 67. 713-730.
Improve this answer
$\endgroup$
0
2
$\begingroup$

Pardon the frivolity, but I almost fell for this clever April-1st spoof:

A. V. Akopyan, J. Crowder, H. Edelsbrunner, R. Guseinov. "Hexagonal tiling of the two-dimensional sphere." Abstract Link.

Keenan Crane

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ The subtle part of this joke is it's actually the truth — sort of. The hexagons appear nearly regular only in this cross-sectional projection. If you rotate the computer image so as to view a different projection, you find the hexagons becoming increasingly elongated until they run into the pentagons which are, indeed, hidden in the other hemisphere. $\endgroup$
    – Oscar Lanzi
    Commented Oct 29, 2023 at 21:33

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .