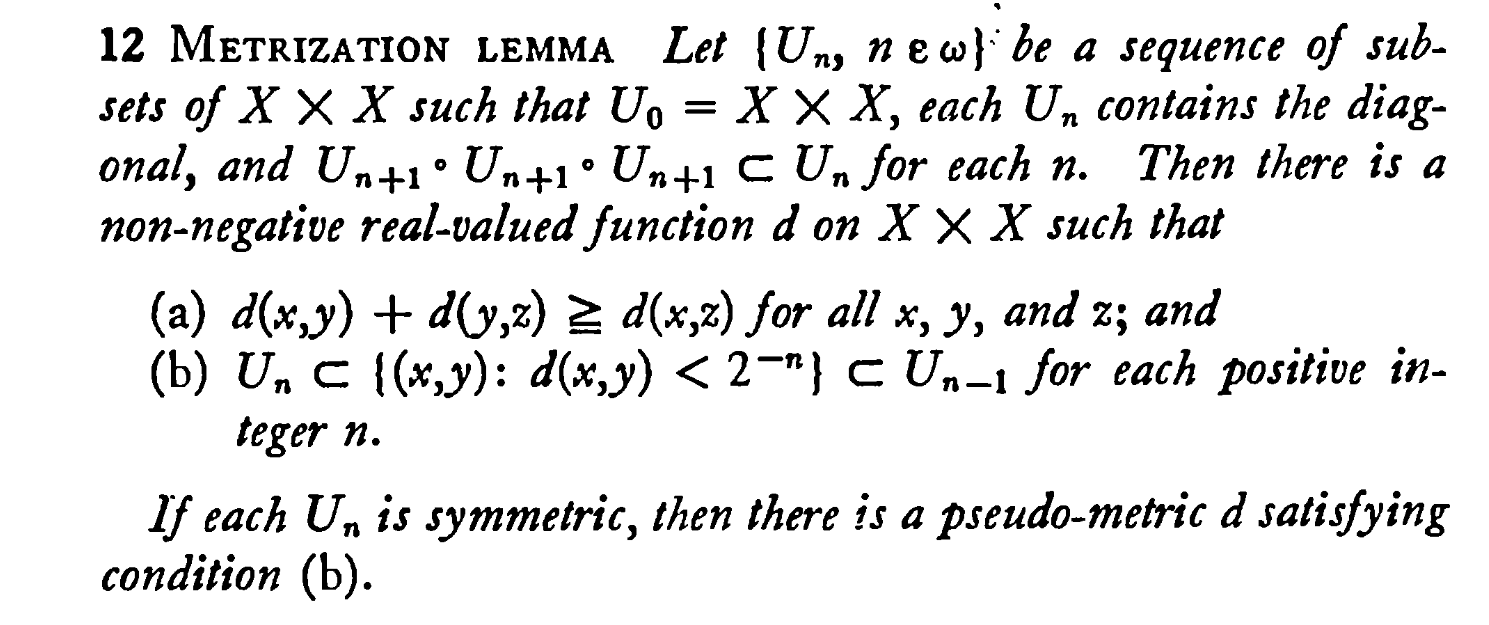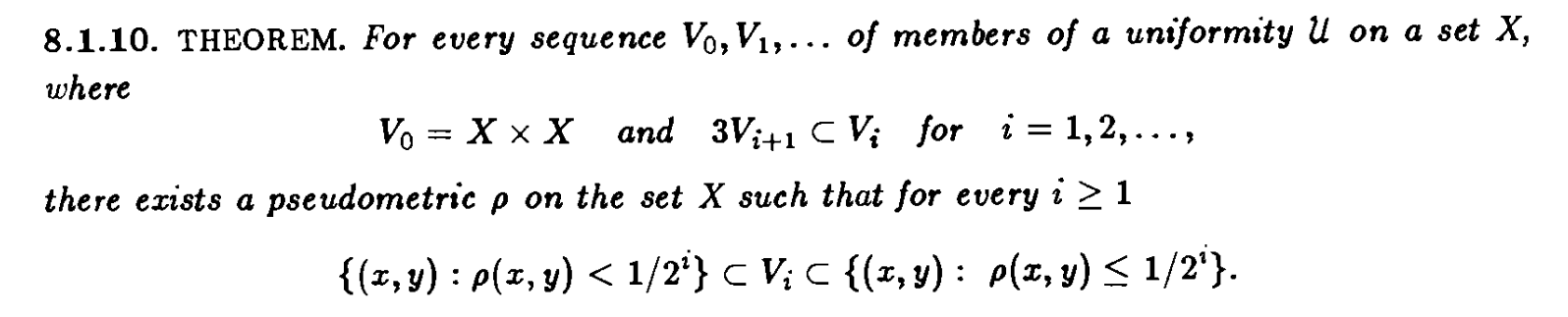The relationship between the two pseudometrics is the following: $\rho(x,y)=2\cdot d(x,y)$ for all $x$ and $y$.
We compare the definitions of the pseudometrics in both books.
Both start with a sequence $\langle U_n:n\in\omega\rangle$ of sets that
contain the diagonal.
The demands on the sequence are: $U_0=X\times X$,
and $U_{n+1}\circ U_{n+1}\circ U_{n+1}\subseteq U_n$ for all $n$.
Engelking specifies that the $U_n$ are members of a uniformity $\mathcal{U}$
and thus, according to his definition, the $U_n$ are, in addition, symmetric.
Kelley imposes no further restrictions.
Kelley defines an auxiliary function $f$ on $X\times X$ by
$$
f(x,y)=\begin{cases} 2^{-n} & (x,y)\in U_{n-1}\setminus U_n\\
0 & (x,y)\in \bigcap_n U_n \end{cases}
$$
and defines
$d(x,y)$ to be the infimum of the set of all sums
$$
\sum_{j=0}^n f(x_j,x_{j+1})
$$
over all finite sequences $\langle x_i:i\le n+1\rangle$ with $x_0=x$
and $x_{n+1}=y$.
Engelking's definition of $\rho(x,y)$ is a bit different: for every
finite sequence $\langle x_i:i\le n+1\rangle$ of points in $X$ with
$x_0=x$ and $x_{n+1}=y$ he takes all possible sums
$$
\sum_{j=1}^{n+1} 2^{-i_j}
$$
subject to $(x_{j-1},x_j)\in U_{i_j}$.
One can rewrite these sums as
$$
\sum_{j=0}^{n} 2^{-i_j}
$$
subject to $(x_j,x_{j+1})\in U_{i_j}$, this merely involves shifting the sequence
$\langle i_j:1\le j\le n+1\rangle$ down by one.
Now observe that, in general, $(x,y)\in U_i$ iff $f(x,y)\le 2^{-(i+1)}$
Indeed: if $(x,y)\in U_i$ then $(x,y)\in U_{n-1}\setminus U_n$ for
some $n$ with $n-1\ge i$, that is for some $n>i$.
And that implies that $f(x,y)=2^{-n}\le2^{-(i+1)}$.
Conversely, if $f(x,y)\le2^{-(i+1)}$ then $(x,y)\in U_{n-1}\setminus U_n$
for some $n\ge i+1$, that is, for some $n>i$.
This means that for every sequence $\langle x_i:i\le n+1\rangle$ and every
associated sum $\sum_{j=0}^{n} 2^{-i_j}$ we have
$$
2\cdot \sum_{j=0}^n f(x_j,x_{j+1}) \le 2\cdot\sum_{j=0}^{n} 2^{-(i_j+1)}
=\sum_{j=0}^{n} 2^{-i_j}
$$
it also implies that $2\sum_{j=0}^n f(x_j,x_{j+1})$ is one of the associated sums,
namely the one where every $i_j$ is such that
$(x_j,x_{j+1})\in U_{i_j}\setminus U_{i_j+1}$.
This implies that $\rho(x,y)=2\cdot d(x,y)$ for all $x$ and $y$.