When I know self convolution of the complex function can I recover function itself or its modulus?

Question

I have a function $A : \mathbb{R} \to \mathbb{C}$.

I know there exists unknown function $u: \mathbb{R} \to \mathbb{C}$, such that $A$ is convolution of $u$ and its complex conjugate $A = u * u^*$.

I would like to compute function $|u|^2 = uu^*$. Is there unique solution to this problem and is there efficient computational method how to find it?

Answer 1

$\newcommand\R{\mathbb R}\newcommand\C{\mathbb C}$No, there is no uniqueness here.

Indeed, let $\hat f$ denote the Fourier transform of a (say integrable) function $f\colon\R\to\C$, so that $\hat f(t)=\int_\R e^{itx}f(x)\,dx$ for real $t$. Then $\widehat{u^*}(t)=\hat u(-t)^*$ for real $t$, and the equation $$u*u^*=A \tag{1}\label{1}$$ for $u$ becomes the equation $$\hat u(t) \hat u(-t)^*=\hat A(t) \tag{2}\label{2}$$ for $\hat u$.

Clearly, equation \eqref{2} can have multiple solutions. So, equation \eqref{1} can have multiple solutions.

---

E.g., suppose that $\hat A(t)=t^2e^{-t^2}$, $$\hat u_1(t)=|t|e^{-t^2/2},\quad\text{and}\quad \hat u_2(t)=e^{-t^2/2}\,t\,(2\,1(t>0)-\tfrac12\,1(t<0))$$ for real $t$. Then $\hat u_1$ and $\hat u_2$ are two different solutions of \eqref{2}.

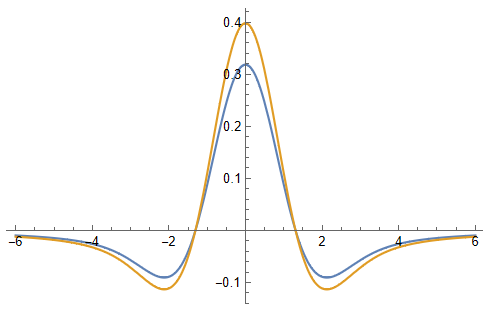
The corresponding $A$ and solutions $u_1,u_2$ of \eqref{1} are given by $$A(x)=\frac1{2\pi}\,\int_\R e^{-itx} \hat A(t)\,dt= \frac{2-x^2}{8 \sqrt{\pi }}\,e^{-x^2/4}, $$ $$u_1(x)=\frac1{2\pi}\,\int_\R e^{-itx} \hat u_1(t)\,dt =\frac{1}{\pi } -\frac 1{\sqrt{2 \pi }} e^{-x^2/2} x\, \text{erfi}\left(\frac{x}{\sqrt{2}}\right),$$ $$u_2(x)=\frac1{2\pi}\,\int_\R e^{-itx} \hat u_2(t)\,dt =\frac{5}{4 \pi }-\frac x{4 \sqrt{2 \pi }}e^{-x^2/2} \left(3 i+5\, \text{erfi}\left(\frac{x}{\sqrt{2}}\right)\right) \\ =\frac54\,u_1(x)-\frac{3ix}{4\sqrt{2\pi}}\,e^{-x^2/2} $$ for real $x$, where $\text{erfi}(x):=\sqrt{\frac2\pi}\int_0^{x\sqrt2}e^{z^2/2}\,dz$.

Here are the graphs of $u_1$ (blue) and $\Re u_2$ (gold) over the interval $[-6,6]$:

Answer 2

Another counterexample with simpler functions.

I work with even and real functions so their Fourier transform are also even and real functions. Hence, one can omit complex conjugations and replacements of the argument of functions by its opposite.

The functions $u_\pm$ defined by $$u_\pm(x) = (e^{i2x}+e^{-i2x}\pm 1)\frac{\sin^2 x}{x^2} = (2\cos(2x)\pm 1)\frac{\sin^2 x}{x^2}$$ do not have the same modulus but verify $u_+*u_+ = u_-*u_-$.

Indeed, call $f$ the tent function, defined by $f(t) = \max(0,1-|t|)$. Then $f$ is continuous with support $[-1,1]$. Thus the functions $x \mapsto f(x-2) + f(x+2) \pm f(x)$ have the same square and their Fourier transform, which are $u_\pm$. Hence $u_+*u_+ = u_-*u_-$.