I have to proof this:
If is a Levy process then for each
the sample path
is, with probability 1, continuous as s=t.
This is the proof:
I don't understand the conclusion. Can someone explain to me how the proof ends? Is there an alternative way to do this?
Thanks
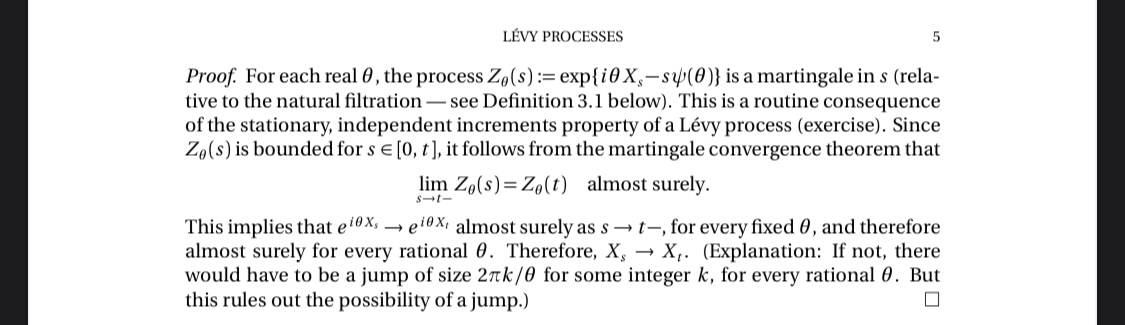
$\newcommand{\R}{\mathbb R}\newcommand{\Q}{\mathbb Q}$This proof is insufficient (where did you take it from?).
Indeed, the logic at the end of the proof boils to down to
Proposition 1: Suppose that for some real $t>0$, some function $[0,\infty)\ni u\mapsto x_u\in\R$, and all $q\in\Q$ we have \begin{equation*} e^{iqx_s}\to e^{iqx_t} \tag{1}\label{1} \end{equation*} (as $s\uparrow t$). Then $x_s\to x_t$.
However, this proposition is false in general. E.g., suppose that \begin{equation*} x_s=x_t+2\pi\Big(\Big\lceil\frac1{t-s}\Big\rceil\Big)! \end{equation*} for some real $t>0$ and all $s\in[0,t)$. Then \eqref{1} holds for each $q\in\Q$, whereas $x_s\not\to x_t$ (as $s\uparrow t$).
(The rate of growth of $x_s$ to $\infty$ can be made arbitrarily slow by replacing $\frac1{t-s}$ by $g(t-s)$, where $g(u)$ grows to $\infty$ arbitrarily slowly as $u\downarrow0$.)
However, with the additional assumption that the function $(x_u)$ be locally bounded, Proposition 1 would be true. (See also the remark at the end of this answer.) Indeed, let \begin{equation*} y_s:=\frac{x_s-x_t}{2\pi}. \end{equation*} Then \eqref{1} can be rewritten as \begin{equation*} qy_s-k_{q,s}\to0 \tag{1a}\label{1a} \end{equation*} for each $q\in\Q$ (as $s\uparrow t$), where $k_{q,s}$ is an integer depending only on $q$ and $s$. Since the function $(x_u)$ is locally bounded, \eqref{1a} implies that without loss of generality (wlog) $2|k_{q,s}|<k_q$ for some integer $k_q$ depending only on $q$.
We want to show that $y_s\to0$. To obtain a contradiction, suppose the contrary. Then wlog $y_s\to c$ for some real $c\ne0$ (because the function $(x_u)$ be locally bounded). So, for any nonzero $q\in\Q$ wlog $k_{q,s}\ne0$ and hence \begin{equation*} k_{q,s}\sim qy_s \end{equation*} and, similarly and therefore, \begin{equation*} k_{q/k_q,s}\sim (q/k_q)y_s\sim k_{q,s}/k_q\in[-1/2,1/2], \end{equation*} which contradicts the condition that $k_{q/k_q,s}$ is a nonzero integer. $\quad\Box$
Remark: For $(x_u)$ to be locally bounded, it is enough that $(x_u)$ be right-continuous in $u\ge0$ and have finite left limits in $u>0$. Indeed, then for each real $t\ge0$ there is a neighborhood $U_t$ of $t$ in $[0,\infty)$ such that $(x_u)$ is bounded in $U_t$. Now the local boundedness of $(x_u)$ follows by the Heine--Borel lemma.
