Understanding the definition of left homotopy as given in Quillen’s Homotopical algebra book

Question

Given two topological spaces $X,Y$, and two maps $f,g:X\rightarrow Y$, there is a notion of homotopy between $f$ and $g$. It is given by a continuous map $H:X\times I\rightarrow Y$ such that the composition $H\circ i_0$ gives $f$ and $H\circ i_1$ gives $g$, where $i_0,i_1$ are what you expect it to be.

Quillen defines left homotopy in the following way:

Let $\mathcal{C}$ be a model category. Let $f,g:A\rightarrow B$ be maps. We say that, $f$ is left homotopic to $g$ if there is a diagram of the form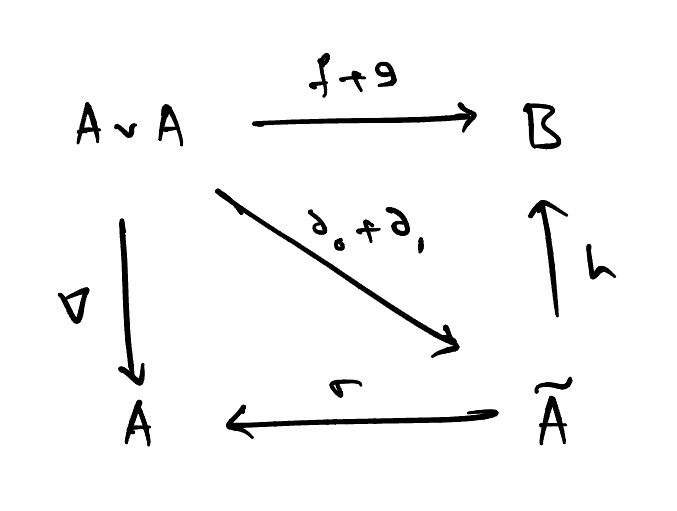

where $\sigma$ is a weak equivalence.

Only after comparing with the notion of homotopy in topological spaces, it can be guessed that they are referring to homotopy as the map $h:\widetilde{A}\rightarrow B$ (as in case of topological spaces, we had a map $A\times I\rightarrow B$). The above condition of $H\circ i_0$ gives $f$ and $H\circ i_1$ gives $g$, seems to be combined in just one diagram (upper triangle) by saying $h\circ (\partial_0+\partial_1)=f+g$. Though it is not very clear, it looks like this is the case.

But, then, there would be no need to mention the map $\sigma$. So, May be the notion of left homotopy is given by not just one morphism, but by a pair of morphisms $(\sigma, h)$ where $\sigma$ is a map from the “special object” to $A$ and $h$ is (the usual, as in the case of topological spaces) a map from the “special object” to $B$. Then, there is a map $\partial_0+\partial_1:A\wedge A\rightarrow \widetilde{A}$. I think it it safe to think this as a pair of maps $(\partial_0:A\rightarrow\widetilde{A}, \partial_1: A\rightarrow\widetilde{A})$. Thus it looks like a left homotopy is a $5$-tuple, out of which, one is an object $\widetilde{A}$, and the other 4 are maps,

$$(\partial_0:A\rightarrow\widetilde{A}, \partial_1: A\rightarrow\widetilde{A}, \sigma: \widetilde{A}\rightarrow A, h:\widetilde{A}\rightarrow B)$$ satisfying certain conditions.

Question :

1. Did I understood correctly? Or, did I misunderstand some part?
2. In case of topological spaces, there is no notion of left or right homotopy. But, in this case, what was the justification for introduction of left and right notion. In case of topological spaces, there is no notion similar to $\sigma$. What is the justification to introduce the extra data here.
3. Why is it done differently in the recent books?

Answer 1

The key observation you need to make sense of Quillen's definition is that $\tilde{A}$ is "the cylinder object on A." In topological spaces, that's $I\times A$ but not every model category has an interval object like $I$, so we wouldn't want to specify this particular definition of a cylinder object. It's better to be more general, and let the cylinder object be any object $\tilde{A}$ fitting in a factorization of $\nabla: A\vee A \to A$ into a cofibration followed by a trivial fibration.

In Top, you can also define homotopy via the "path space object" $Y^I$, i.e., the space of paths in $Y$. But, for a general model category without an interval I, it's better to define the path space object as a factorization of the diagonal map $X\to X\times X$ into a trivial cofibration followed by a fibration.

A priori, these two definitions of homotopy could be different, hence calling the first one "left homotopy" and the second one "right homotopy." It's a theorem that if $M$ is actually a model category, then these two notions of homotopy coincide. But, for any old category with chosen classes of morphisms called weak equivalences, cofibrations, and fibrations, they wouldn't have to coincide.

Being a student of Mark Hovey, I have to recommend his book on model categories, which spells this out in general and also for topological spaces.