1
$\begingroup$

PeakFit (Systat, v. 4.12) is a software for fitting experimental peaks obtained in physics or chemical experiments. Under the miscellenous peak functions, it shows the following equations with a name, "Pulse peak modified with a power term". No reference or further documentation is provided. If we search Google, Google Scholar and even Google Books for the same term there is no result.

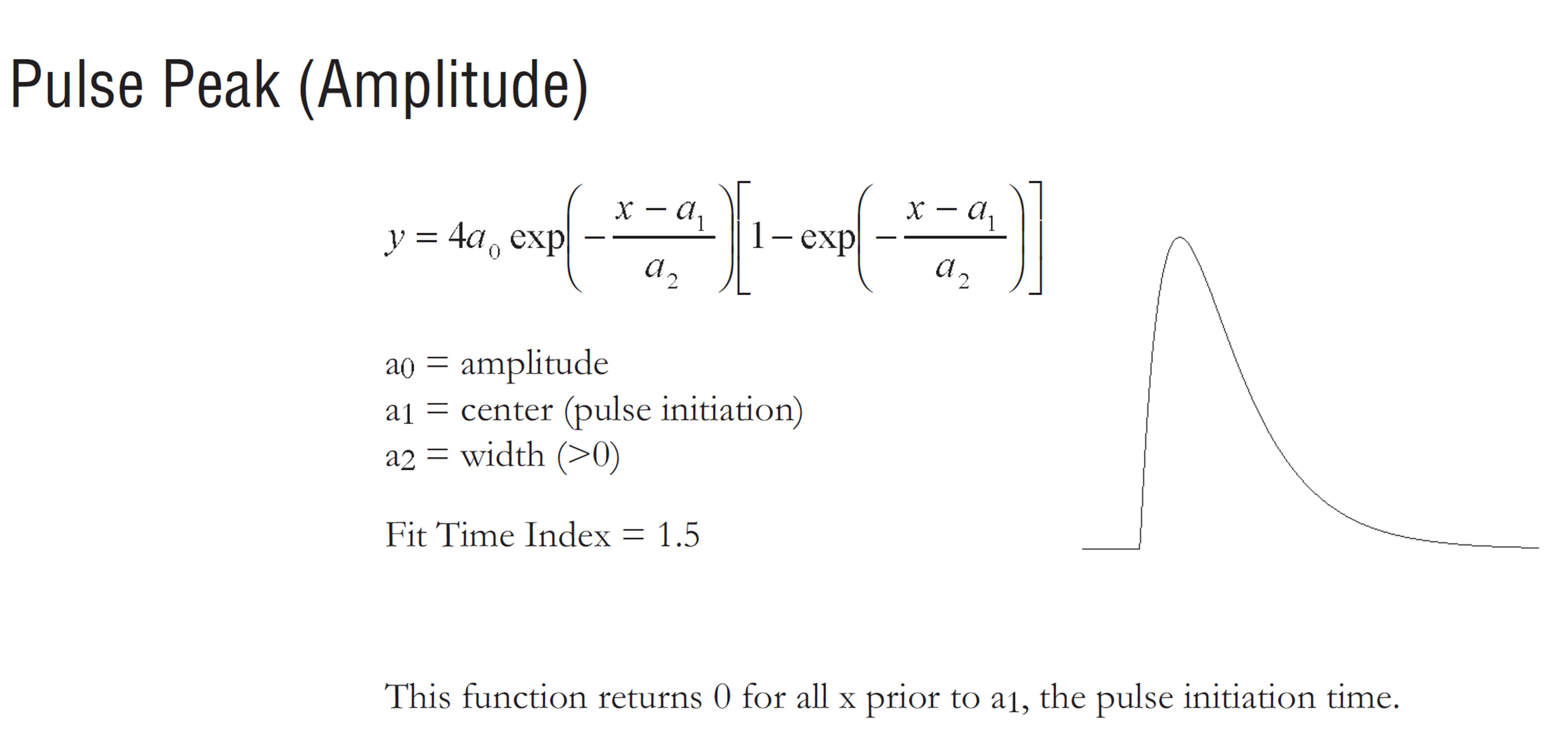
For reference, the "simple" pulse peak function is shown below as it appears in the software manual without any reference.

enter image description here

I am trying to find a reference or the name of this function shown below to see the background/origin or derivation of this peak function. Most likely, this must be a well studied function with a different name in mathematics. My background is chemistry.

$$ y=\frac{a_0\left[1-\exp \left(-\frac{x-a_1}{a_2}\right)\right]^{a_3} \exp \left(-\frac{x-a_1}{a_2}\right)}{a_3^{a_3}\left(a_3+1\right)^{-a_3-1}} $$

$$ \begin{aligned} &a_0=\text { amplitude } \\ &a_1=\text { center (pulse initiation) } \\ &a_2=\text { width }(>0) \\ &a_3=\text { shape }(>0) \end{aligned} $$

enter image description here

enter image description here

Improve this question
$\endgroup$
1
  • $\begingroup$ Maybe it is a convolution of a square pulse with some function. I have seen experimental flow cell data fit this function, but never knew the origin of it. $\endgroup$
    – Troy Handlovic
    Commented Sep 28, 2022 at 20:01

0

Reset to default

You must log in to answer this question.