2
$\begingroup$

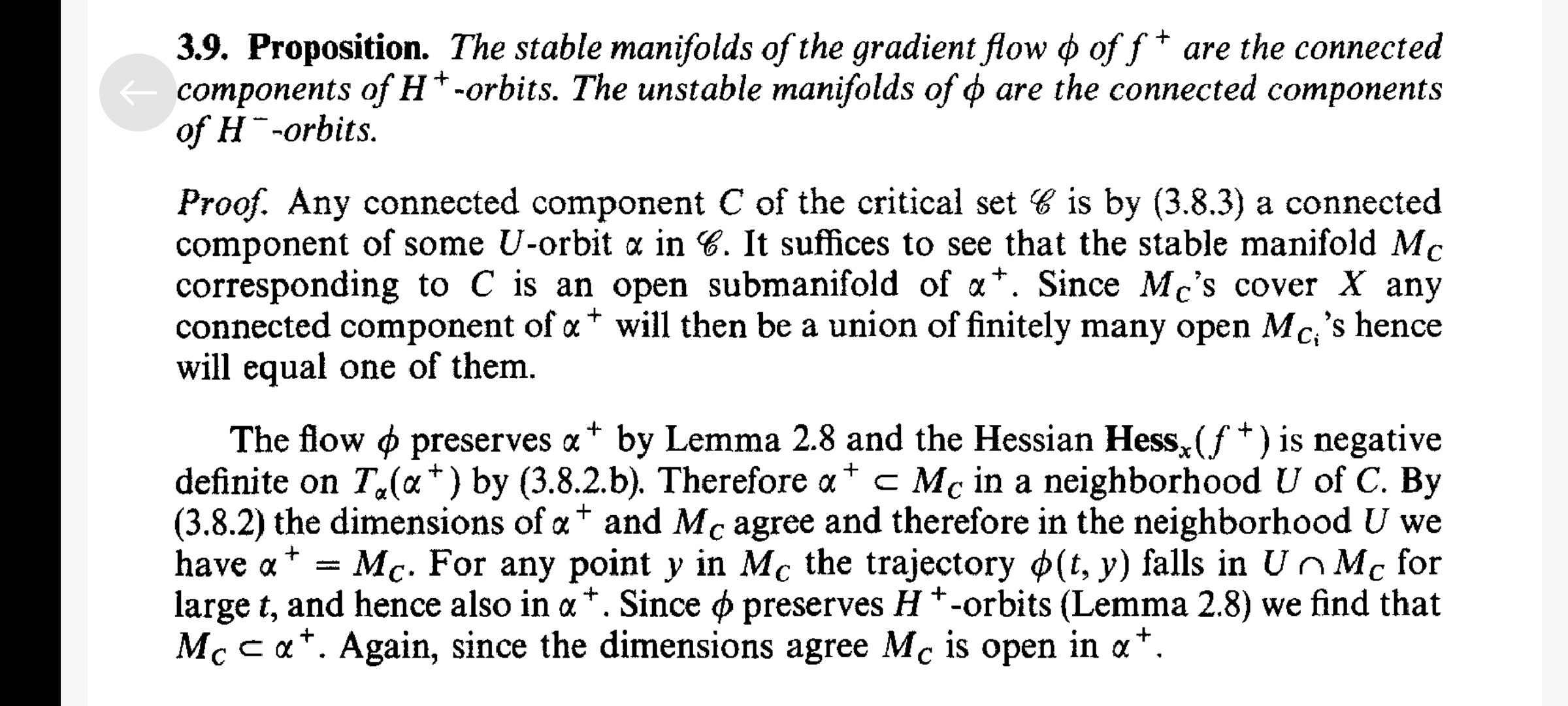
Let $X$ be a Riemannian compact manifold on which acts a compact Lie group $H^+$. Let $f^+ : X \rightarrow \mathbb{R} $ be a smooth function on $X$. Consider a Lie subgroup $U$ of $H^+$ and suppose that $U$ acts on the set of critical points of $f^+$. Denote by $\phi $ the flow of the gradient vector field of $f^+$. Suppose that $\phi$ is $U$-invariant, and that $\lim_{t \rightarrow +\infty} \phi_t(x)$ exists and it belongs to the set of critical points of $f^+$ for all $x \in X$.$\DeclareMathOperator{\Hess}{\operatorname{Hess}}$

Let $\alpha:= U.x_0$ be a $U$-orbit which pass through a critical point $x_0$ and denote by $\alpha^+:= H^+.x_0$ the corresponding $H^+$-orbit on $X$. Let $C$ be a connected component of $\alpha$ and let $M_C$ be the set $M_C:= \lbrace x \in X, \lim_{t \rightarrow+ \infty} \phi_t(x) \in C \rbrace $.

$\textbf{Question}$: Show that if flow $\phi$ preserves the orbit $\alpha ^+$ and if the Hessian $\Hess_x(f^+)$ is negative definite on $T_x( \alpha^+)$ for all $x \in \alpha $, then $\alpha^+ \subset M_C $ in a neighborhood $V$ of $C$.

My motivaion to ask this question is to understand the proof of proposition 3.9 in the paper Matsuki correspondence for sheaves.

enter image description here

Improve this question
$\endgroup$
3
  • 1
    $\begingroup$ I have ask the same question in MSE here math.stackexchange.com/questions/4524311/… but didn't receive any comments or answer. $\endgroup$
    – Mira
    Commented Sep 4, 2022 at 14:20
  • $\begingroup$ May you provide the exact reference of Matsuki article? $\endgroup$
    – user56980
    Commented Sep 8, 2022 at 12:37
  • $\begingroup$ @user56980, yes , the reference is this: I. Mirković, T. Uzawa & K. Vilonen Matsuki correspondence for sheaves Invent. Math. 109, (1992). $\endgroup$
    – Mira
    Commented Sep 8, 2022 at 15:36

2 Answers 2

Reset to default
1
+100
$\begingroup$

The authors use the Morse-Bott theory for the equivariant moment map $f^+$. Any connected component $C$ of the critical set of $f^+$ is a submanifold and for any critical point $x\in C$, $T_xC=\ker\mathrm{Hess}_x(f^+)$. Moreover $$T_xX=T_xC\oplus E_x^-\oplus E_x^+$$ where $E_x^-$ is spanned by the negative eigenspaces and $E_x^+$ is spanned by the positive eigenspaces of $\mathrm{Hess}_x(f^+)$ (the hessian is symmetric, thus all its eigenvalues are real).

If $U$ is connected, then $U\cdot x\subset C$ and since $X$ is compact, it's partitioned into a finite number od stable (resp. unstable) manifolds $W^\pm(C)=\{x\in X,\ \lim_{t\to\pm\infty}\phi_t(x)\in C\}$, where $\phi_t$ is the (global) flow of the gradient (or minus-gradient). Take a look to the following paper (pages 6-7):

The Atiyah-Guillemin-Sternberg convexity theorem

Improve this answer
$\endgroup$
8
  • $\begingroup$ .@user56980, Could you please explain How to Find the neighborhood $V$ in my question ? $\endgroup$
    – Mira
    Commented Sep 11, 2022 at 23:38
  • 1
    $\begingroup$ The notations are somehow confusing. The neighborhood $V$ can be deduced from the Morse-Bott lemma (also compare the dimension of $M_C$ given by the number of positive eigenvalues of the hessian and the codimension of $\alpha^+$). $\endgroup$
    – user56980
    Commented Sep 12, 2022 at 10:47
  • $\begingroup$ For the Morse-Bott lemma: sciencedirect.com/science/article/pii/S0723086904800148 $\endgroup$
    – user56980
    Commented Sep 12, 2022 at 10:47
  • 1
    $\begingroup$ Use the neighborhood $U$ (theorem2, page 366). The function $f$ in the Morse chart is $f(C)-y_1^2-\ldots -y_k^2+y_{k+1}^2+\dots+y_n^2$. To simplify, $-\nabla f$ is $(2y_1,\ldots,2y_k,-2y_{k+1},\ldots,-2y_n)$. Its flow is $(e^{2t}y_1,\ldots,e^{2t}y_k,e^{-2t}y_{k+1},\ldots,e^{-2t}y_n)$...the hessian is $Diagonal(2,...,2,-2,...,-2)$, $W_C=\phi^{-1}(\{0\}\times\mathbb{R}^{n-k})$. Use this to show that $U\cap\alpha^+\subset M_C$ $\endgroup$
    – user56980
    Commented Sep 12, 2022 at 20:10
  • 1
    $\begingroup$ (Now I'm wondering: the Morse chart doesn't have to be isometric, right? Which means it doesn't have to preserve gradient flow, right? So how do we know it preserves stable points?) $\endgroup$
    – Jonathan L Long
    Commented Sep 12, 2022 at 20:24
1
$\begingroup$

The facts that the Hessian is negative definite on the normal bundle $T_\alpha (\alpha^+)$ to $\alpha$ in $\alpha^+$ and that $\alpha$ are all critical points tells you that there is a neighborhood $V$ of $C$ in $\alpha^+$ such that $V \subseteq M_C$. (In other words, near enough to $C$, the gradient flow will return you to $C$.) Of course, it follows that $\alpha^+ \subseteq M_C$ in that neighborhood.

(Note that $T_\alpha (\alpha^+)$ in (3.8.2.b) apparently refers to the normal bundle to $\alpha$ in $\alpha^+$. The Hessian is not negative definite across any tangent space $T_x (\alpha^+)$ at a point $x \in \alpha$, since it is zero over the directions tangent to $\alpha$, (3.8.2.a).)

Improve this answer
$\endgroup$
3
  • $\begingroup$ .@Jonathan L Long How did you prove the existence of the neighborhood $V$ ? $\endgroup$
    – Mira
    Commented Sep 11, 2022 at 23:33
  • 1
    $\begingroup$ It seems to me this amounts to stability analysis in the theory of ODEs; the Hessian is precisely what determines local stability under integration of the gradient vector field (it would more commonly be called the Jacobian in the ODE context, where the vector field need not itself be a gradient). Does that help or have I misunderstood the missing piece? $\endgroup$
    – Jonathan L Long
    Commented Sep 12, 2022 at 2:31
  • 1
    $\begingroup$ (I think user56980's answer is much more direct within the Morse theory context; consider the above if you're concerned with how you could get this point from first principles.) $\endgroup$
    – Jonathan L Long
    Commented Sep 12, 2022 at 19:56

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .