On why this is here I tried posting on math stackexchange but I got no comments or answers. I even bountied the question but I am still not getting any responses. I am getting the sense that I wasn't posting in the correct forum. If this is also not the correct place to ask this please let me know.
I would like to prove the following result
I will be quoting the previous result and its proof :
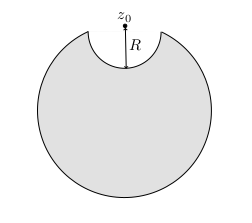
Proof for Theorem 1.4 Suppose $\exists (a,b)\in D(0,1)$ such that $u(a,b)>0$. Let \begin{align*} X = \left\{z: |z|\leq 1 \quad \text{and} \quad z\neq z_0 \right\} \end{align*} If $u$ has a maximum in $D(0,1)$, then apply the same argument as in the proof of the above theorem. Else consider $C_{R}$ defined by \begin{align*} C_{R}=\left(D(0,1) \cup \partial D(0,1)\right) \setminus D(z_0,R) \end{align*} illustrated as
By construction $u$ achieves its minimum and maximum on the boundary $\partial C_{R}$. Let \begin{align*} M= \sup \left\{\max_{C_{R}}u \mid 0<R<1 \right\} \end{align*} which exists as $u$ is bounded and because the set is non empty. By assumption $u$ can not achieve $M$ on $X$.
Now given $\epsilon>0$, define \begin{align*} T=\left\{\max_{C_{R}}u \mid 0<R<\epsilon \right\} \end{align*} By construction \begin{align*} M=\sup T. \end{align*} By definition, given $\delta >0$, there exists $\tau $ with $0<\tau<\epsilon$ such that \begin{align*} \max_{C_{\tau }}u & > \sup T + \delta \\ & = M +\delta \end{align*} by applying the definition of supremum of $T$. Now we use continuity. For any point $z_1 $ on the boundary of the open disc, except for $z_0$, we have that given $A>0$ , there exists $B>0$ such that \begin{align*} |z-z_1 |<B \implies |u(z)-u(z_1 )|<A \end{align*} By hypothesis, the maximum on $C_{\tau }$ lies on the semi circle. Let $z_2$ be that point. Then let $z_3$ be one of the points where the circle and semi circle intersect. Then \begin{align*} |z_3-z_2|<2\tau \end{align*} geometrically as they lie on the same circle of radius $\tau $. Take $A<M$. Now set $\epsilon<\frac{B}{2}$, so $\tau<\frac{B}{2}$. Then \begin{align*} |z_3 -z_2|<B \end{align*} and \begin{align*} |u(z_3)-u(z_2)|& = |u(z_2)| \\ & > M +\delta > A \end{align*} which contradicts the continuity.
Question: Does my proof hold?