8
$\begingroup$

I am not very conversant with the growth of a group, so this may be a very silly question.

It is known that $F_2$, the free group of rank $2$, has exponential growth. I was wondering whether the following is true:

If a group has exponential growth does it contain a free subgroup?

Improve this question
$\endgroup$
3
  • 3
    $\begingroup$ It's enough to contain a free subsemigroup. Many solvable groups have free subsemigroups but none have free subgroups. There are more involved examples without free semigroups $\endgroup$
    – Benjamin Steinberg
    Commented May 1, 2022 at 12:31
  • 3
    $\begingroup$ Amenable groups cannot contain $F_2$, but there are amenable groups of exponential growth. $\endgroup$
    – Joseph Van Name
    Commented May 1, 2022 at 13:55
  • $\begingroup$ Many groups admit a free semigroup of rank 2 and hence have exponential growth, yet are solvable. For instance any semidirect product $\mathbf{Z}^n\rtimes_A\mathbf{Z}$ with $A$ not virtually unipotent has a free subsemigroup of rank 2. $\endgroup$
    – YCor
    Commented May 1, 2022 at 14:24

2 Answers 2

Reset to default
12
$\begingroup$

A famous theorem of Wolf shows that the growth of a solvable group is either polynomial or exponential. So no intermediate growth among solvable groups. And a famous theorem of Gromov shows that having polynomial growth implies being virtually nilpotent. Consequently, any solvable group that is not virtually nilpotent provides an example of a group with exponential growth but no non-abelian free subgroups.

Of course, using big theorems is not necessary to find explicit examples, but it gives some general perspectives, and it justifies that many examples exist. One simple example is the Baumslag-Solitar group $BS(1,2)$. It has a Cayley graph that is sufficiently simple to be drawn.

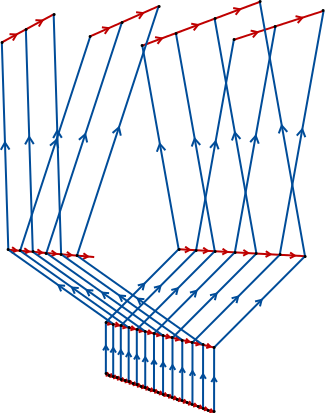
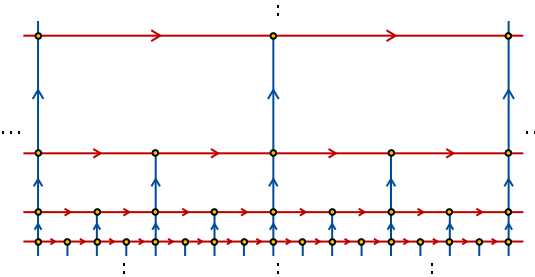
enter image description here

enter image description here

The pictures are taken from Wikipedia, where there is also a nice animation.

Improve this answer
$\endgroup$
0
6
$\begingroup$

Not necessarily. -- For example, the lamplighter group has exponential growth, but does not have a free subgroup of rank 2 (if $a$ and $b$ are two elements of that group of infinite order, then there are always nonzero integers $i$ and $j$ such that $a^ib^j$ is either the identity or has order $2$).

Improve this answer
$\endgroup$
6
  • 1
    $\begingroup$ Do you know if the OP’s idea holds for finitely presented groups? $\endgroup$
    – user44143
    Commented May 1, 2022 at 12:28
  • 2
    $\begingroup$ For a finitely presented group with exponential growth and no non-abelian free subgroups you can use Thompson's group F. I think there should also be solvable (i.e., easier) examples too. $\endgroup$
    – Matt Zaremsky
    Commented May 1, 2022 at 13:50
  • 4
    $\begingroup$ The solvable Baumslag-Solitar groups $\langle x,y \mid y^{-1}xy=x^k \rangle$ for $|k| > 1$ are examples. $\endgroup$
    – Derek Holt
    Commented May 1, 2022 at 13:52
  • 3
    $\begingroup$ A semidirect product $\mathbb Z^2\rtimes \mathbb Z$ is solvable and finitely presented. If the action is hyperbolic, as is generically true, it has exponential growth. $\endgroup$
    – Ben Wieland
    Commented May 1, 2022 at 14:10
  • $\begingroup$ Why is it that if every you have two elements of the Lamplighter group of infinite order you can arrange then in a way such that the square is the identity? Is this easily derived from its presentation? $\endgroup$
    – Joseph Adams
    Commented May 22 at 12:21

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .