Reference request for facts about bi(co)descent objects

Question

I know the following facts are true, but I struggle to find adequate references for them:

1. Let $T$ be a pseudo-monad on a bicategory $\mathcal{C}$, and let $A$, $B$ be pseudo-algebras for $T$. Then, the following descent diagram 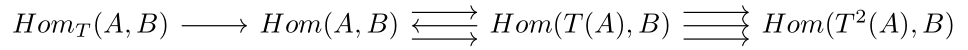is a bilimit in $\mathcal{C}$.
2. Let 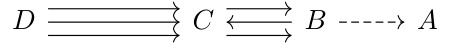be a bicodescent diagram in $\mathcal{C}$. The object $A$ is a bicolimit of this diagram if and only if the pseudofunctor $H:\mathcal{C}\rightarrow \mathbf{Bicat}$ defined as the bilimit 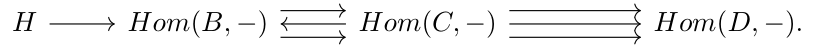in the bicategory of pseudofunctors from $\mathcal{C}$ to $\mathbf{Bicat}$ is represented by $A$.

Does anyone know where to find them in the litterature?

Answer 1

1. This follows from Lemma 2.3 and Proposition 3.2 of Creurer–Marmolejo–Vitale's Beck's theorem for pseudo-monads, together with fact that the bicategorical Yoneda embedding preserves bilimits.
2. Presumably you mean a pseudofunctor to $\mathbf{Cat}$, rather than $\mathbf{Bicat}$, because you are working with bicategories rather than tricategories? This then follows from the fact that the bicategorical Yoneda embedding creates bilimits, which is a consequence of the bicategorical Yoneda lemma.