Imaginary only values of the Lambert W function

Question

For which values of $x \in \mathbb{R}$ is the principal branch $W_0$ of Lambert's $W$ function a purely imaginary value, i.e. for which $x$'s is $Re(W_0(x))=0$? For instance, $W_0(2 / \pi) = -i \pi / 2$, so $2/\pi$ would be one element of the set of values I'm interested in.

Answer 1

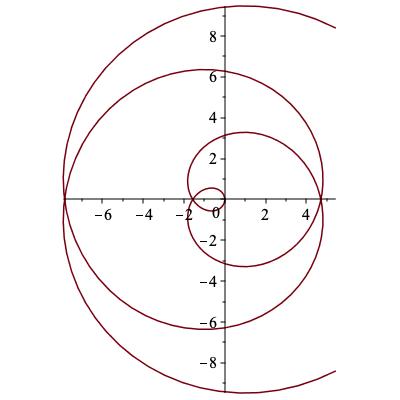
If $W(z) = it$, where $t$ is real, then (definition of $W$) we have $z = ite^{it}$. So: the complex numbers $z$ such that $\operatorname{Re} W(z) = 0$ for at least one branch of $W$ are these: $\{ite^{it} \mid t \in \mathbb R\}$.

Graph of the set

It is a "spiral of Archimedes", two copies, one for $t>0$ and one for $t<0$.

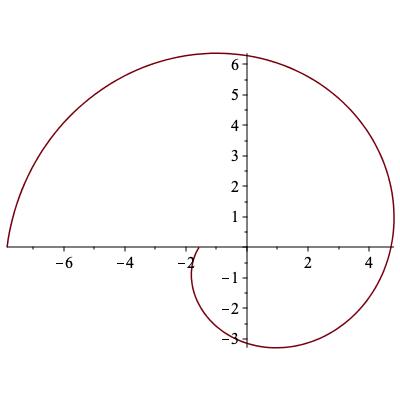
The portion of this corresponding to the branch $W_0$ is $-\pi/2 \le t \le \pi/2$.

The portion of this corresponding to the branch $W_1$ is $\pi/2 \le t \le 5\pi/2$.