On some curves of real values of a rational function

Question

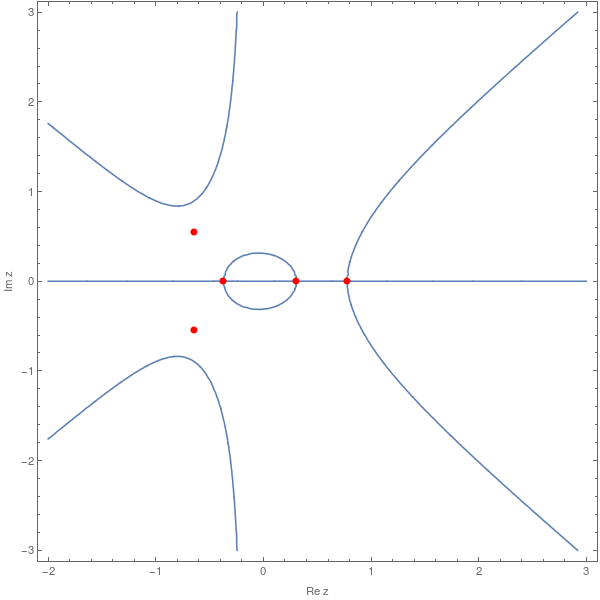
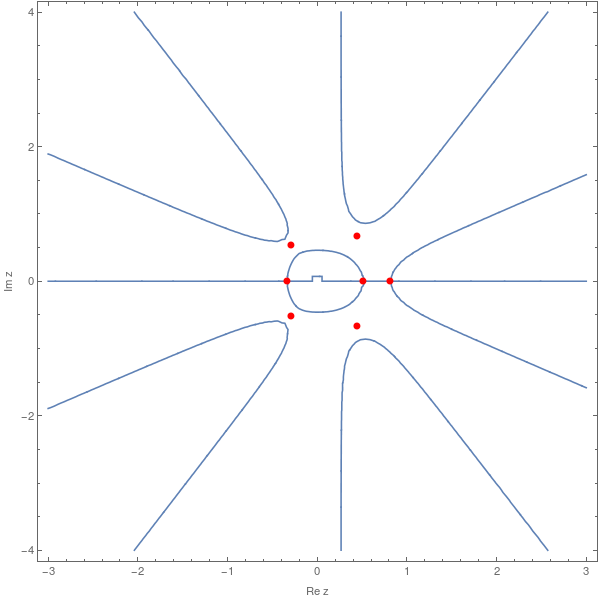
For given parameters $a_{1},\dots,a_{k}\in\mathbb{R}$, define the rational function $\phi:\mathbb{C}\to\mathbb{C}$ as $$\phi(z)=\frac{1}{z}-a_{1}z-a_{2}z^{2}-\dots-a_{k}z^{k}.$$ The domain of its real values $$\Gamma=\{z\in\mathbb{C} \mid \text{Im } \phi(z)=0\}$$ is a union of finitely many algebraic curves in $\mathbb{C}$. I am interested in situations where $\Gamma$ contains a closed algebraic curve, see the pictures below.

Question: Is it possible to deduce a condition in terms of parameters $a_{1},\dots,a_{k}$ (sufficiently general, of course) which would guarantee that the set $\Gamma$ contains a closed algebraic curve? Are there some known results (published papers) related to this topic? Thanks!

Example 1: $$\phi(z)=1/z+10 z+3 z^2-3 z^3-4 z^4$$

The red points are the critical points of $\phi$, i.e., the points where $\phi'(z)\neq0$. The singularity at the origin is not visible. Note the closed algebraic curve in the picture.

Example 2: $$\phi(z)=1/z+5 z-2 z^2+3 z^3-6 z^4+8 z^5-5 z^6$$

Note the closed algebraic curve is again present in this example. (The little error is caused by the singularity in $0$.)

Example 3: $$\phi(z)=1/z+z+3 z^2-3 z^3-4 z^4+3 z^5-10 z^6$$

The set $\Gamma$ contains no closed algebraic curve in this example.

Answer 1

A closed curve where $\Im \phi(z)=0$ is the boundary of a bounded region in the upper half-plane where $\Im \phi(z)<0$, or in the lower half-plane, where $\Im\phi(z)>0$. (This follows from the maximum Principle).

Such region $D$ in the upper half-plane always exists, because $\Im \phi(it)<0$ for small $t$, and this region has some interval $(-t,t)$ on the boundary. There are exactly two regions of this kind: one in the upper half-plane another symmetric in the lower half-plane. I will consider the one in the upper half-plane.

Now the question is reduced to this: is the region $D$ bounded? Here is a criterion: it is bounded if and only if it contains no critical point of $\phi$.

For example, if all critical points of $\phi$ are real, $D$ is bounded, and its boundary is a closed curve. More generally, if $\Im\phi(z)\leq 0$ at all critical points in the upper half-plane, $D$ is bounded and you have a closed curve.

In principle, one can make an algorithm which will check the exact condition on computer. 1. Find critical points of $\phi$. 2. For each critical point in the upper half-plane whose critical value has negative imaginary part, draw a ray to infinity in the upper half-plane which does not pass through other critical values. This ray has two preimages. If one preimage ends at $0$, there is no closed curve. If both end at $\infty$, and this happens for all such critical points, there is a bounded curve. Of course one has to be more careful if there is a critical point in the upper half-plane with real critical value, but this is a rare (non-generic) case.