3
$\begingroup$

It appears that the max area inscribed $n$-gon for an ellipse is not unique - if one inscribes a regular $n$-gon in a circle with radius a (there are infinitely many orientations for this inscribed regular $n$-gon) and then compresses the circle along one direction into an ellipse with minor axis $b$, the inscribed regular $n$-gon gets pressed into an $n$-gon that seems to be a max area inscribed $n$-gon for the $(a,b)$ ellipse.

  1. Given an integer $n$ and an ellipse with major and minor axes $a$ and $b$, how does one find and characterize inscribed $n$-gon(s) of maximum perimeter?

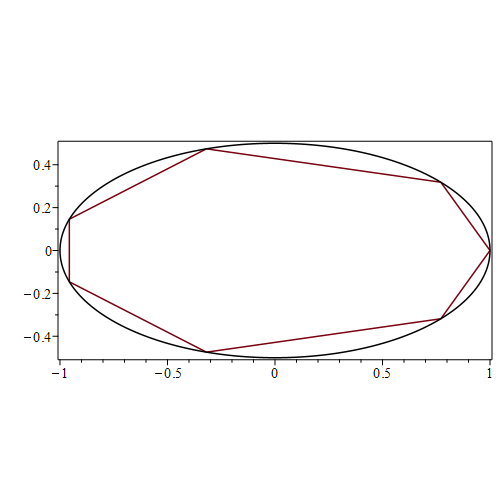
Guess: the max perimeter inscribed $n$-gons for an ellipse are probably polygonal closed billiard paths (such polygons for $n \ge 6$ are shown here). If this guess is right, does it generalize to cases where a closed convex curve allows such polygonal billiard paths with m edges? The guess also implies a unique answer for an ellipse and $n$; that brings up the issue: given any point $P$ on the ellipse boundary, to find the inscribed $n$-gon that necessarily contains $P$ and has maximum perimeter.

  1. When are the circumscribed $n$-gons of least perimeter and least area for an ellipse identical?

Note: one can also ask about inscribed and circumscribed $n$-gons in curves of constant width.

Improve this question
$\endgroup$
1
  • $\begingroup$ Ellipses and circles are the same, up to linear automorphisms. Your description captures thus the situation: In cercles you get maximal area for regular inscribed polygons and in ellipses you get maximal area for polygons which correspond to regular polygons of the associated circle. $\endgroup$
    – Roland Bacher
    Commented Oct 28, 2021 at 20:18

1 Answer 1

Reset to default
4
$\begingroup$

Consider allowing one vertex $B$ to vary on your curve, with neighbouring vertices $A$ and $C$ fixed. You want $B$ to be the point on the curve between $A$ and $C$ that maximizes total distance from $A$ and $C$. The curves of constant total distance from $A$ and $C$ are ellipses with foci at $A$ and $C$, so this total distance will be maximized at a point where your curve is tangent to one of those ellipses. As is well known, a light ray from one focus of an ellipse reflected off the ellipse goes to the other focus. If your curve is tangent to the ellipse at $B$, that means a light ray from $A$ will reflect off your curve at $B$ and go to $C$.

Standard methods of numerical optimization ought to work to find inscribed $n$-gons of maximum perimeter.

Here's one for $n=7$ with an ellipse of major and minor axes $2$ and $1$, produced using Maple.

enter image description here

Improve this answer
$\endgroup$
2
  • $\begingroup$ Thanks for the argument that appears to conclusively confirm the guess - the perimeter maximizing inscribed n-gon is a closed billiards trajectory. $\endgroup$
    – Nandakumar R
    Commented Oct 27, 2021 at 16:38
  • $\begingroup$ This question, mathoverflow.net/questions/78572/…, cites a result by Berger which I cannot access, but I read it is saying that all such ngon billiard paths have equal maximal perimeter. $\endgroup$
    – Yoav Kallus
    Commented Oct 31, 2021 at 17:55

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .