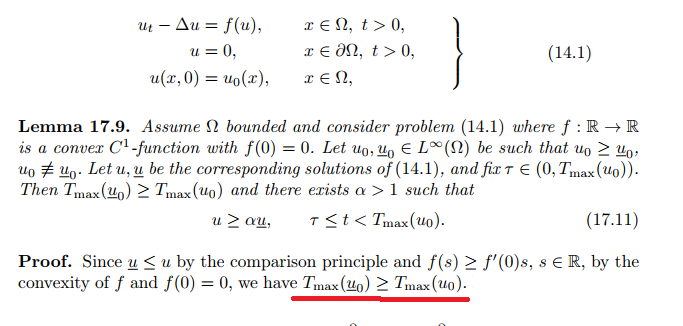
I'm reading the book superlinear parabolic problems and I came across the following situation twice: given two initial data $u_0$ and $\underline{u_0}$ with $u_0\geq \underline{u_0}$, $u_0\neq \underline{u_0}$, and considering $u$ and $\underline{u}$ the solutions of the problem below corresponding to these data, then maximal existence time of the $\underline{u}$ is larger than $u$, this is $T_{\max}(\underline{u_0})\geq T_{\max}(u_0)$. Is this always true? Is it an already formalized theorem? Where can I find him? Below I put the studied problem, and the Lemma in which I saw this fact
