$\newcommand\C{\mathcal C}\newcommand{\R}{\mathbb{R}}\newcommand{\tf}{\tilde f}
\newcommand{\tih}{\tilde h}$The answer is still no.
Indeed, suppose that we have a function $f\colon[0,\infty)\to\R$ such that $f(0)=0$ and $f$ is continuous, nonnegative, nondecreasing, and subadditive, but not superhomogeneous.
Then, for $\C:=[0,\infty)^n$, the function $h\colon\C\to\R$ given by the formula $h(x):=f(|x|)$ for all $x\in\C$ (where $|x|$ is the Eucludean norm of $x$) will be such that $h(0)=0$ and $h$ is continuous, nonnegative, nondecreasing in each of the coordinates of the argument, and subadditive, but not superhomogeneous on $\C$. In particular, the subadditivity of $h$ will follow from the subadditivity and monotonicity of $f$ and the triangle inequality $|x+y|\le|x|+|y|$ for $x$ and $y$ in $\C$.
So, it is enough to construct a function $f$ as above.
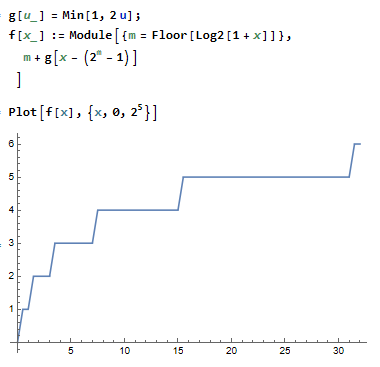
To do that, let
\begin{equation*}
f(x):=f_m(x):=m+g(x-(2^m-1))\text{ if }x\in J_m:=[2^m-1,2^{m+1}-1)
\end{equation*}
for some $m=0,1,\dots$, where
\begin{equation*}
g(u):=\min(1,2u).
\end{equation*}
Then $f(0)=0$ and $f$ is continuous, nonnegative, nondecreasing, and subadditive, but not superhomogeneous on $\C$.
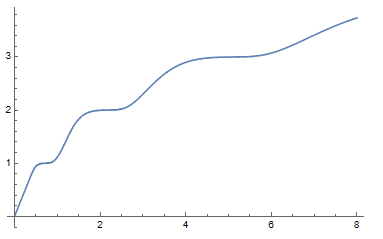
Here is the graph $\{(x,f(x))\colon0\le x\le2^5\}$:
Details: It is easy to see that $f(0)=0$ and $f$ is continuous, nonnegative, and nondecreasing. Also, if e.g. $x\in\mathcal C$,
$1<x<3/2$, and $t=1/x$, then $t\in(0,1)$, $f(x)=f_1(x)=1+2(x-1)$, $f(tx)=f_1(tx)=1$, and hence $f(tx)<tf(x)$, so that $f$ is not superhomogeneous on $\C$.
It remains to check that $f$ is subadditive. Take any $x$ and $y$ in $[0,\infty)$. Without loss of generality $x\le y$, so that $x\in J_k$ and $y\in J_m$ for some nonnegative integers $k$ and $m$ such that $k\le m$. So, $x+y<2^{k+1}-1+2^{m+1}-1<2^{m+2}-1$. Also, the function $g\colon[0,\infty)\to\R$ is nondecreasing and concave, with $g(0)=0$, so that $g$ is subadditive. So, if $k\ge1$, then
\begin{equation*}
\begin{aligned}
f(x+y)&\le f_{m+1}(x+y) \\
&=m+1+g(x+y-(2^{m+1}-1)) \\
&\le k+m+g(x+y-(2^{m+1}-1)) \\
&\le k+m+g(x-(2^k-1)+y-(2^m-1)) \\
&\le k+m+g(x-(2^k-1))+g(y-(2^m-1)) \\
&=f_k(x)+f_m(y)=f(x)+f(y).
\end{aligned}
\tag{0}
\end{equation*}
If $k=m=0$, then $x$ and $y$ are in $J_0=[0,1)$, and
$f(x)+f(y)=g(x)+g(y)$. So, if $x+y<1$, then $f(x+y)=f_0(x+y)\le g(x+y)$, and the inequality $f(x+y)\le f(x)+f(y)$ follows because $g$ is subadditive.
If now $x+y\ge1$, then $x+y\in J_1$ and hence $f(x+y)=f_1(x+y)=1+g(x+y-1)=g(1)+g(x+y-1)\le g(x)+g(y)$ by the concavity of $g$. So, $f(x+y)\le g(x)+g(y)=f(x)+f(y)$.
It remains to consider the case $k=0<m$, so that $x\in J_0=[0,1)$, $f(x)=g(x)$, and
\begin{equation*}
f(x)+f(y)=g(x)+m+g(y-(2^m-1)). \tag{1}
\end{equation*}
If now $x+y<2^{m+1}-1$, then
\begin{equation*}
f(x+y)\le f_m(x+y)=m+g(x+y-(2^m-1)) \\
\le m+g(x)+g(y-(2^m-1)),
\end{equation*}
since $g$ is subadditive. So, by (1), $f(x+y)\le f(x)+f(y)$.
It remains to consider the case when $k=0<m$ and $x+y\ge2^{m+1}-1$.
Then
$y\ge2^{m+1}-1-x\ge2^{m+1}-2\ge2^m$, since $m\ge1$.
Therefore and because $g$ is nondecreasing,
\begin{equation*}
f(x)+f(y)=g(x)+m+g(y-(2^m-1)) \\
\ge g(x)+m+g(1)=g(x)+m+1. \tag{2}
\end{equation*}
As in multiline display (0),
\begin{equation*}
f(x+y)\le m+1+g(x+y-(2^{m+1}-1)).
\end{equation*}
Therefore and because $y\in J_m$ and $g$ is nondecreasing, we have
\begin{equation*}
f(x+y)\le m+1+g(x+y-(2^{m+1}-1)) \\
\le g(x)+m+1\le f(x)+f(y),
\end{equation*}
by (2).
This completes the proof.
Moreover, we can construct a function $\tf\colon[0,\infty)\to\R$ such that $\tf(0)=0$, $\tf(x)=2x$ in a right neighborhood of $0$, $\tf$ is infinitely smooth on $(0,\infty)$, and still is nonnegative, nondecreasing, and subadditive, but not superhomogeneous. So, the function $\tih\colon\C\to\R$ given by the formula $\tih(x):=\tf(|x|)$ for all $x\in\C$ will be such that $\tih(0)=0$, $\tih(x)=2|x|$ in a neighborhood of $0$ in $\C$, $\tih$ is continuous on $\C$, infinitely smooth on the interior of $\C$,
nonnegative, nondecreasing in each of the coordinates of the argument, and subadditive, but not superhomogeneous on $\C$.
Indeed, let $K\colon(0,\infty)\to\R$ be any nonnegative infinitely smooth function supported on a small neighborhood, say $V$, of $1$ and such that $\int_0^\infty cK(c)\,dc=1$. Let then the function $\tf\colon[0,\infty)\to\R$ be defined as the multiplicative convolution with $K$ of the function $f$ constructed above:
\begin{equation}
\tf(x):=\int_0^\infty f(cx)K(c)\,dc
\end{equation}
for $x\in[0,\infty)$. So, the function $\tf$ is a mixture of the horizontal rescalings $[0,\infty)\ni x\mapsto f(cx)$ of $f$. So, $\tf(0)=0$ and $\tf$ is nonnegative, nondecreasing, and subadditive.
Moreover, if the neighborhood $V$ of $1$ is small enough, then $\tf$ will be close enough to $f$ and thus not superhomogeneous. Furthermore, if the neighborhood of $1$ is small enough, then for all $x$ in some right neighborhood of $0$ we will have
\begin{equation}
\tf(x)=\int_0^\infty 2cx K(c)\,dc=2x.
\end{equation}
Finally, for any $x\in(0,\infty)$,
\begin{equation}
\tf(x)=\frac1x\,\int_0^\infty f(u)K\Big(\frac ux\Big)\,du,
\end{equation}
which shows that $\tf$ is infinitely smooth on $(0,\infty)$.
Here is the graph $\{(x,\tf(x))\colon0\le x\le2^3\}$ for $$K(c):=\frac1C\,\exp\Big(-\frac1{1/4-(c-1)^2}\Big)\,1(|c-1|<1/2)$$
and $C:=\displaystyle{\int_{1/2}^{3/2} \exp\Big(-\dfrac1{1/4-(c-1)^2}\Big)\,dc}$: