-1
$\begingroup$

I have the equation

$$M = c_1 + c_2M - c_3T\ln\left(\left|\frac{e^{(c_4M + c_5)/T}-1}{e^{(c_6M + c_5)/T}-1}\right|\right)$$

where $c_1, \dots, c_6$ are constants.

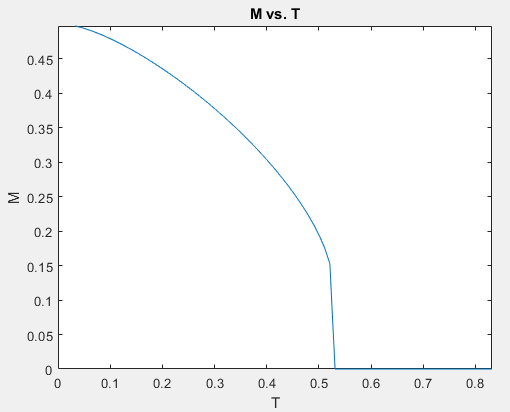
I am interested in the roots of $$M\in[0,0.5]$$ at each value of T. According to the following graph, the equation should have roots.

enter image description here

My question is, how to find analytically the cut off point $T_c$ (which appears as a sudden drop in the graph)after which the equation has no more roots in this interval?

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ please don't change the question after it has been answered, at least not without indicating the changes you made (to avoid your question becoming a moving target) $\endgroup$
    – Carlo Beenakker
    Commented Jul 1, 2021 at 19:18
  • $\begingroup$ Sorry for that but I thought it was not clear enough! $\endgroup$
    – Essa Ibrahim
    Commented Jul 1, 2021 at 21:51

1 Answer 1

Reset to default
1
$\begingroup$

There does not seem to be something as simple as a single cutoff point $T_c$. For any given real $T$, there is an odd number of real solutions $M_1,M_2,M_3,\dots M_{2p+1}$. There are critical $T$'s where the number of solutions changes by $\pm 2$, but there is alway at least 1 solution.

By way of illustration, here are two plots of $M_n$ versus $T$, for two different choices of the parameters $c_1,c_2,c_3,c_4,c_5,c_6$:

Mathematica command to explore this:

Manipulate[ContourPlot[m==c1+c2*m-c3*t*Log[Abs[(Exp[(c4*m+c5)/t]-1)/(Exp[(c6*m+c5)/t]-1)]],{t,-6,6},{m,-5,5},PlotPoints->75,MaxRecursion->3],{c1,-1,1},{c2,-1,1},{c3,-1,1},{c4,-1,1},{c5,-1,1},{c6,-1,1}]

The OP has edited the question and now restricts $M$ to the interval $(0,0.5)$, but that does not really change things, there are still multiple solutions even in that interval, see plot for an example.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .