3
$\begingroup$

I am looking for the proof of the following claim:

Consider a family of bicentric quadrilaterals with the same inradius length and the same distance between incenter and circumcenter. Denote by $P$ and $Q$ the midpoints of the diagonals, and by $I$ the incenter. Then, $|PI| \cdot |QI|$ has the same value for all quadrilaterals in the family.

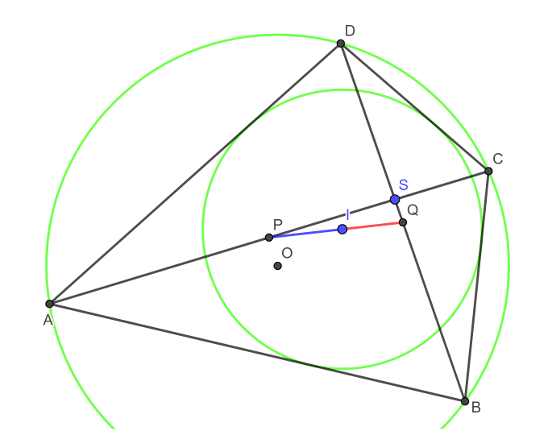
enter image description here

The GeoGebra applet that demonstrates this claim can be found here.

Improve this question
$\endgroup$
5
  • $\begingroup$ What is fixed except inradius? $\endgroup$
    – Fedor Petrov
    Commented Jun 20, 2021 at 5:41
  • $\begingroup$ A suggestion, using the $p,q$ method, since I haven't worked out the details. One can assume that the quadrilateral has vertices $(0,0),(a,0),(p,q),(r,s)$. The three conditions imposed (bicentricity, constant inradius) can be expressed as polynomial ones on $p,q,r,s,a$, as can the final identity. One then uses Gröbner bases. As usual, care must be taken when squaring up. $\endgroup$
    – bathalf15320
    Commented Jun 20, 2021 at 6:08
  • $\begingroup$ @FedorPetrov The distance between incenter and circumcenter. Also radius of circumscribed circle is the same for all quadrilaterals in the family because distance between incenter and circumcenter, inradius and radius of circumscribed circle are related by Fuss theorem. $\endgroup$
    – Pedja
    Commented Jun 20, 2021 at 6:27
  • $\begingroup$ All points $P$ and $Q$ lie on the same circle, so $-PI\cdot QI$ is just a power of a point $I$ in this circle. $\endgroup$
    – Fedor Bakharev
    Commented Jul 31, 2021 at 9:35
  • $\begingroup$ @FedorBakharev How do you know that all $P$ and $Q$ lie on some circle? $\endgroup$
    – Alex M.
    Commented Jul 31, 2021 at 15:13

1 Answer 1

Reset to default
5
$\begingroup$

Let the incircle be the unit circle. Let $A'$, $B'$, $C'$, $D'$ be the points where this is tangent to $AB$, $BC$, $CD$, $DA$, and let them have coordinates $(\cos \alpha, \sin \alpha)$, $(\cos \beta,\sin \beta)$, $(\cos \gamma, \sin \gamma)$ and $(\cos \delta, \sin \delta)$.

Then the quadrilateral $IA'BB'$ has two right angles, so $\angle A'BB' = \pi - \angle B'IA' = \pi - \beta + \alpha$. Likewise, the quadrilateral $IC'DD'$ has $\angle C'DD' = \pi - \delta + \beta$. But these two angles are opposite angles in a cyclic quadrilateral, so they sum to $\pi$. Thus $(\pi - \beta + \alpha) + (\pi - \delta + \beta) = \pi$, and $\delta = \pi + \alpha - \beta + \gamma$.

Now $$A = \left( \frac{\sin\alpha-\sin\delta}{\sin(\alpha-\delta)}, -\frac{\cos\alpha-\cos\delta}{\sin(\alpha-\delta)} \right)$$ $$B = \left( \frac{\sin\beta-\sin\alpha}{\sin(\beta-\alpha)}, -\frac{\cos\beta-\cos\alpha}{\sin(\beta-\alpha)} \right)$$ $$C = \left( \frac{\sin\gamma-\sin\beta}{\sin(\gamma-\beta)}, -\frac{\cos\gamma-\cos\beta}{\sin(\gamma-\beta)} \right)$$ $$D = \left( \frac{\sin\delta-\sin\gamma}{\sin(\delta-\gamma)}, -\frac{\cos\delta-\cos\gamma}{\sin(\delta-\gamma)} \right)$$ Letting $\theta=\alpha-\beta$ and $\phi=\beta-\gamma$, we have: $$|PI|^2=\frac14(A+C)\cdot(A+C)=\frac{1-\sin\theta\, \sin\phi}{(\sin\phi)^2}$$ $$|QI|^2=\frac14(B+D)\cdot(B+D)=\frac{1-\sin\theta\, \sin\phi}{(\sin\theta)^2}$$ And after finding the circumcenter $O$, $$|IO|^2=\frac{1-\sin\theta\, \sin\phi}{(\sin\theta)^2(\sin\phi)^2}$$ This leads to $$|IO|^2=|PI||QI|+|PI|^2|QI|^2$$ or in unit-free terms $$|IO|^2=|PI||QI|+|PI|^2|QI|^2/\text{inradius}^2$$ which establishes the claim.

Improve this answer
$\endgroup$

Not the answer you're looking for? Browse other questions tagged .