Let's take a Riemann surface $\Sigma$ and three Lagrangians $L_0,L_1,L_2$ in general position. let's assume that we can set up Lagrangian Floer (co)homology - Here I'm being vague because I don't want to assume too much and I'm interested in what can be said. By counting $J$-holomorphic triangles one can define a product and a coproduct map: $$ \mu_2 \colon CF(L_0,L_2)\otimes CF(L_2,L_1)\to CF(L_0,L_1)$$ $$ \mu^2 \colon CF(L_0,L_1)\to CF(L_0,L_2)\otimes CF(L_2,L_1)$$
It seems natural to consider $\mu_2\circ \mu^2 : CF(L_0,L_1)\to CF(L_0,L_1)$. What can be said about this map? is there any "explicit" description/property of it?
If we use the interpretation of maps between Floer cochains as the count of sections of a given (Lefschetz) fibration (a la Seidel say), it's clear that we are counting J-hol sections of a trivial fibration $\Sigma \times A$, where $A$ is the given surface with boundary (and strip-like ends) 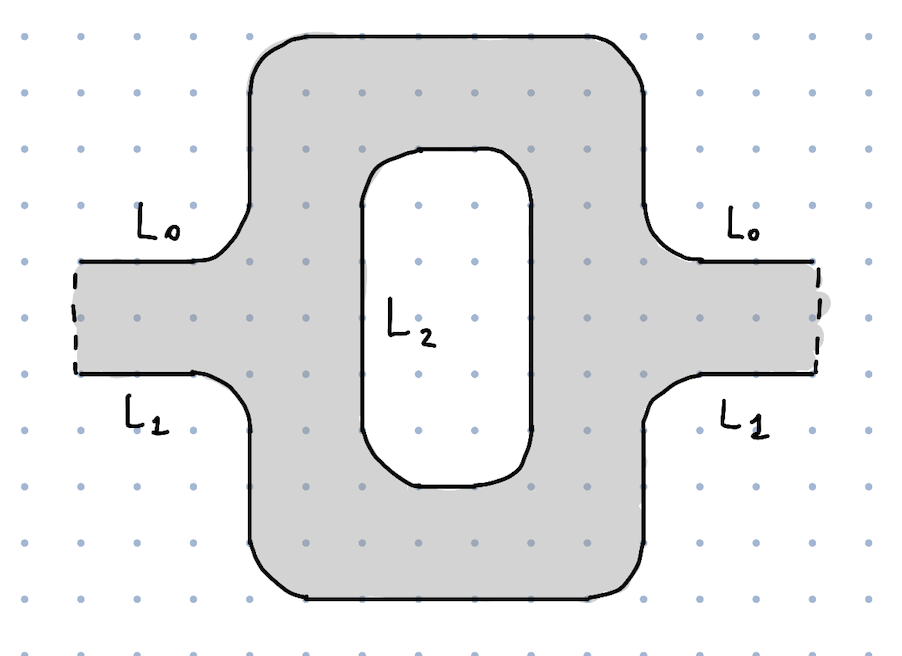
The labels on the boundary indicate that our $J$-hol section has the prescribed Lagrangian boundary condition.
is there any reference who dealt with a similar question/has some explicit computations regarding this composition?
