Non-isomorphic algebras becoming isomorphic after adding identities: mistake in an exercise

Question

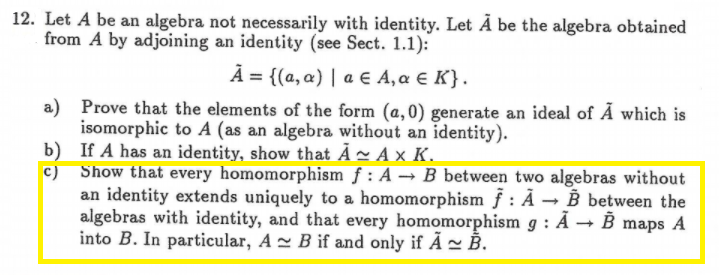
In the book: Drozd, Y.A., Kirichenko, V.V.: Finite dimensional algebras. Springer, Berlin (1994), there is an exercise which suggests a positive answer to the next question, and Ryszard R. Andruszkiewicz gives a counterexample:

Let $A,B$ be two arbitrary finite-dimensional algebras (spaces with associative laws) over a field $K$, and let $\overset{\sim}{A}:=\{(a,\alpha)\mid a\in A, \alpha\in K\}$, it is a well-known method of adjoining an identity to an arbitrary algebra $A$.

$$(a_1, α_1) · (a_2, α_2) = (a_1 · a_2 + α_2a_1 + α_1a_2, α_1α_2)$$ $$(a_1, α_1) + (a_2, α_2) = (a_1 + a_2, α_1 + α_2)$$ $$\forall\beta\in K:\beta· (a, α) = (βa, βα)$$

$\overset{\sim}{A}$ is an algebra with identity $(0, 1)$, in the same way we will define $\overset{\sim}{B}$.

The exercise: $A\cong B$ if and only if $\overset{\sim}{A}\cong\overset{\sim}{B}$.

Ryszard R. Andruszkiewicz's counterexample: Surprise at adjoining an identity to an algebra.

Andruszkiewicz's counterexample is, and if I understand correctly, $A$ is not isomorphic to $B$ because an isomorphism of algebras preserves both the left and right identities, but $A$ has neither a right identity nor an identity while $B$ has a right identity.

Which shows that there might be an error in the exercise.

Is it true that there is an error in the exercise?

Edit: For the discussion in the comments

Answer 1

The counterexample is correct. I had put the same counterexample on mathoverflow in a comment last year, unaware of the above paper, on a question asking about rings not isomorphic to their opposite. See my comment on Pete Clark's answer to Simplest examples of rings that are not isomorphic to their opposites. Conceptually it is this. If you take a 2 element left zero semigroup $S=\{x,y\}$ with multiplication $ab=a$ for all $a,b\in S$ then obviously $x,y$ are distinct right identities and if $T$ is the opposite semigroup consisting of two right zeroes then $T$ has two distinct left identies. Thus the semigroup algebras $KS$ and $KT$ are not isomorphic over any field. But when you adjoin identities to $KS$ and $KT$ (or equivalently adjoin an identity to the semigroups and take monoid algebras) you get algebras both isomorphic to $2\times 2$ upper triangular matrices. The trick is in $KS^1$ where $S^1$ is the monoid obtained by adjoining an identity to $S$ the elements $1,1-x,1-y$ is a basis but $1-x,1-y$ form a right zero semigroup isomorphic to $T$. So $KS^1\cong KT^1$.

Both the algebras live in $2\times2$ upper triangular matrices, which is the result of adding and identity. $KS$ consists of those upper triangular matrices whose second row is zero and $KT$ consists of those whose first column is zero.

I don't have the book. By any chance were they assuming $A$ and $B$ have identities in the exercise?