3
$\begingroup$

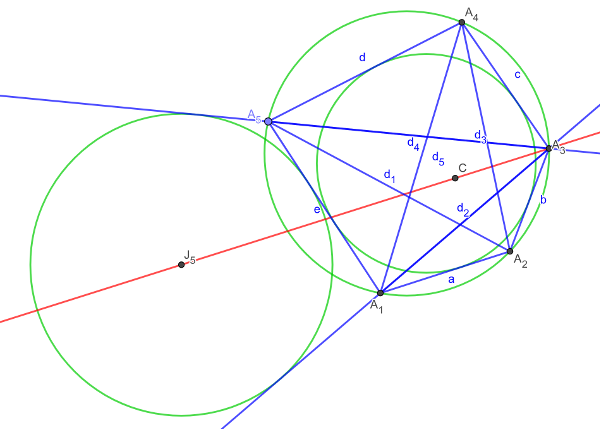
Can you provide a proof for the following claim?

Claim. Given bicentric pentagon. Consider the triangle whose sides are two diagonals drawn from the same vertex and side of pentagon opposite from that vertex.Then, center of excircle of this triangle which touches side of pentagon, the vertex of pentagon opposite to that side and the incenter of the pentagon formed by diagonals are collinear.

enter image description here

GeoGebra applet that demonstrates this claim can be found here.

Improve this question
$\endgroup$
2
  • $\begingroup$ This would be clearer if it included the letter labels in the text, e.g. "the same vertex ($A_3$) and the opposite side ($A_1A_5$)" $\endgroup$
    – user44143
    Commented Apr 22, 2021 at 12:31
  • $\begingroup$ @MattF. Thank you, but I think that there is no need for additional clarification. $\endgroup$
    – Pedja
    Commented Apr 22, 2021 at 12:48

1 Answer 1

Reset to default
3
$\begingroup$

Both $J_5$ and $C$ are equidistant from the lines on which $d_2$ and $d_4$ lie, hence they are on the bisector of $\angle A_5A_3A_1$ and thus are collinear with $A_3$.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .